751.40 LFD Widening and Repair
751.40.1 General
751.40.1.1 Widening and Repair of Existing Structures
The Federal Highway Administration and the States have established a goal that the LRFD standards be used on all new bridge designs after October 2007. For modification to existing structures and with the approval of the Structural Project Manager or Structural Liaison Engineer, the LRFD Specifications or the specifications which were used for the original design, may be used by the designer.
751.40.2 Typical Sections of Concrete Repairs
751.40.2.1 Resurfacing
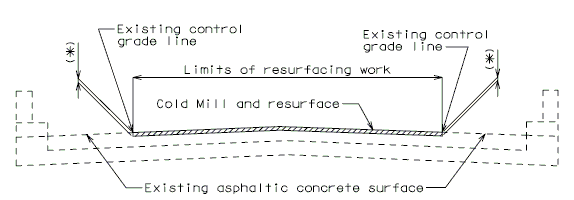
Place the following notes on plans.
- The existing Asphaltic Concrete surface shall be removed to a uniform grade line (*) below the existing control grade line as noted.
- Resurface with (*) Asphaltic Concrete.
- (*) Depth of Asphaltic Concrete as specified in the Design Layout.
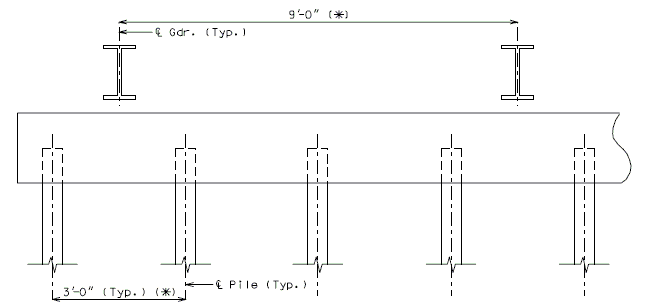
751.40.2.2 Special Repair Zones
The following order of repair zones are to be used for the deck repair on continuous concrete structures.
Zones with the same letter designation may be repaired at the same time. Sequence of repairs follows zone A, zone B then zone C.
Zone A is to be completed before Zone B and Zone B before Zone C, etc.
Any repair in the remainder of the bridge that is adjacent to Zone A shall be completed prior to work in Zone A.
If an excessive number of zones are required at one bent, See the Structural Project Manager.
Consider combining zones if it is ≤ 24".
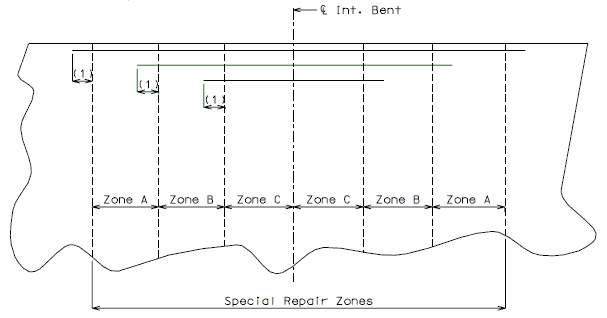
(1) Development Length.
See EPG 751.50 Standard Detailing Notes for appropriate notes.
751.40.2.3 Substructure Repair

751.40.2.4 Deck Repair
| Bridge Deck, Repair |
| Report 2002 |
| See also: Innovation Library |
DECKS WITHOUT AN EXISTING WEARING SURFACE (NEAR CENTERLINE SPAN)

TOTAL SURFACE HYDRO DEMOLITION DECK REPAIR

DECKS WITHOUT AN EXISTING WEARING SURFACE (NEAR CENTERLINE SPAN)



| (1) | Scarify existing slab. See the Design Layout for the minimum depth of scarification for the Concrete Wearing Surface. Scarification not required for Asphaltic Concrete Wearing Surface and Epoxy Polymer Concrete Overlay. |
| (2) | One inch vertical side shall be established outside the deteriorated area. See Sec 704. |
| (3) | 1/4" (min.) for Epoxy Polymer Concrete Overlay. 1-3/4" (min.) for Latex Modified Concrete. 2-1/4" (min.) for Low Slump Concrete or Silica Fume Concrete. |
DECKS WITH AN EXISTING WEARING SURFACE (NEAR CENTERLINE SPAN)



| (1) | Remove existing wearing surface plus 1/4" removal not required for Asphaltic Concrete Wearing Surface and Epoxy Polymer Concrete Overlay. |
| (2) | One inch vertical side shall be established outside the deteriorated area. See Sec 704. |
| (3) | 1/4" (min.) for Epoxy Polymer Concrete Overlay. 1-3/4" (min.) for Latex Modified Concrete. 2-1/4" (min.) for Low Slump Concrete or Silica Fume Concrete. |
DECKS WITHOUT AN EXISTING WEARING SURFACE (NEAR CENTERLINE SPAN)
HYDRO DEMOLITION



| (1) | Scarify existing slab. See the Design Layout for the minimum depth of scarification for the Concrete Wearing Surface. Scarification not required for Asphaltic Concrete Wearing Surface and Epoxy Polymer Concrete Overlay. |
| (2) | Total surface hydro demolition of existing slab. See the Design Layout for the minimum depth of total surface hydro demolition. |
| (3) | One inch vertical side shall be established outside the deteriorated area. See Sec 704. |
| (4) | 1-3/4" (min.) for Latex Modified Concrete. 2-1/4" (min.) for Low Slump Concrete or Silica Fume Concrete. |
| Partial Depth Repair is not allowed for Low Slump Concrete. Repairing Concrete Deck (Half-Soling) shall be substituted for Partial Depth Repair in the above details and not monolithically with the wearing surface. |
DECKS WITH AN EXISTING WEARING SURFACE (NEAR CENTERLINE SPAN)
HYDRO DEMOLITION



| (1) | Remove existing wearing surface plus 1/4" of existing deck. 1/4" removal not required for Asphaltic Concrete Wearing Surface and Epoxy Polymer Concrete Overlay. |
| (2) | Total surface hydro demolition of existing slab. See the Design Layout for the minimum depth of total surface hydro demolition. |
| (3) | One inch vertical side shall be established outside the deteriorated area. See Sec 704. |
| (4) | 1-3/4" (min.) for Latex Modified Concrete. 2-1/4" (min.) for Low Slump Concrete or Silica Fume Concrete. |
| Partial Depth Repair is not allowed for Low Slump Concrete. Repairing Concrete Deck (Half-Soling) shall be substituted for Partial Depth Repair in the above details and not monolithically with the wearing surface. |
751.40.2.5 Filled Joints
LATEX, LOW SLUMP OR SILICA FUME

ASPHALTIC CONCRETE OVERLAY


(*) Saw cut 1" deep and fill with concrete and asphalt joint sealer, hot-poured elastic type.
EPOXY POLYMER CONCRETE OVERLAY


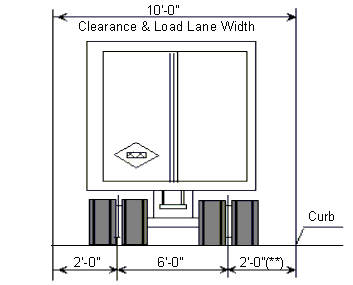
751.40.2.6 Temporary Traffic Control Device
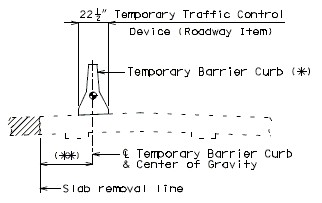
| (*) | Show Barrier Curb as per District recommendation. Typically Barrier Curb is shown when structure is on interstate and/or the rail is being removed. Otherwise, show the dimension lines with 2' 0" dimension. |
| (**) | If this dimension is less than 48", the temporary curb may have to be doweled in, check with Structural Project Manager. |
751.40.3 Dimensions
751.40.3.1 Concrete Wearing Surface
REPLACEMENT OF EXISTING EXPANSION DEVICE PREFORMED COMPRESSION JOINT SEAL (STRIP SEAL & SILICONE EXPANSION SEALANT DETAILS ARE SIMILAR)

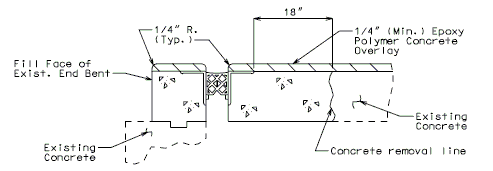
Note: The contractor shall exercise care to ensure that spillage over joint edges is prevented and that a neat line is obtained along any terminating edge of the epoxy polymer concrete.
Note:
Concrete overlay shall be forced into the cavity under the armor angle. Proper consolidation of the concrete shall be achieved by localized internal vibration.
When concrete is removed and expansion device armor is replaced, see Section 3.35 of this manual for the appropriate expansion device.
TYPICAL SECTION OF ELASTOMERIC EXPANSION DEVICE
When Low Slump, Silica Fume, Latex or Asphaltic Concrete wearing surface is used, the elastomeric joint must be replace by another type of expansion device.

TYPICAL SECTIONS OF FLAT PLATE EXPANSION DEVICE

| (*) | Latex, Low Slump or Silica Fume Concrete Wearing Surface. |
| (**) | When this dimension exceeds 3" and a Concrete Wearing Surface is used, tack weld a one inch bar chair to the plate for each 3" of plate to be covered by the Wearing Surface. |
| (***) | Scarify existing slab. See the Design Layout for the minimum depth of scarification. Scarification not required for Asphalt Concrete Wearing Surface. |
| Note: | See Standard Plans for Steel Dams at Expansion Devices for Resurfacing Bridge Floors. |

LATEX, LOW SLUMP, SILICA FUME, ASPHALTIC OR EPOXY POLYMER

OUTLET SHOWING LIMITS OF EPOXY SEAL
Note:
(*) Dimension to edge of girder or stringer ±. For bridges that do not have girders or stringers use 2'-6", except that if with thrie beam rail, then use 4'-0".
Consult with Structural Project Manager or Liaison for making work incidental to another item or use of pay item "Clean and Epoxy Seal".

OUTLET SHOWING LIMITS OF EPOXY SEAL
(Wearing surface not shown for clarity)
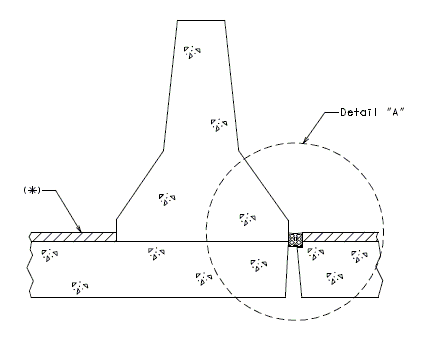
SLAB EDGE REPAIR
If slab edge repair is specified on the Design Layout when the barrier curb is not removed or when full depth repair is not a pay item, the following detail shall be provided.

If the barrier curb is removed when full depth repair and slab edge repair are pay items, the following detail shall be provided.

(*) If the dimension excceds 4", the repair extending to the edge of slab will be paid for as Full Depth Repair.
751.40.3.2 Longitudinal Joint Details
REPLACEMENT OF EXISTING EXPANSION DEVICE
MEDIAN BARRIER CURB
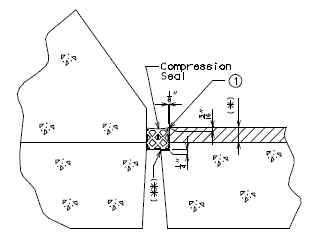
| (1) | May be cast vertical and saw cut to slant. |
| (*) | Latex Concrete Wearing Surface = 1-3/4". Low Slump Concrete Wearing Surface = 2-1/4". |
| (**) | Cut minimum 1/2" support notch (rought finish). Remove any existing compression seal. |
751.40.4 Curb Treatments
751.40.4.1 Replacement of Brush Curb with Safety Barrier Curb
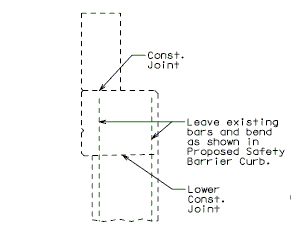
NON-INTEGRAL END BENTS
|
|
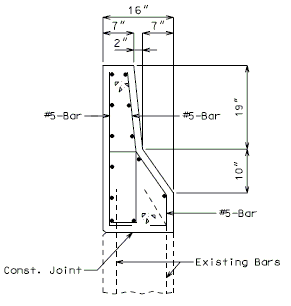
| EXISTING BRUSH CURB | PROPOSED SAFETY BARRIER CURB |
| SECTIONS THRU WING | |
Note: Remove existing Brush Curb above lower Const. Joint. For details not shown, see Section 3.30 of this manual.
|
|
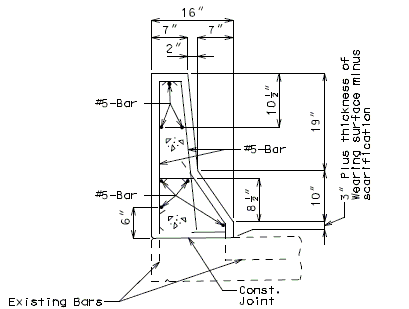
| EXISTING BRUSH CURB | PROPOSED SAFETY BARRIER CURB |
| SECTIONS THRU SLAB | |
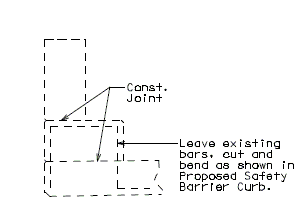
INTEGRAL END BENTS
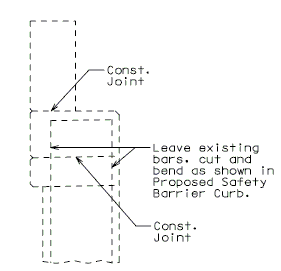
|
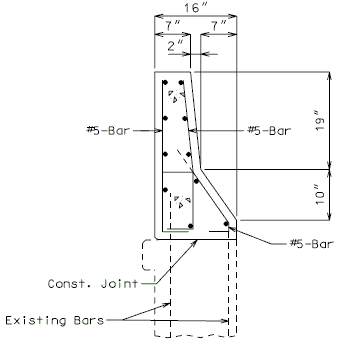
|
| EXISTING BRUSH CURB | PROPOSED SAFETY BARRIER CURB |
| SECTIONS THRU WING | |
Note: Remove existing Brush Curb above lower Const. Joint.
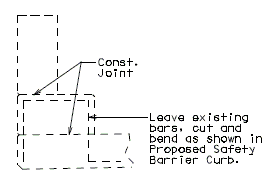
|
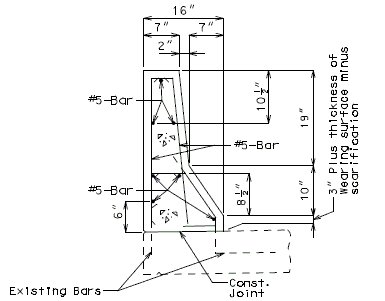
|
| EXISTING BRUSH CURB | PROPOSED SAFETY BARRIER CURB |
| SECTIONS THRU SLAB | |
751.40.4.2 Replacement of Existing Curb Using Anchor Systems
SAFETY BARRIER CURB ON SLAB


(OPTIONAL ANCHORING SYSTEM)
SAFETY BARRIER CURB ON WING


(OPTIONAL ANCHORING SYSTEM)
Note: See Section 4.0 for appropriate notes. For details not shown, see Section 3.30 of this manual.
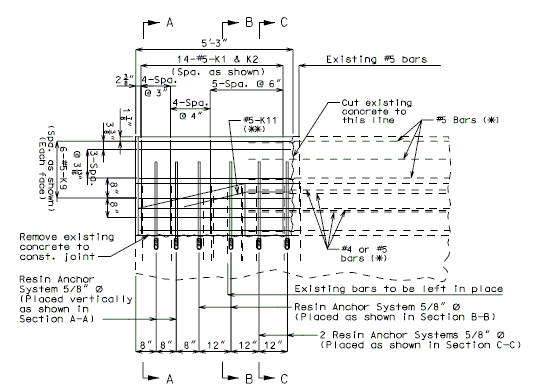
REPLACEMENT OF EXISTING CURB AT END OF WING USING ANCHOR SYSTEMS
INTEGRAL END BENTS
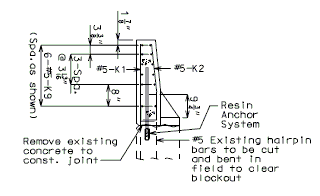
|
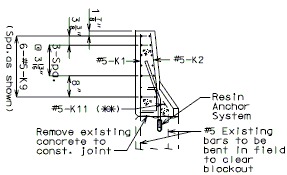
|
|
| SECTION A-A | SECTION B-B | SECTION C-C |
| (Horizontal bars are not shown for clarity) |
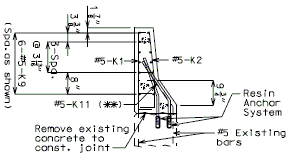
REPLACEMENT OF EXISTING CURB AT END OF WING USING ANCHOR SYSTEMS
NON-INTEGRAL END BENTS
|
| ||||||
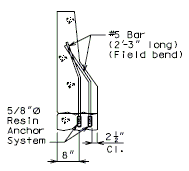
| |||||||
| ANCHOR SYSTEMS AT SECTION C-C | PART ELEVATION |
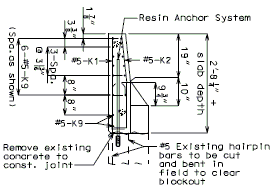
|
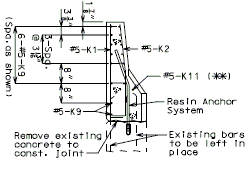
|
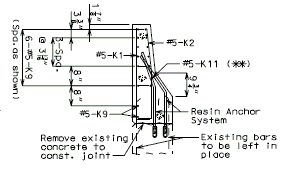
|
| SECTION A-A | SECTION B-B | SECTION C-C |
| (Horizontal bars are not shown for clarity) |
751.40.4.3 Replacement of Existing Curb with Thrie Beam Rail
SYSTEM 2
TYPICAL CONNECTION
System 2: Applicable for rehabs only with slab depths 22" or greater. These are typically voided slabs. Connection design load is 1.5 times plastic moment capacity (Mp) of W6 x 20 Post.

|
Blockout-to-Post Conn.
Centerline 2 Holes 13/16"Ø in W6 x 20 Post flange and W6 x 15 Blockout flange Centerline 2 Hex head bolt 5/8"Ø with two washers and hex nut in W6 x 20 Post flange Thrie Beam-to-Blockout Conn. Centerline 13/16" x 2-1/2" Vertical slotted hole in W6 x 15 Blockout flange (*) Centerline 5/8"Ø Carriage bolt with one flat washer and hex nut |
| (*) Required on one side of web only, but may be provided on both sides of web at the contrator's option.
(**) Use 10" from top of original slab (before any future wearing surface). For 20" voided slabs reduce to 8", see Structural Project Manager. | |
| (1) 2 Resin Anchor Systems that shall have a minimum ultimate pullout strength (each) of 72 kip in concrete with f'c = 4,000 psi to include:
2 Drilled holes 1-1/8"Ø (min.) in slab or as recommended by manufacturer (2) 2 Resin Anchor Systems that shall have a minimum ultimate pullout strength (each) of 20.4 kip in concrete with f'c = 4,000 psi to include:
2 Drilled holes 7/8"Ø (min.) in slab or as recommended by manufacturer |

|

| |
| SECTION A-A | DETAIL "B" |
DETAILS OF POST PLATE


DETAILS OF POST


|

|
| SECTION C-C | SECTION D-D |
SYSTEM 3
TYPICAL CONNECTION
System 3: Applicable for rehab and widenings with slab depths between 8-1/2" and 19" inclusive. Connection design load is 1.5 times plastic moment capacity (mp) of W6 x 20 Post. Vertical clearance must be checked due to obtruding lower connection.


|

|
| SECTION A-A | DETAIL "B" |
DETAILS OF BENT PLATE


DETAILS OF POST


|

|
| POST STIFFENER | POST PLATE WASHER |
SYSTEM 4
TYPICAL CONNECTION
System 4: Applicable for rehabs on Deck Girder, Box Girder and similar structures.


|
Notes:
(*) CANTILEVER MAY VARY. (1) Anchorage into cantilever portion of slab provided original slab thickness allows for min. embedment and 1" cover (check negative moment in cantilever). (2) Extending bent plate (within reason). (3) Alternating method of attachment, i.e. System 1 or System 3 types and variations thereof, Consult Structural Project Manager. |
| OPTIONAL TIE CONNECTION |
SYSTEM 4 AND OPTIONAL SYSTEM 4
DETAILS OF BENT PLATE
When a latex, a low slump or a silica fume concrete overlay is used, add the following detailis:


DETAILS OF POST


|

|
| POST STIFFENER | POST PLATE WASHER |
OPTIONAL SYSTEM 4
TYPICAL CONNECTION
Optional system 4: Applicable for rehabs on Deck Girder and Box Girder with large cantilevers.

| (1) | Tie-to-Deck Girder Conn. |
| Centerline 2 Resin Anchor Systems to include: | |
| Centerline 2 Drilled holes 7/8"Ø (min.) or as recommended by manufacturer in girder | |
| Centerline 2 Holes 1"Ø in 6" x 6" x 3/8" plate | |
| Centerline 2 Threaded rod 3/4"Ø A449 H.S., snug tight, 5" embedment in girder | |
| Centerline 2 Hardened locking washers | |
| (2) | Bent Plate-to-Deck Conn. |
| Centerline 3 Bolts 1"Ø A325 H.S. snug tight, with hardened washers | |
| Centerline 3 Drilled holes 1-1/8"Ø (min.) in old concrete or as recommended by manufacturer | |
| Centerline 3 Holes 1-1/4"Ø in bent plate and 9" x 16" x 3/8" plate | |
| (3) | Post-to-Bent Plate Conn. |
| Centerline 2 Bolts 1"Ø A325 H.S. with hardened washers | |
| Centerline 2 Vertical slotted holes 1-1/16" x 1-1/2" in post flanges | |
| Centerline 2 Holes 1-1/16"Ø in bent plate and post plate washer | |
| (4) | Post-to-Tie Conn. |
| Centerline 2 Bolts 3/4"Ø A325 H.S. with hardened washers | |
| Centerline 2 Holes 15/16"Ø in post flange and 6" x 6" x 3/8" plate |
751.40.5 Drainage
751.40.5.1 Slab Drain Details
FOR STRUCTURES WITH OVERLAYS
(GIRDER DEPTH LESS THAN 48")


| (*) | Deck thickness minus 1/8" minus the depth of the scarification. |
| (**) | Do not include the depth of the scarification. |

FOR STRUCTURES WITH OVERLAYS
(GIRDER DEPTH 48" AND OVER)
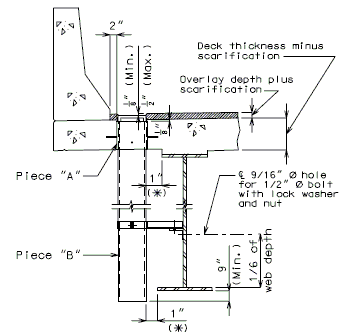
|

|
| ELEVATION OF DRAIN | |
| |
| PART ELEVATION OF SLAB AT DRAIN | PLAN OF DRAIN |
| (*) | If dimension is less than 1", drains shall be placed parallel to roadway. Otherwise, place drains transverse to roadway. |
| (**) | Do not include the depth of the scarification. |
| (***) | Deck thickness minus 1/8" minus the depth of the scarification. |
| |
| DRAIN TRANSVERSE TO ROADWAY | DRAIN PARALLEL TO ROADWAY |
| PART PLANS SHOWING BRACKET ASSEMBLY | |
FOR STRUCTURES WITH OVERLAYS
(CONTINUOUS CONCRETE STRUCTURES)
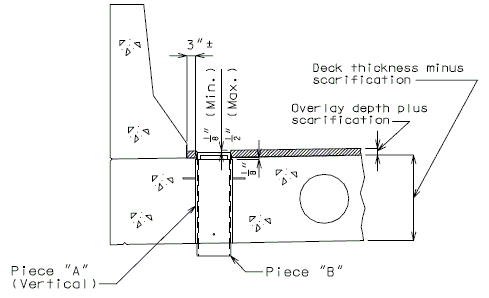
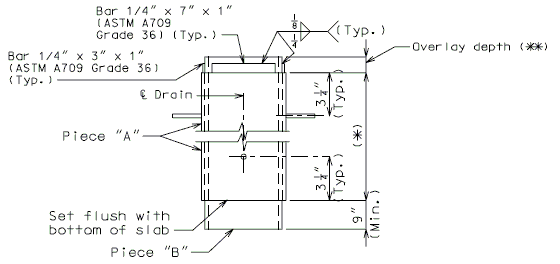
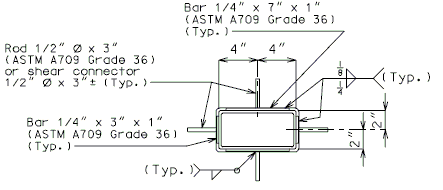
| (*) | Deck thickness minus 1/8" minus the depth of the scarification. |
| (**) | Do not include the depth of scarification. |
FOR STRUCTURES WITH OVERLAYS
(VARIABLE DEPTH GIRDERS)

Note: For variable depth girders with drains in deeper section, let the deeper section control and use throughout the structure.

FOR STRUCTURES WITH OVERLAY
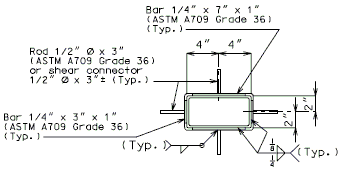
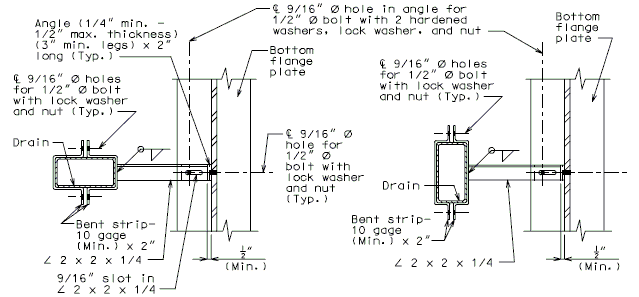
MISCELLANEOUS DETAILS - ROUND DRAINS
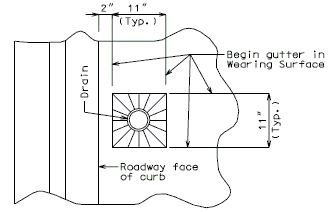
|
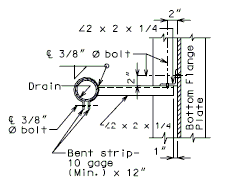
|
| TYPICAL PART PLAN | PART SECTION SHOWING BRACKET ASSEMBLY |

FOR STRUCTURES WITH OVERLAYS
RAISING STANDARD SLAB DRAINS


Note:
Outside dimensions of drain extension are 7-1/4" x 3-1/4", and drain extension shall be galvanized in accordance with ASTM A123.
FOR STRUCTURES WITH OVERLAYS
DETAILS FOR RAISING SCUPPERS


AND
PLAN OF SCUPPER EXTENSION
(*) Plate thicknesses should match those of existing scupper and existing grate.
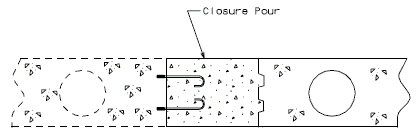
751.40.6 Closure Pour
Note:
For closure pour on solid slab or voided slab bridges, use expansive concrete.
Release the forms before the closure pour is placed.
751.40.7 Design and Posting Considerations
Existing structures to redecked and/or widened should be evaluated to determine if the superstructure is considered to be structurally adequate. The structural adequacy check should be determined based on load ratings using the Load Factor Method. Strengthening of the superstructure will not be required if the minimum posting values shown below meet or exceed legal load requirements. In addition, there may be cases where the existing bridge posting is acceptable based on the bridge specific site conditions such as ADT, amount of truck traffic, overweight permit route, etc.
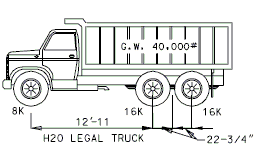
| 1) | H20 (one lane with Impact) [Posting Rating] ≥ 23 tons |
| 2) | 3S2 (one lane with impact) [Posting Rating] ≥ 40 tons |
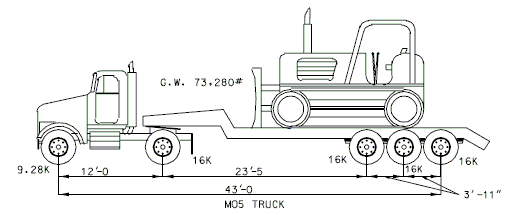
Posting Rating = 86% of Load Factor Operating Rating (Refer to figures below for H20, 3S2 and MO5 criteria).
If a structure is located within a commercial zone, then the following additional posting condition must be investigated:
| 3) | M05 (two lane with impact) [Operating Rating] ≥ 70 Tons (posting limit) |
Any other overstresses or inadequacies (slab, substructure, etc.) shall be reported to the Structural Project Manager.
Deck thickness for redecks shall be determined such that Posting will not be required or the existing posting is not lowered, and it is generally not less than original deck thickness.
Deck thickness for widenings shall be existing thickness unless thicker slab does not create overall deck stiffening irregularities.
See Structural Project Manager if AASHTO minimum deck thickness can not be used on redecks and widenings.
Future Wearing Surface (FWS) Loadings for widenings with concrete overlays - In addition to weight of overlay:
- Add FWS of 35 psf to the design of new girders if existing girders are sufficient for the 35 psf FWS
- Lower FWS loading to 15 psf if existing girders are not sufficient for FWS loading of 35 psf
- If existing girders are not sufficient for any FWS then lower FWS to FWS = 0.
- The existing ratings should be reviewed to determine what wearing surface loads were used. When necessary, the rating should be evaluated for acceptability of the proposed changes in the wearing surface loads and geometry. Preliminary ratings that are based on estimated geometry shall be revised when the updated, final geometry is known.

751.40.8 Design Information when using AASHTO Standard Specifications for Highway Bridges 17th Edition
751.40.8.1 Loadings
751.40.8.1.1 Live Load
Structures shall be designed to carry the dead load, live load, impact (or dynamic effect of the live load), wind load and other forces, when they are applicable.
Members shall be designed with reference to service loads and allowable stresses as provided in AASHTO (17th edition) Service Load Design Method (Allowable Stress Design) or with reference to factored load and factored strength as provided in AASHTO Strength Design Method (Load Factor Design). Load groups represent various combination of loads and forces to which a structure may be subjected. Group loading combinations for Service Load Design and Load Factor Design are given by AASHTO (17th edition) 3.22.1 and AASHTO (17th edition) Table 3.22.1A.
The live load shall consist of the applied moving load of vehicles and pedestrians. The design live load to be used in the design of bridges for the state system will be as stated on the Design Layout.
- The design truck: HS20-44 or HS20-44 Modified
- The design tandem (Military)
- The design lane loading
Criteria
- All widened or retrofitted bridges on the National Highway System and in commercial zones may be designed for HS20-44 Modified loading. All remaining bridges will be designed for HS20-44 loading.
- The Design Tandem loading is to be checked on national highway system or when Alternate Military loading appears on the Design Layout.
- Carrying members of each structure shall be investigated for the appropriate loading.
- Main carrying members include:
- Steel or Concrete stringers or girders.
- Longitudinally reinforced concrete slabs supported on transverse floor beams or substructure units (includes hollow slabs).
- Transversely reinforced concrete slabs supported by main carrying members parallel to traffic and over 8'-0" center to center. Use the formulas for moment in AASHTO Article 3.24.3.1 Case A.
- Steel grid floors when the main elements of the grid extend in a direction parallel to traffic, or with main elements transverse to traffic on supports more than 8'-0" apart.
- Timber floors and orthotropic steel decks.
- Main carrying members include:
- The reduction in live load for calculating substructure members is based on AASHTO 3.12.1. See Live Load Distribution in the Load Distribution Section.
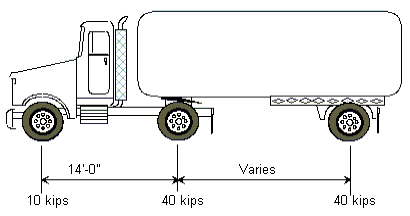
HS20-44 Truck Loading
The HS20-44 truck is defined below as one 8 kip axle load and two 32 kip axle loads spaced as shown.
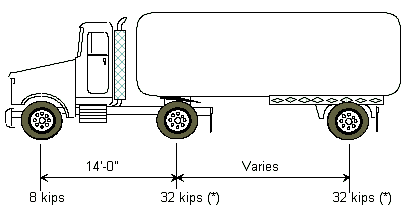
Varies = Variable spacing 14’ to 30’ inclusive. Spacing to be used is that which produces the maximum stresses.
(*) In the design of timber floors and orthotropic steel decks (excluding transverse beams) for H-20 Loading, one axle load of 24 kips or two axle loads of 16 kip each, spaced 4 feet apart may be used, whichever produces the greater stress, instead of the 32 kip axle load shown.
(**) For slab design, the center line of wheels shall be assumed to be one foot from face of cur
HS20-44 Modified Truck Loading
The HS20-44 Modified truck is defined below as one 10 kip axle load and two 40 kip axle loads spaced as shown. This is the same as HS20-44 truck modified by a factor of 1.25.
Varies = Variable spacing 14’ to 30’ inclusive. Spacing to be used is that which produces the maximum stresses.
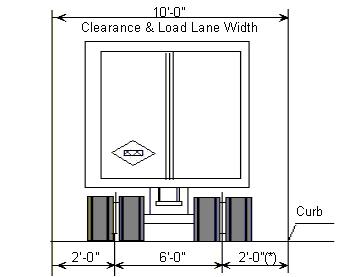
(*) For slab design, the center line of wheels shall be assumed to be one foot from face of curb.
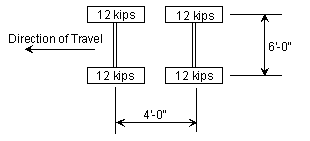
Design Tandem Loading
The Design Tandem Loading is a two axle load each of 24 kips. These axles are spaced at 4'-0" centers. The transverse spacing of wheels shall be taken as 6'-0".
Design Lane Loading
- For HS20-44 Truck, the design lane load shall consist of a load 640 lbs per linear foot, uniformly distributed in the longitudinal direction with a single concentrated load (or two concentrated loads in case of continuous spans for determination of maximum negative moment), so placed on the span as to produce maximum stress. The concentrated load and uniform load shall be considered as uniformly distributed over a 10'-0" width on a line normal to the center line of the lane.
- For HS20-44 Modified Truck, use the HS20-44 truck modified by a factor of 1.25.
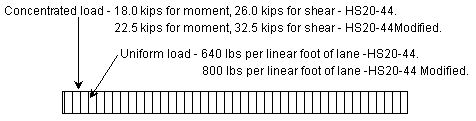
- For the design of continuous structures, an additional concentrated load is placed in another span to create the maximum effect. For positive moments, only one concentrated load is used, combined with as many spans loaded uniformly as are required to produce the maximum moment.
Standard Roadway Width
- 26'-0" (up to 2 traffic lanes)
- 28'-0" (up to 2 traffic lanes)
- 30'-0" (up to 3 traffic lanes)
- 32'-0" (up to 3 traffic lanes)
- 36'-0" (up to 3 traffic lanes)
- 38'-0" (up to 3 traffic lanes)
- 40'-0" (up to 4 traffic lanes)
- 44'-0" (up to 4 traffic lanes)
751.40.8.1.2 Impact
Highway live loads shall be increased by a factor given by the following formula:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, I = \frac {50}{L + 125}} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \,L } in feet
For continuous spans, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} to be used in this equation for negative moments is the average of two adjacent spans at an intermediate bent or the length of the end span at an end bent. For positive moments, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} is the span length from center to center of support for the span under consideration.
Impact is never to be more than 30 percent. It is intended that impact be included as part of the loads transferred from superstructure to substructure but not in loads transferred to footings or parts of substructure that are below the ground line. The design of neoprene bearing pads also does not include impact in the design loads.
751.40.8.1.3 Collision Force
Collision forces shall be applied to the barrier curb in the design of the cantilever slab. A force of 10 kips is to be applied at the top of the standard barrier curb. This force is distributed through the barrier curb to the slab.
751.40.8.1.4 Centrifugal Force
Structures on curves shall be designed for a horizontal radial force equal to the following percentage of the live load in all the lanes, without impact.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, C = \frac {6.68 S^2}{R}}
Where:
| = the centrifugal force in percent of the live load | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, S} | = the design speed in miles per hour |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, R} | = the radius of the curve in feet |
This force shall be applied at 6 feet above the centerline of the roadway with one design truck being placed in each lane in a position to create the maximum effect. Lane loads shall not be used in calculating centrifugal forces.
The effects of superelevation shall be taken into account.
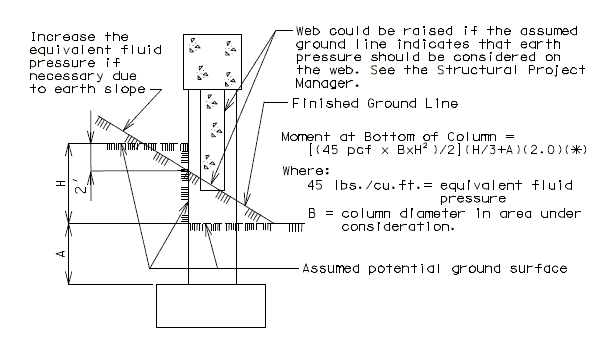
751.40.8.1.5 Lateral Earth Pressure
Structures which retain fills shall be designed for active earth pressures as
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P_a = 0.5 (\gamma K_a) H^2}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P_a} | = active earth pressure per length (lb/ft) | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \gamma} | = unit weight of the back fill soil = 120 lb/ft³ | |
| = coefficient of active earth pressure as given by Rankine’s formula | ||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \gamma K_a} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, p_a} | = equivalent fluid pressure (lb/ft³)(*) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, H} | = height of the back fill soil (ft) | |
Rankine's Formula
The coefficient of active earth pressure Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a} is:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a = (cos \alpha) \Bigg( \frac {cos \alpha - \sqrt{cos^2 \alpha - cos^2 \phi}}{cos \alpha + \sqrt{cos^2 \alpha - cos^2 \phi}} \Bigg)}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \phi} | = angle of internal friction of the backfill soil (*) |
| = the angle of incline of the backfill |
If the backfill surface is level, angle a is zero and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a} is:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a = \frac {1 - sin \phi}{1 + sin \phi}}
(*) Use the internal friction angle indicated on the Design Layout. However, if the friction angle is not determined, use the minimum equivalent fluid pressure value, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, p_a} , of 45 lb/ft³ for bridges and retaining walls. For box culverts use a maximum of 60 lb/ft³ and a minimum of 30 lb/ft³ for fluid pressure.
Live Load Surcharge
An additional earth pressure shall be applied to all structures which have live loads within a distance of half the structure height. This additional force shall be equal to adding 2'-0" of fill to that presently being retained by the structure.
751.40.8.1.6 Longitudinal Forces (Braking Forces)
A longitudinal force of 5% of the live load shall be applied to the structure. This load shall be 5% of the lane load plus the concentrated load for moment applied to all lanes and adjusted by the lane reduction factor. Apply this force at 6 feet above the top of slab and to be transmitted to the substructure through the superstructure.
751.40.8.1.7 Wind Load
Wind loads shall be applied to the structure regardless of length.
The pressure generated by wind load is:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = K V^2}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} | = wind pressure in pounds per square foot |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V} | = design wind velocity = 100 miles per hour |
| = 0.004 for wind load |
Basic wind load (pressure) = 0.004 x (100)² = 40 lb/ft²
Wind Load for Superstructure Design
Transverse
A wind load of the following intensity shall be applied horizontally at right angles to the longitudinal axis of the structure.
- Trusses and Arches = 75 pounds per square foot = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_t}
- Girders and Beams = 50 pounds per square foot (*) = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_t} (for plate girder lateral bracing check only)
- The total force shall not be less than 300 pounds per linear foot in the plane of windward chord and 150 pounds per linear foot in the plane of the leeward chord on truss spans, and not less than 300 pounds per linear foot on girder spans.
Wind Load for Substructure Design
Forces transmitted to the substructure by the superstructure and forces applied directly to the substructure by wind load shall be as follows:
Forces from Superstructure: Wind on Superstructure
Transverse
A wind load of the following intensity shall be applied horizontally at right angles to the longitudinal axis of the structure.
- Trusses and Arches = 75 pounds per square foot = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_t}
- Girders and Beams = 50 pounds per square foot (*) = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_t}
(*) Use Wt = 60 lbs/ft² for design wind force on girders and beams If the column height on a structure is greater than 50 feet, where the height is the average column length from ground line to bottom of beam.
The transverse wind force for a bent will be:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = L \times H \times W_t}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} | = length in feet = the average of two adjacent spans for intermediate bents and half of the length of the end span for end bents. |
| = the total height of the girders, slab, barrier curb and any superelevation of the roadway, in feet | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_t} | = wind force per unit area in pounds per square foot |
This transverse wind force will be applied at the top of the beam cap for the design of the substructure.
Longitudinal (**)
The standard wind force in the longitudinal direction shall be applied as a percentage of the transverse loading. Use approximately 25%.
Truss and Arch Structures Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_I} = 75 x 0.25 = approximately 20 lbs/ft² Girder Structures Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_I} = 50 x 0.25 = approximately 12 lbs/ft²
The total longitudinal wind force Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} will be:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = L \times H \times W_I}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} | = the overall bridge length in feet |
| = the total height of the girders, slab, barrier curb and anysuperelevation of the roadway, in feet | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W_I} | = wind force per unit area in pounds per square foot |
This longitudinal force is distributed to the bents based on their stiffness. (**)
The longitudinal wind force for the bent will be applied at the top of the beam cap for the design of the substructure.
Forces from Superstructure: Wind on Live Load
A force of 100 pounds per linear foot of the structure shall be applied transversely to the structure along with a force of 40 pounds per linear foot longitudinally. These forces are assumed to act 6 feet above the top of slab. The transverse force is applied at the bents based on the length of the adjacent spans affecting them. The longitudinal force is distributed to the bents based on their stiffness. (**)
(**) See Longitudinal Distribution of Wind Loads in Distribution of Loads Section.
Forces Applied Directly to the Substructure
The transverse and longitudinal forces to be applied directly to the substructure elements shall be calculated from an assumed basic wind force of 40 lbs/ft². This wind force per unit area shall be multiplied by the exposed area of each substructure member in elevation (use front view for longitudinal force and side view for transversely force, respectively). These forces are acting at the center of gravity of the exposed portion of the member.
A shape factor of 0.7 shall be used in applying wind forces to round substructure members.
When unusual conditions of terrain or the special nature of a structure indicates, a procedure other than the Standard Specification may be used subject to approval of the Structural Project Manager.
751.40.8.1.8 Temperature Forces
Temperature stresses or movement need to be checked on all structures regardless of length. Generation of longitudinal temperature forces is based on stiffness of the substructure. (*)
Coefficients
Steel: Thermal - 0.0000065 ft/ft/°F Concrete: Thermal - 0.0000060 ft/ft/°F Shrinkage - 0.0002 ft/ft (***) Friction - 0.65 for concrete on concrete
| Rise | Fall | Range | |
| Steel Structures | 60°F | 80°F | 140°F |
| Concrete Structures | 30°F | 40°F | 70°F |
(*) See Longitudinal Distribution of Temperature Forces in Distribution of Loads Section.
(**) Temperature Range for expansion bearing design and expansion devices design see Bearing Section, Expansion Devices Section, respectively.
(***) When calculating substructure forces of concrete slab bridges, the forces caused by the shrinkage of the superstructure should be included with forces due to temperature drop. This force can be ignored for most other types of bridges.
751.40.8.1.9 Sidewalk Loading
Sidewalk floors and their immediate support members shall be designed for a live load of 85 pounds per square foot of sidewalk area. Girders, trusses, and other members shall be design for the following sidewalk live load:
Spans 0 to 25 feet 85 lbs/ft² Spans 26 to 100 feet 60 lbs/ft² Spans over 100 feet use the following formula
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = \Bigg( 30 + \frac {3000}{L} \Bigg) \Bigg( \frac {55-W}{50} \Bigg)}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} | = live load per square foot, max. 60 lbs/ft² |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} | = loaded length of sidewalk in feet |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W} | = width of sidewalk in feet |
When sidewalk live loads are applied along with live load and impact, if the structure is to be designed by service loads, the allowable stress in the outside beam or stringer may be increased by 25 percent as long as the member is at least as strong as if it were not designed for the additional sidewalk load using the initial allowable stress. When the combination of sidewalk live load and traffic live load plus impact governs the design under the load factor method, use a b factor of 1.25 instead of 1.67.
Unless a more exact analysis can be performed, distribution of sidewalk live loads to the supporting stringers shall be considered as applied 75 percent to the exterior stringer and 25 percent to the next stringer.
751.40.8.1.10 Other Loads
Stream Pressure
Stream flow pressure shall be considered only in extreme cases. The affect of flowing water on piers shall not be considered except in cases of extreme high water and when the load applied to substructure elements is greater than that which is applied by wind on substructure forces at low water elevations.
The pressure generated by stream flow is:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = KV^2}
Where:
| = stream pressure in pounds per square foot | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V} | = design velocity of water in feet per second |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K} | = shape constant for the surface the water is in contact with. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K} | = 1.4 for square-ended piers |
| = 0.7 for circular piers | |
| = 0.5 for angle-ended piers where the angle is 30 degrees or less |
Ice Forces
Ice forces on piers shall be applied if they are indicated on the Design Layout.
Buoyancy
Buoyancy shall be considered when its effects are appreciable.
Fatigue in Structural Steel
Steel structures subjected to continuous reversal of loads are to be designed for fatigue loading.
Prestressing
See 751.22 P/S Concrete I Girders section.
Other Loads
Other loads may need to be applied if they are indicated on the Design Layout. Otherwise see Structural Project Manager before applying any additional loads.
751.40.8.1.11 Group Loads
Group Loading (Service Load Design)
Group loading combinations are:
GP I SL 100% GP II SL Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = D+W} 125% GP III SL Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = D+L+I+0.3W+WL+LF} 125% GP IV SL 125% GP V SL Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = D+W+T} 140% GP VI SL Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = D+L+I+0.3W+WL+LF+T} 140%
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, D} | = dead load |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} | = live load |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, I} | = live load impact |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W} | = wind load on structure |
| = wind load on live load | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, T} | = temperature force |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, LF} | = longitudinal force from live load |
Group Loading (Load Factor Design)
Group loading combinations are:
GP I LF Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = 1.3[\beta_d D+1.67 (L+I)]} GP II LF Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = 1.3[\beta_d D+W]} GP III LF Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = 1.3[\beta_d D+L+I+0.3W+WL+LF]} GP IV LF GP V LF Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = 1.25[\beta_d D+W+T]} GP VI LF Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, = 1.25[\beta_d D+L+I +0.3W+WL+LF+T]}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, D} | = dead load |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} | = live load |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, I} | = live load impact |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W} | = wind load on structure |
| = wind load on live load | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, T} | = temperature force |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, LF} | = longitudinal force from live load |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \beta_d} | = coefficient, see AASHTO Table 3.22.1A |
Other group loadings in AASHTO Table 3.22.1A shall be used when they apply.
751.40.8.2 Distribution of Loads
751.40.8.2.1 Distribution of Dead Load
Composite Steel or Prestressed Concrete Structures
The dead load applied to the girders through the slab shall be:
Dead Load 1
Non-composite dead loads should be distributed to girders (stringers) on the basis of continuous spans over simple supports.
Dead Load 2
Composite loads shall be distributed equally to all girders. The following are all Dead Load 2 loads:
- Barrier curb
- Future wearing surface on slab
- Sidewalks
- Fences
- Protective coatings and waterproofing on slab
Concrete Slab Bridges
Distribute entire dead load across full width of slab.
For longitudinal design, heavier portions of the slab may be considered as concentrated load for entry into the "Continuous Structure Analysis" computer program.
For transverse bent design, consider the dead load reaction at the bent to be a uniform load across entire length of the transverse beam.
751.40.8.2.2 Distribution of Live Load
Live loading to be distributed shall be the appropriate loading shown on the Design Layout. Applying Live Load to Structure
Superstructure
For application of live load to superstructure, the lane width is considered 12 feet. Each design vehicle has wheel lines which are 6 feet apart and adjacent design vehicles must be separated by 4 feet.
Substructure
To produce the maximum stresses in the main carrying members of substructure elements, multiple lanes are to be loaded simultaneously. The lane width is 12 feet. Partial lanes are not to be considered. Due to the improbability of coincident maximum loading, a reduction factor is applied to the number of lanes. This reduction however, is not applied in determining the distribution of loads to the stringers.
| Number of Lanes | Percent |
|---|---|
| one or two lanes | 100 |
| three lanes | 90 |
| four lanes or more | 75 |
Moment Distribution
Moments due to live loads shall not be distributed longitudinally. Lateral distribution shall be determined from AASHTO Table 3.23.1 for interior stringers. Outside stringers distribute live load assuming the flooring to act as a simple span, except in the case of a span with a concrete floor supported by four or more stringers, then AASHTO 3.23.2.3.1.5 shall be applied. In no case shall an exterior stringer have less carrying capacity than an interior stringer.
Shear Distribution
As with live load moment, the reactions to the live load are not to be distributed longitudinally. Lateral distribution of live load shall be that produced by assuming the flooring to act as simply supported. Wheel lines shall be spaced on accordance with AASHTO 3.7.6 and shall be placed in a fashion which provides the most contribution to the girder under investigation, regardless of lane configuration. The shear distribution factor at bents shall be used to design bearings and bearing stiffeners.
Deflection Distribution
Deflection due to live loads shall not be distributed longitudinally. Lateral distribution shall be determined by averaging the moment distribution factor and the number of wheel lines divided by the number of girder lines for all girders. The number of wheel lines shall be based on 12 foot lanes. The reduction in load intensity (AASHTO Article 3.12.1) shall not be applied.
Deflection Distribution Factor = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \cfrac {\big\{ \frac{2n}{N} \big\} + MDF}{2}}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} | = number of whole 12 foot lanes on the roadway |
| = number of girder lines; | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, MDF} | = Moment Distribution Factor. |
Example: 38'-0" Roadway (Interior Girder), Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n=3}
, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, N=5}
, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, MDF=1.576}
Deflection Distribution Factor = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \cfrac {\big\{ \frac{2 \times 3lanes}{5 girders} \big\} + 1.576}{2} = 1.388}
| Roadway Width |
Number Girders |
Girder Spacing |
Exterior Girder | Interior Girder | (1) | ||||
|---|---|---|---|---|---|---|---|---|---|
| Mom. | Shear | Defl. | Mom. | Shear | Defl. | ||||
| 26’-0” | 4 | 7’-6” | 1.277 | 1.133 | 1.139 | 1.364 | 1.667 | 1.182 | 1.071 |
| 28’-0” | 4 | 8’-2” | 1.352 | 1.204 | 1.176 | 1.485 | 1.776 | 1.243 | 1.167 |
| 30’-0” | 4 | 8’-8” | 1.405 | 1.308 | 1.453 | 1.576 | 1.846 | 1.538 | 1.238 |
| 32’-0” | 4 | 9’-2” | 1.457 | 1.400 | 1.479 | 1.667 | 1.909 | 1.584 | 1.310 |
| 36’-0” | 5 | 8’-2” | 1.352 | 1.184 | 1.276 | 1.485 | 1.776 | 1.343 | 1.167 |
| 38’-0” | 5 | 8’-8” | 1.405 | 1.231 | 1.303 | 1.576 | 1.846 | 1.388 | 1.238 |
| 40’-0” | 5 | 9’-0” | 1.440 | 1.333 | 1.520 | 1.636 | 1.889 | 1.618 | 1.286 |
| 44’-0” | 5 | 9’-9” | 1.515 | 1.487 | 1.558 | 1.773 | 1.974 | 1.687 | 1.393 |
Distribution of Live Load to Substructure
For substructure design the live load wheel lines shall be positioned on the slab to produce maximum moments and shears in the substructure. The wheel lines shall be distributed to the stringers on the basis of simple spans between stringers. The number of wheel lines used for substructure design shall be based on 12 foot lanes and shall not exceed the number of lanes times two with the appropriate percentage reduction for multiple lanes where applicable.
In computing these stresses generated by the lane loading, each 12 foot lane shall be considered a unit. Fractional units shall not be considered.
Distribution of Loads to Slabs
For simple spans, the span length shall be the distance center to center of supports but need not be greater than the clear distance plus the thickness of the slab. Slabs for girder and floor beam structures should be designed as supported on four sides.
For continuous spans on steel stringers or on thin flanged prestressed beams (top flange width to thickness ratios > 4.0), the span length shall be the distance between edges of top flanges plus one quarter of each top flange width. When the top flange width to thickness is < 4.0 the span distance shall be the clear span between edges of the top flanges
When designing the slab for live load, the wheel line shall be placed 1 foot from the face of the barrier curb if it produces a greater moment.
Bending Moments in Slab on Girders
The load distributed to the stringers shall be:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Bigg( \frac {S + 2}{32} \Bigg) } P20 or P25 = Moment in foot-pounds per-foot width of slab.
Where:
| = effective span length between girders in feet | |
| P20 or P25 | = wheel line load for HS20 or HS20 Modified design Truck in kips. |
For slabs continuous over 3 or more supports, a continuity factor of 0.8 shall be applied.
Main Reinforcement Parallel to Traffic
This distribution may be applied to special structure types when its use is indicated.
Distribution of Live Load to Concrete Slab Bridges
Live load for transverse beam, column and pile cap design shall be applied as concentrated loads of one wheel line. The number of wheel lines used shall not exceed the number of lanes x 2 with the appropriate reduction where applicable.
For slab longitudinal reinforcement design, use live load moment distribution factor of 1/E for a one-foot strip slab with the appropriate percentage reduction.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E = 4' + 0.06S, E (max.) = 7'}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E} | = Width of slab in feet over which a wheel is distributed |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, S} | = Effective span length in feet. |
For slab deflection, use the following deflection factor for a one-foot strip slab without applying percentage reduction.
Deflection Factor = (Total number of wheel line) / (width of the slab)
751.40.8.2.3 Frictional Resistance
The frictional resistance varies with different surfaces making contact. In the design of bearings, this resistance will alter how the longitudinal forces are distributed. The following table lists commonly encountered materials and their coefficients. These coefficients may be used to calculate the frictional resistance at each bent.
| Frictional Resistance of Expansion Bearings | |||
|---|---|---|---|
| Bearing Type | Coef. | General Data | |
| Type C Bearing | 0.14 | Coef. of sliding friction steel to steel = 0.14
Coef. for pin and rocker Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac {0.14 (Radius\ of\ pin)}{Radius\ of\ Rocker}}
Frictional Force = | |
| 6” Diameter Roller | 0.01 | ||
| Type D Bearing | |||
| Pin Diameter | Rocker Radius | ||
| 2” | 6.5” | 0.0216 | |
| 2” | 7” | 0.0200 | |
| 2” | 7.5” | 0.0187 | |
| 2” | 8” | 0.0175 | |
| 2” | 10.5” | 0.0133 | |
| PTFE Bearing | 0.0600 | ||
The design of a bent with one of the above expansion bearings will be based on the maximum amount of load the bearing can resist by static friction. When this static friction is overcome, the longitudinal forces are redistributed to the other bents.
The maximum static frictional force at a bent is equal to the sum of the forces in each of the bearings. The vertical reaction used to calculate this maximum static frictional force shall be Dead Loads only for all loading cases. Since the maximum longitudinal load that can be experienced by any of the above bearings is the maximum static frictional force, the effects of longitudinal wind and temperature can not be cumulative if their sum is greater than this maximum static frictional force.
Two conditions for the bents of the bridge are to be evaluated.
- Consider the expansion bents to be fixed and the longitudinal loads distributed to all of the bents.
- When the longitudinal loads at the expansion bearings are greater than the static frictional force, then the longitudinal force of the expansion bearings is equal to the dynamic frictional force. It is conservative to assume the dynamic frictional force to be zero causing all longitudinal loads to be distributed to the remaining bents.
751.40.8.3 Unit Stresses
751.40.8.3.1 Fatigue in Structural Steel
Steel structures subjected to continuous reversal of loads are to be designed for fatigue loading.
ADTT, Average Daily Truck Traffic (one direction), shall be indicated on the Design Layout. Based on ADTT, the fatigue case and corresponding stress cycles can be obtained from AASHTO Table 10.3.2A.
When Case I fatigue is considered, it is necessary to check fatigue due to truck loading for both the 2,000,000 and over 2,000,000 stress cycles. For the over 2,000,000 stress cycles, the moment distribution factor for all stringers or girders (for fatigue stresses only) will be based on one lane loaded. For truck loading 2,000,000 cycles and lane loading 500,000 cycles, use the moment distribution factor based on two or more traffic lanes (same as for design moment).
The number of cycles to be used in the fatigue design is dependent on the case number and type of load producing maximum stress as indicated in AASHTO Table 10.3.2A. The allowable fatigue stress range based on the fatigue stress cycles can be obtained from AASHTO Table 10.3.1A.
The type of live load used to determine the number of cycles will be the type of loading used to determine the maximum stress at the point under consideration.
In continuous beams, the maximum stresses may be produced by the truck loading at some points, but by lane loading at other points. However, if the lane loading governs, then the longitudinal members should also be checked for truck loading.
Only live loading and impact stresses need to be considered when designing for fatigue.
Fatigue criteria applies only when the stress range is one of tension to tension or reversal. The fatigue criteria does not apply to the stress range from compression to compression.
All fracture critical structures, those which consist of only one or two main carrying members, trusses or single box girders, shall be considered as Non-redundant structures. Use the appropriate table which accompanies these structures.
751.40.8.3.2 Reinforced Concrete
Allowable Stresses of Reinforcing Steel
Tensile stress in reinforcement at service loads, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} :
| Concrete | ||
|---|---|---|
| Reinforcing Steel (Grade 40) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} | = 20,000 psi |
| Reinforcing Steel (Grade 60) | = 24,000 psi | |
For compression stress in beams, see AASHTO Article 8.15.3.5.
For compression stress in columns, see AASHTO Article 8.15.4.
For fatigue stress limit, see AASHTO Article 8.16.8.3.
Fatigue in Reinforcing Steel
For flexural members designed with reference to load factors and strengths by Strength Design Method, stresses at service load shall be limited to satisfy the requirements for fatigue. Reinforcement should be checked for fatigue at all locations of peak service load stress ranges and at bar cut-off locations except for concrete deck slab in multi-girder applications.
Allowable Stress Range: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fr_{allow}}
The allowable stress range is found using the equation listed below and the minimum stresses from dead load, live load, and impact based on service loads.
The term minimum stress level fmin for this formula indicates the algebraic minimum stress level: tension stress with a positive sign and compression stress with a negative sign.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fr_{allow} = 21 - 0.33 f_{min} + 8(r/h)}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fr_{allow}} | = allowable stress range (ksi) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_{min}} | = algebraic minimum stress level ksi): |
| positive if tension, negative if compression. | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, r/h} | = ratio of base radius to height of rolled-on transverse deformation; if the actual value is not know, 0.3 may be used. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle fr_{allow}} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 23.4 - 0.33 f_{min}} when Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, r/h = 0.3} |
Fatigue research has shown that increasing minimum tensile stress results in a decrease in fatigue strength for a tension to tension stresses case. The fatigue strength increases with a bigger compressive stress in a tension to compression stresses case.
Actual Stress Range:
The actual stress range, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fr_{act}} , is found using dead load, live load, and impact from service loads.
| = | ||
| = greatest tension stress level (ksi), always positive. | ||
| (Not necessary to check compression to compression for fatigue.) | ||
| = algebraic least stress level (ksi): | ||
| = positive if the least stress is tension | ||
| (tension to tension stresses) | ||
| = negative if the least stress is compression | ||
| (tension to compression stresses) | ||
Tension and Compression Stress Computation
Tension and compression stress are determined by using the following formulae for double reinforced concrete rectangular beams.
= tensile stress in reinforcement at service loads (ksi)
- Tensile stress
= compressive stress in reinforcement at service loads (ksi)
- Compressive stress
Where:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, j = \cfrac {k^2 \Big( 1 - \frac {k}{c} \Big) + 2 \rho'n \Big( k - \frac {d'}{d} \Big) \Big( 1 - \frac {d'}{d} \Big)}{k^2 + 2 \rho'n \Big( k - \frac {d'}{d} \Big)}} Eq. 2.2-1
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, k = \sqrt{2n \Bigg( \rho + \rho' \Bigg( \frac{d'}{d}\Bigg) \Bigg) + n^2 \big( \rho + \rho' \big)^2 - n \big( \rho + \rho' \big)}}
Eq. 2.2-2
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \rho} | = tension reinforcement ratio, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \rho = \frac{A_s}{bd}} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \rho'} | = compression reinforcement ratio, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \rho' = \frac{A'_s}{bd}} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A_s} | = area of tension reinforcement (sq. inch) |
| = area of compression reinforcement (sq. inch) | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, b} | = width of beam (inch) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, d} | = distance from extreme compression fiber to centroid of tension reinforcement (inch) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, d'} | = distance from extreme compression fiber to centroid of compression reinforcement (inch) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, jd} | = distance from tensile steel to resultant compression (inch) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, kd} | = distance from neutral plane to compression surface (inch) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} | = ratio of modulus of elasticity of steel to that of concrete |
751.40.8.4 Standard Details
751.40.8.4.1 Welding Details
All welding shall be detailed in accordance with ANSI / AASHTO / AWS D1.5, Bridge Welding Code.
For ASTM A709, Grade 36 steel (Service Load Design Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} = 58,000 psi) the allowable shear stress in fillet welds ( Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_V} ) is:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_V = 0.27 F_u}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_V} | = allowable basic shear stress |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} | = tensile strength of the electrode classification but not greater than the tensile strength of the connected part |
| Size of Fillet Weld (Inch) |
Allowable Shear Loads per Length (Pound per lineal inch) |
| 1/8” | 1,380 |
| 3/16” | 2,075 |
| 1/4" | 2,770 |
| 5/16” | 3,460 |
| 3/8” | 4,150 |
| 1/2" | 5,535 |
| 5/8” | 6,920 |
| 3/4" | 8,300 |
| 7/8” | 9,690 |
| 1” | 11,070 |
(*) Allowable Shear Load = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (0.27)(58000 psi)(0.707xSize of Weld)(L)}
Where:
| = Effective Length, in inch | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (0.707xSize of Weld)} | = Effective Throat, in inch |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (0.707xSize of Weld)(L)} | = Effective weld area in sq. inch |
751.40.8.4.2 Development and splicing of Reinforcement
751.40.8.4.2.1 General
Development of Tension Reinforcement
Development lengths for tension reinforcement shall be calculated in accordance with AASHTO Article 8.25. Development length modification factors described in AASHTO Articles 8.25.3.2 and 8.25.3.3 shall only be used in situations where development length without these factors is difficult to attain. All other modification factors shown shall be used.
Development lengths for tension reinforcement have been tabulated on the following pages and include the modification factors except those described above.
Lap Splices of Tension Reinforcement
Lap splices of reinforcement in tension shall be calculated in accordance with AASHTO Article 8.32.1 and 8.32.3. Class C splices are preferred when possible, however it is permissible to use Class A or B when physical space is limited. The designer shall satisfy AASHTO Table 8.32.3.2 when using Class A or B splices. It should be noted that As required is based on the stress encountered at the splice location, which is not necessarily the maximum stress used to design the reinforcement.
Temperature and shrinkage reinforcement is assumed to fully develop the specified yield stresses. Therefore the development length shall not be reduced by (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A_s} required)/(Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A_s} supplied).
Splice lengths for tension reinforcement have been tabulated on the following pages and include the development length modifications as described above.
Development of Tension Hooks
Development of tension hooks shall be calculated in accordance with AASHTO Article 8.29. Hook length modification factors described in Articles 8.29.3.3 and 8.29.3.4 shall only be used in situations where hook length without these factors is difficult to attain. All other modification factors shown shall be used.
Development lengths of tension hooks have been tabulated on the following pages and include the modification factors except those described above.
Development of Compression Reinforcement
Development lengths for compression reinforcement shall be calculated in accordance with AASHTO Article 8.26. Development length modification factors described in AASHTO Articles 8.26.2.1 and 8.26.2.2 shall only be used in situations where development length without these factors is difficult to attain. All other modification factors shown shall be used.
Development lengths for compression reinforcement have been tabulated on the following pages and include the modification factors except those described above.
Lap Splices of Compression Reinforcement
Lap splices of reinforcement in compression shall be calculated in accordance with AASHTO Article 8.32.1 and 8.32.4.
Splice lengths for compression reinforcement have been tabulated on the following pages.
Mechanical Bar Splices
Mechanical bar splices may be used in situations where it is not possible or feasible to use lap splices. Mechanical bar splices shall meet the criteria of AASHTO Article 8.32.2. Refer to the manufacturers literature for more information on the design of mechanical bar splices.
751.40.8.4.2.2 Development and Tension Lap Splice Lengths - Top Bars (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 60 ksi)

Top reinforcement is placed so that more than 12” of concrete is cast below the reinforcement.
Class A splice =1.0 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_d} , Class B splice =1.3 , Class C splice =1.7 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_d}
Use development and tension lap splices of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 4 ksi for concrete strengths greater than 4 ksi.
751.40.8.4.2.3 Development and Tension Lap Splice Lengths - Other Than Top Bars (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 60 ksi)

Class A splice =1.0 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_d}
, Class B splice =1.3 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_d}
, Class C splice =1.7 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_d}
Use development and tension lap splices of = 4 ksi for concrete strengths greater than 4 ksi.
751.40.8.4.2.4 Development and Lap Splice Lengths - Bars in Compression (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 60 ksi)

Development length for spirals, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_d}
, , should be used if reinforcement is enclosed in a spiral of not less than 1/4” diameter and no more than 4” pitch. See AASHTO 8.26 for special conditions.
All values are for splices with the same size bars. For different size bars, see AASHTO 8.32.4.
(*) Lap splices for #14 and #18 bars are not permitted except as column to footing dowels.
751.40.8.4.2.5 Development of Standard Hooks in Tension, Ldh ( = 60 ksi)
The development length, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_{dh}} , is measured from the critical section to the outside edge of hook. The tabulated values are valid for both epoxy and uncoated hooks.

Case A - For #11 bar and smaller, side cover (normal to plane of hook) less than 2 1/2 inches and for a 90 degree hook with cover on the hook extension less than 2 inches.
Case B - For #11 bar and smaller, side cover (normal to plane of hook) greater than 2 1/2 inches and for a 90-dgree hook with cover on the hook extension 2 inches or greater.
(*) See Structural Project Manager before using #14 or #18 hook.
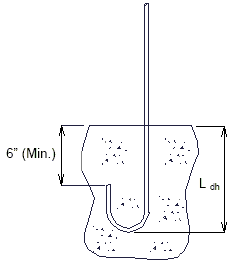
|
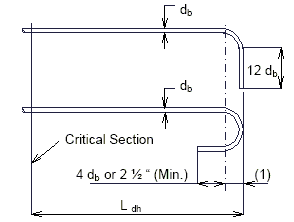
| |
| (1) = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \,4d_b} (#3 thru #8) | ||
| (1) = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \,5d_b} (#9, #10 and #11) | ||
| (1) = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \,6d_b} (#14 and #18) | ||
| DETAILS NEAR FREE EDGE <br\>OR CONSTRUCTION JOINT | HOOKED-BAR DETAILS FOR<br\>DEVELOPMENT OF STANDARD HOOKS |
|---|
751.40.8.4.2.6 Development of uncoated Grade 40 deformed bars in tension, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L_d} (AASHTO 8.25)
| Bar | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 3 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 4 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 5 ksi | |||
|---|---|---|---|---|---|---|
| Top bar | Top bar | Top bar | ||||
| #3 | 12 | 12 | 12 | 12 | 12 | 12 |
| #4 | 12 | 12 | 12 | 12 | 12 | 12 |
| #5 | 12 | 14 | 12 | 14 | 12 | 14 |
| #6 | 13 | 19 | 12 | 17 | 12 | 17 |
| #7 | 18 | 25 | 16 | 22 | 14 | 20 |
| #8 | 23 | 33 | 20 | 28 | 18 | 25 |
| #9 | 30 | 41 | 26 | 36 | 23 | 32 |
| #10 | 38 | 52 | 33 | 45 | 29 | 41 |
| #11 | 46 | 64 | 40 | 56 | 36 | 50 |
| #14 | 63 | 87 | 54 | 76 | 49 | 68 |
| #18 | 81 | 113 | 70 | 98 | 63 | 88 |
| Bar | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 3 ksi | = 4 ksi | = 5 ksi | |||
|---|---|---|---|---|---|---|
| Top bar | Top bar | Top bar | ||||
| #3 | 12 | 12 | 12 | 12 | 12 | 12 |
| #4 | 12 | 12 | 12 | 12 | 12 | 12 |
| #5 | 12 | 12 | 12 | 12 | 12 | 12 |
| #6 | 12 | 15 | 12 | 14 | 12 | 14 |
| #7 | 15 | 20 | 13 | 18 | 12 | 16 |
| #8 | 19 | 26 | 16 | 23 | 15 | 20 |
| #9 | 24 | 33 | 21 | 29 | 19 | 26 |
| #10 | 30 | 42 | 26 | 36 | 23 | 33 |
| #11 | 37 | 52 | 32 | 45 | 29 | 40 |
| #14 | 50 | 70 | 44 | 61 | 39 | 54 |
| #18 | 65 | 90 | 56 | 78 | 50 | 70 |
751.40.8.4.2.7 Minimum lap length for uncoated Grade 40 tension lap splices, (AASHTO 8.32)
| Other than Top Bars | Top Bars | |||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| = 3 ksi | = 4 ksi | = 5 ksi | = 3 ksi | = 4 ksi | = 5 ksi | |||||||||||||
| Bar | A | B | C | A | B | C | A | B | C | A | B | C | A | B | C | A | B | C |
| #3 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 16 | 21 | 12 | 16 | 21 | 12 | 16 | 21 |
| #4 | 12 | 12 | 14 | 12 | 12 | 14 | 12 | 12 | 14 | 12 | 16 | 21 | 12 | 16 | 21 | 12 | 16 | 21 |
| #5 | 12 | 13 | 17 | 12 | 13 | 17 | 12 | 13 | 17 | 14 | 19 | 24 | 14 | 19 | 24 | 14 | 19 | 24 |
| #6 | 13 | 17 | 22 | 12 | 16 | 21 | 12 | 16 | 21 | 19 | 24 | 31 | 17 | 22 | 29 | 17 | 22 | 29 |
| #7 | 18 | 23 | 30 | 16 | 20 | 26 | 14 | 19 | 24 | 25 | 32 | 42 | 22 | 28 | 37 | 20 | 26 | 34 |
| #8 | 23 | 30 | 40 | 20 | 26 | 34 | 18 | 24 | 31 | 33 | 42 | 55 | 28 | 37 | 48 | 25 | 33 | 43 |
| #9 | 30 | 38 | 50 | 26 | 33 | 43 | 23 | 30 | 39 | 41 | 54 | 70 | 36 | 47 | 61 | 32 | 42 | 54 |
| #10 | 38 | 49 | 63 | 33 | 42 | 55 | 29 | 38 | 49 | 52 | 68 | 89 | 45 | 59 | 77 | 41 | 53 | 69 |
| #11 | 46 | 60 | 78 | 40 | 52 | 68 | 36 | 46 | 61 | 64 | 84 | 109 | 56 | 72 | 95 | 50 | 65 | 85 |
| Other than Top Bars | Top Bars | |||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| = 3 ksi | = 4 ksi | = 5 ksi | = 3 ksi | = 4 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 5 ksi | |||||||||||||
| Bar | A | B | C | A | B | C | A | B | C | A | B | C | A | B | C | A | B | C |
| #3 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 16 | 21 | 12 | 16 | 21 | 12 | 16 | 21 |
| #4 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 16 | 21 | 12 | 16 | 21 | 12 | 16 | 21 |
| #5 | 12 | 12 | 14 | 12 | 12 | 14 | 12 | 12 | 14 | 12 | 16 | 21 | 12 | 16 | 21 | 12 | 16 | 21 |
| #6 | 12 | 14 | 18 | 12 | 13 | 17 | 12 | 13 | 17 | 15 | 19 | 25 | 14 | 18 | 23 | 14 | 18 | 23 |
| #7 | 15 | 19 | 24 | 13 | 16 | 21 | 12 | 15 | 20 | 20 | 26 | 34 | 18 | 23 | 29 | 16 | 21 | 27 |
| #8 | 19 | 24 | 32 | 16 | 21 | 28 | 15 | 19 | 25 | 26 | 34 | 44 | 23 | 29 | 38 | 20 | 26 | 34 |
| #9 | 24 | 31 | 40 | 21 | 27 | 35 | 19 | 24 | 31 | 33 | 43 | 56 | 29 | 37 | 49 | 26 | 33 | 44 |
| #10 | 30 | 39 | 51 | 26 | 34 | 44 | 23 | 30 | 39 | 42 | 54 | 71 | 36 | 47 | 62 | 33 | 42 | 55 |
| #11 | 37 | 48 | 63 | 32 | 42 | 54 | 29 | 37 | 49 | 52 | 67 | 87 | 45 | 58 | 76 | 40 | 52 | 68 |
751.40.8.4.3 Miscellaneous
Negative Moment Steel over Intermediate Supports
Dimension negative moment steel over intermediate supports as shown.
| Prestressed Structures: | |
|---|---|
| (1) | Bar length by design. |
| (2) | Reinforcement placed between longitudinal temperature reinforcing in top. |
Bar size: #5 bars at 7-1/2" cts. (Min.) #8 bars at 5" cts. (Max.)
| Steel Structures: | |
|---|---|
| (1) | Extend into positive moment region beyond "Anchor" Stud shear connectors at least 40 x bar diameter x 1.5 (Epoxy Coated Factor)(*) as shown below. (AASHTO 10.38.4.4 & AASHTO 8.25.2.3) |
| (2) | Use #6 bars at 5" cts. between longitudinal temperature reinforcing in top. |
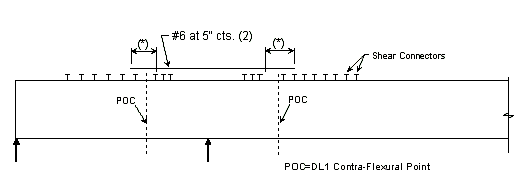
| (*) | 40 x bar diameter x 1.5 = 40 x 0.75" x 1.5 = 45” for #6 epoxy coated bar. |
751.40.8.5 General Superstructure
751.40.8.5.1 Concrete Slabs
751.40.8.5.1.1 Design Criteria
Slabs on Girders
Stresses:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_c} = 1,600 psi Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 4,000 psi Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} = 8 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 60,000 psi
Moments Over Interior Support (Use for positive moment reinf. also) (Sec. 1.5 E40A)
| Dead Load = | (Continuous over 5 supports) | |
| Dead Load = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, -0.100wS^2} | (Continuous over 4 supports) |
| Live Load = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (S + 2) \frac {P}{32}} | Continuity Factor | = 0.8 | |
| Impact Factor | = 1.3 | |||
| P | = 16 Kips for HS20 | |||
| P | = 20 Kips for HS20 Modified | |||
| Design Load: | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_u = 1.3 (M_{DL} + 1.67 M_{LL+I})} | |||
Cantilever Moment
Dead Load = Moment due to slab, F.W.S. and S.B.C.
Live Load:
Wheel Load = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_{LL=I} = \frac {Px}{E}}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} | = Wheel load (apply impact factor) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, x} | = Dist. from load to support (ft.) |
| = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 0.8x + 3.75} |
Collision Load = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle M_{COLL} = \frac {Py}{E}}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} | = 10 kips (Collision force) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, y} | = Moment arm (Curb ht.+ 1/2 Slab th.) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E} | = |
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, x} | = Dist. from C.G. of S.B.C. to support |
| The "support" is assumed at the 1/4 pt. of the minimum flange. | 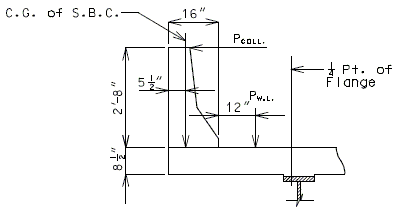
|
| Wheel loads and collision loads shall not be applied simultaneously. | |
| Use the greater of the two for the Design Load. | |
| Design Laod:<br./>Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_u = 1.3 (M_{DL} + 1.67 M_{LL+I})} | |
| Slab Cantilever Section |
|---|
Design of top reinf. is based on maximum moment over supports or cantilever moment. Flexural reinforcement shall meet the criteria of AASHTO Art. 8.16.3.
When designing for bottom transverse reinforcement, a 1" wearing surface is removed from the effective depth.
Prestressed panels replace the bottom transverse reinforcement.
Prestressed panels are assumed to carry DL1 stresses. Therefore, the negative moment due to DL1 at interior supports may be neglected.
The maximum P/S panel width (clear span + 6") for HS20 Modified is 9'-6". (Based on 10'-0" girder spacing and 10" flanges) The maximum P/S panel width (clear span + 6") for HS20 is 9'-11".
Distributioon of Flexural Reinforcement
Allowable Stress: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s = \frac {Z}{(d_c \times A)^{1/3}} \le 0.6 f_y}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Z} | = 130 k/in. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, d_c} | = Dist. from extreme tension fiber to center of closest bar (concrete cover shalll not be taken greater than 2") |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A} | = Effective tension area of concrete |
| = | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, s} | = Bar spacing ctr. to ctr. |
Actual Stress: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s = \frac {M_W}{A_S \times j \times d}}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_W} | = Service load moment |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A_S} | = Area of steel |
| = | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, k} | = |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_S/E_C} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \rho} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A_S /(b \times d)} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, b} | = Effective width |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, d} | = Effective depth |
Distribution of flexural reinforcement does not need to be checked in concrete considered unexposed to weather.
Longitudinal distribution reinforcement:
Top of slab - use #5 bars at 15" cts. for temperature distribution.
Bottom of slab - by design.
Negative moment reinforcement over supports:
Steel structures - add. #6 bars at 5" between #5 bars.
P/S girder structures - by design.
Additional reinforcement over supports shall be a minimum of #5 bars and a maximum of #8 bars at 5" ctrs. When necessary, replace the #5 temperature reinforcement with a larger bar to satisfy negative moment reinforcement requirement, but keep all bars within two bar sizes.
Note: See details of negative moment reinforcement.
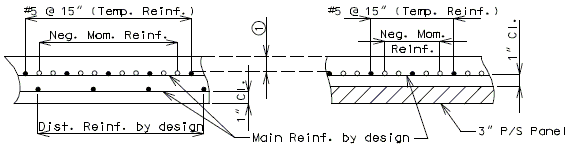
| |
| CIP Slab | P\S Panel Slab |
|---|---|
| 3" Cl. preferred min., 2 3/4" Cl. preferred min. for P/S panels to accommodate #8 bars over supports and 2 1/2" Cl. absolute min. by AASHTO 8.22.1. |
Method of measurement:
The area of the concrete slab shall be measured and computed to the nearest square yard. This area shall be measured transversely from out to out of slab and longitudinally from end to end of bridge slab.
Precast Prestressed Panels
3" Precast prestressed concrete panels with 5-1/2" minimum cast-in-place concrete will be the standard slab used on all girder superstructures except curved steel structures.
Concrete for prestressed panels shall be Class A1 with Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'{c}} = 6,000 psi, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_{ci}} = 3,500 psi. Prestressing tendons shall be uncoated, low-relaxation, seven-wire(7) strands for prestressed concrete conforming to AASHTO M203 Grade 270, with nominal diameter of strand = 3/8" and area = 0.085 sq.in., minimum ultimate strength = 22.95 kips (270 ksi), and strand spacing = 4.5 inches.
Panels shall be set on joint filler or polystyrene bedding material. Filler thickness shall be a Min. of 3/4" and a Max. of 2". Standard filler width is 1 1/2" except at splice plates where 3/4" Min. is allowed to clear splice bolts. Joint filler thickness may be reduced to a minimum of 1/4" over splice plates on steel structures. For prestressed girder structures, joint filler thickness may be varied within these limits to offset girder camber or at the contractor's option a uniform 3/4" (Min.) thickness may be used throughout. The same thickness shall be used under any one edge of any panel and the maximum change in thickness between adjacent panels shall be 1/4".
Standard roadway cross sections and slab reinforcement for HS20 and HS20 Modified live loads are shown in this section. Reinforcement shown is for a cast-in-place slab or a P/S panel slab with the bottom layer of reinforcement between girders being replaced by the panels. Cantilever reinforcement details for P/S panel slab are shown in this section.
- Maximum panel width (clear span + 6") = 9'-6" for HS20 Modified.
- Maximum panel width (clear span + 6") = 9'-11" for HS20.
When a safety barrier curb or median barrier curb is permanently required on the structure, other than at the edge of slab, precast prestressed panels will not be allowed in the bay underneath the curb. P/S panels are not allowed for use as simply supported for live loads, i.e. staging, where only two supports may be provided for live loads.
S.I.P.
Stay-in-place corrugated metal forms with cast-in-place concrete may be used on horizontally curved steel structures with the approval of the Structural Project Manager.
The standard slab reinforcements shown in this section for HS20 live load were designed using S.I.P. Dead Loads. If design is for HS20 Modified, the standard slab reinforcement needs to be checked for S.I.P. forms.
The bottom transverse reinforcement shall maintain a 1" clear distance from the top of forms.
C.I.P.
8 1/2" cast-in-place concrete slab with conventional forming may be used at the contractor's option, on all girder structures. Conventional forming shall also be used between girders with stage construction joints.
|
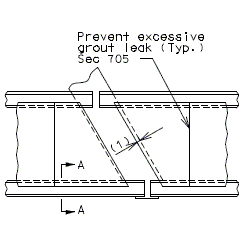
| |
| Panels-Squared Ends | Panels-Skewed Ends | |
|---|---|---|
| PLAN OF PRECAST PRESTRESSED PANELS PLACEMENT | ||
| (1) | End panels shall be dimensioned 1" min. to 1-1/2" max. from the inside face of diaphragm. |
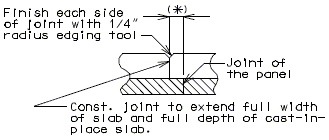
| |
| (2) | S-Bars shown are bottom steel in slab between panels and used with squared end panels only. | ||
| (3) | Extend S-Bars 18 inches beyond the front face of end bents only. | ||

|
Section Thru Const. Joint | ||
|---|---|---|---|
| (*) | Adjust the permissible construction joint to a clearance of 6 inches minimum from the joints of the panels. Note: All reinforcement other than prestressing strands shall | ||
| Section A-A | 
| ||
| (**) | 3/4" Min. thru 2" max. thickness and 1 1/2" width of preformed fiber expansion joint material or Sec 1057 or polystyrene bedding material Sec 1073. | ||
| Section Thru Cantilever | |||
|
|
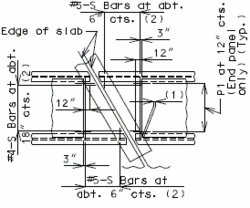
| |
| End Bent | End Bent (Integral) | Int. Bent (Exp. Gap) | |
|---|---|---|---|
| Panels-Squared Ends | |||
|
|
| |
| Int. Bent (Exp. Gap) | End Bent | End Bent (Integral) | |
| Panels-Skewed Ends | |||
| PLAN OF PRECAST PRESTRESSED PANELS PLACEMENT | |||
| (1) | End panels shall be dimensioned 1" min. to 1 1/2" max. from the inside face of diaphragm. | 
| |
| (2) | S-Bars shown are bottom steel in slab between panels and used with squared end panels only. | ||
| (3) | Extend S-bars 18 inches beyond the front face of end bents only. | Section A-A (*) Over splice plates, 3/4" Min. thickness allowed. | |
| (5) | S-Bars shown are used with skewed end panels, or square end panels of square structures only. The #5-S Bars will extend the width of slab (30" lap if necessary) or to within 3" of expansion device assemblies. | 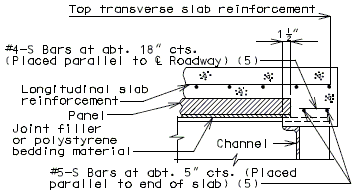
| |
| Note: | All reinforcement other than prestressing strands shall be epoxy coated. | ||
| Part Section B-B | |||
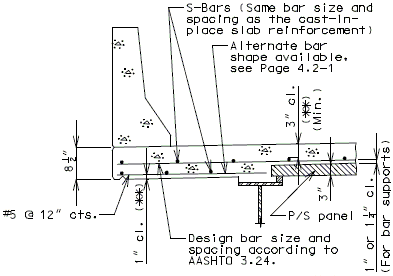
| |||
| Section Thru Cantilever | |||
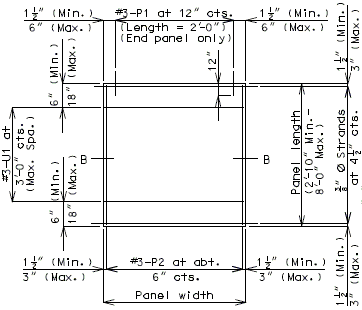
|

| ||
| Plan of Precast Prestressed Panel | Plan of Precast Prestressed Panel (Skewed End-Optional) | ||
|---|---|---|---|
| (*) | = 3" (Typ.) for steel girder structures | 
| |
| (*) | = 3" (Typ.) for P/S girder structures | ||
| (**) | Use #3-P3 bars if panel is skewed Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \,45^\circ} or greater. | ||
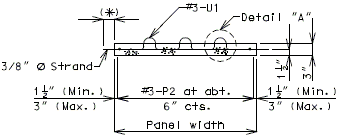
|
Detail "A" | ||
| Note: | Area of Strand = Astra = 0.085 sq. in./strand Initial prestressing stress = fsi = (0.75)(270 ksi) = 202.5 ksi Initial prestressing force = Astra x fsi = (0.085 sq. in./strand)(202.5 ksi) = 17.2 kips/strand | ||
| Section B-B | |||
751.40.8.5.1.2 Details of Concrete Slabs for Structures
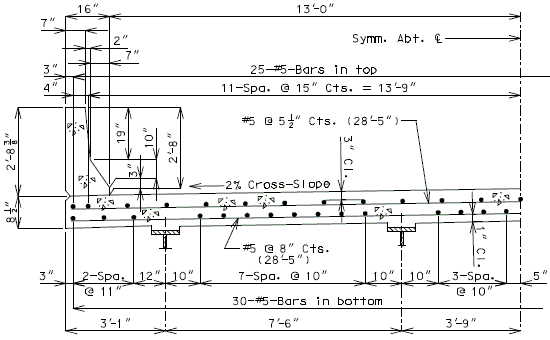
| |
| HS20 (26'-0" ROADWAY - 4 GIRDER) | |
|---|---|
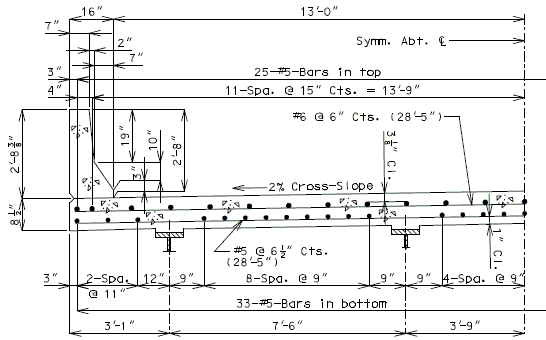
| |
| HS20 Modified (26'-0" ROADWAY - 4 GIRDER) | |
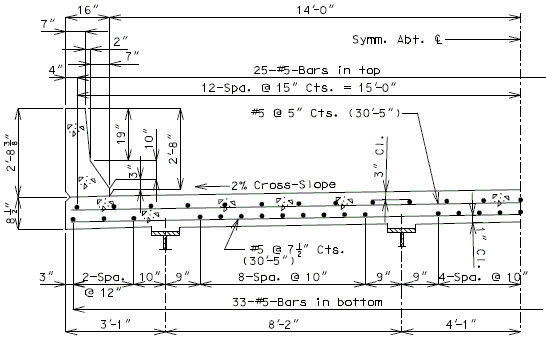
| |
| HS20 (28'-0" ROADWAY - 4 GIRDER) | |

| |
| HS20 Modified (28'-0" ROADWAY - 4 GIRDER) | |
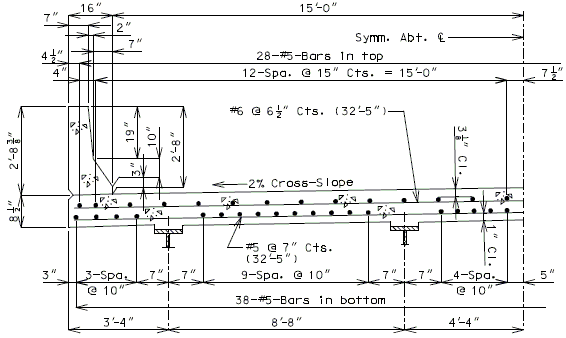
| |
| HS20 (30'-0" ROADWAY - 4 GIRDER) | |
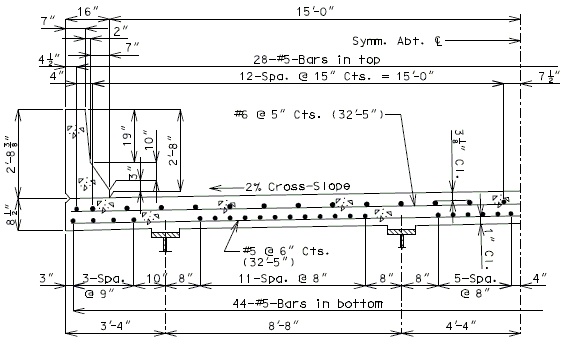
| |
| HS20 Modified (30'-0" ROADWAY - 4 GIRDER) | |
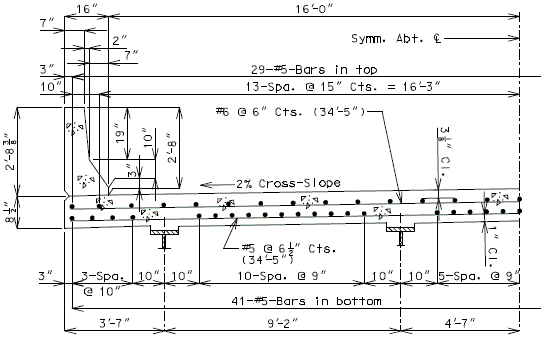
| |
| HS20 (32'-0" ROADWAY - 4 GIRDER) | |
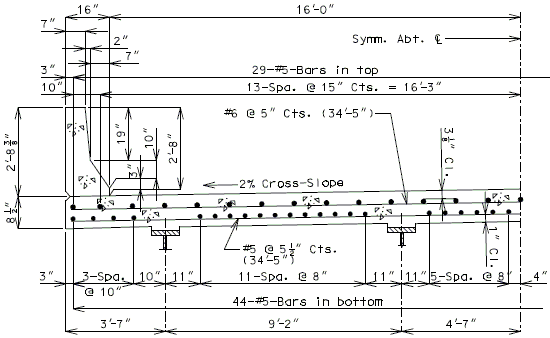
| |
| HS20 Modified (32'-0" ROADWAY - 4 GIRDER) | |
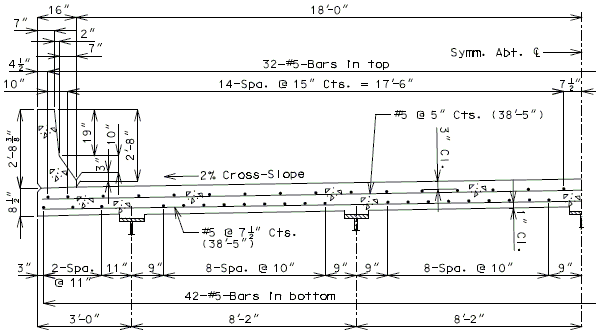
| |
| HS20 (36'-0" ROADWAY - 5 GIRDER) | |
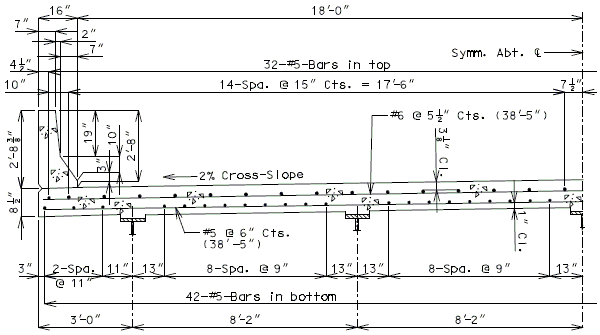
| |
| HS20 Modified (36'-0" ROADWAY - 5 GIRDER) | |
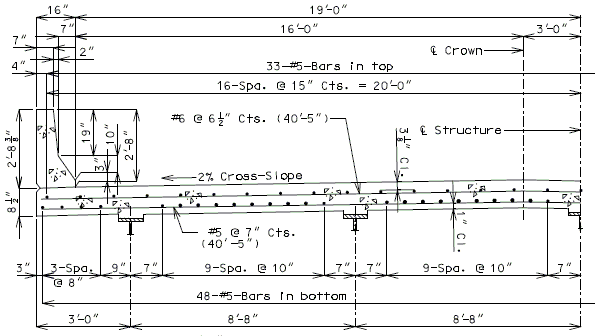
| |
| HS20 (38'-0" ROADWAY - 5 GIRDER)(Unsymmetrical) | |
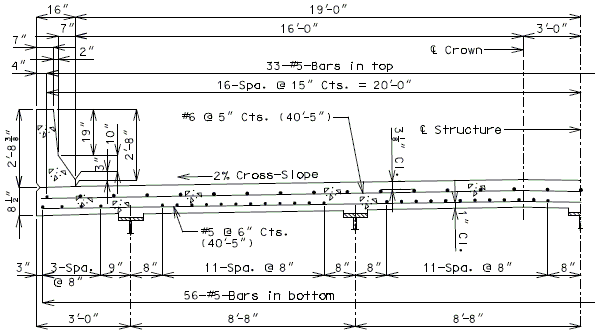
| |
| HS20 Modified (38'-0" ROADWAY - 5 GIRDER)(Unsymmetrical) | |
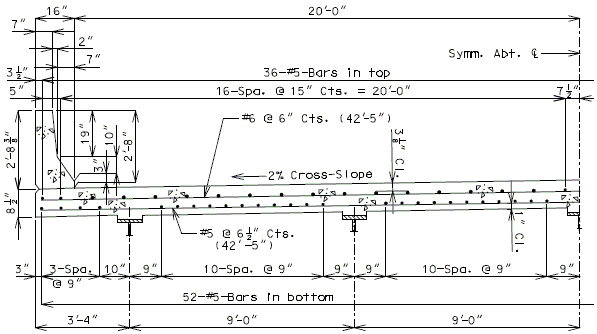
| |
| HS20 (40'-0" ROADWAY - 5 GIRDER) | |
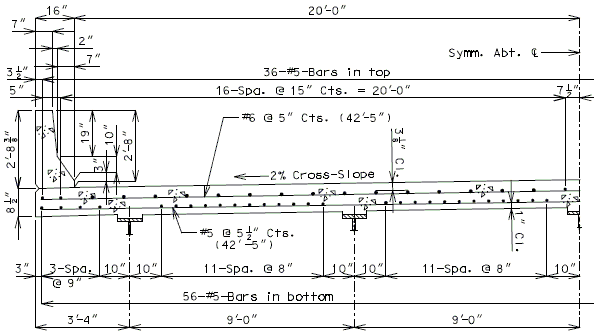
| |
| HS20 Modified (40'-0" ROADWAY - 5 GIRDER) | |
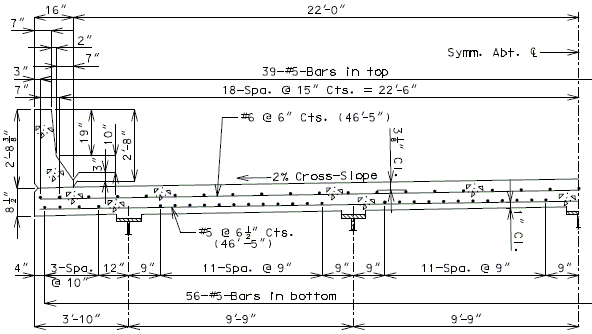
| |
| HS20 (36'-0" ROADWAY - 5 GIRDER) | |
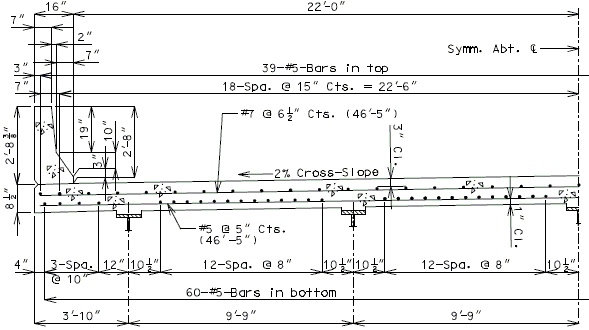
| |
| HS20 Modified (36'-0" ROADWAY - 5 GIRDER) | |
751.40.8.5.2 Timber Floor
| Maximum stringer spacing as determined by strength of timber floor | ||
|---|---|---|
| Stress = 1,200 lbs. per square inch | ||
| H-10 | H-15 | |
| (*) 3" x 12" Plank | 18" + 1/2 Flange Width | 16" + 1/2 Flange Width |
| 4" Laminated Floor | 2'-11" + 1/2 Flange Width | 2'-3" + 1/2 Flange Width |
| 6" Laminated Floor | 6'-0" + 1/2 Flange Width | 4'-4" + 1/2 Flange Width |
| Stress = 1,600 lbs. per square inch | ||
| H-10 | H-15 | |
| 3" x 12" Plank | 23" + 1/2 Flange Width | 21" + 1/2 Flange Width |
| 4" Laminated Floor | 3'-9" + 1/2 Flange Width | 2'-11 1/2" + 1/2 Flange Width |
| 6" Laminated Floor | 7'-10 3/4" + 1/2 Flange Width | 5'-9" + 1/2 Flange Width |
751.40.8.5.3 Steel Grid Bridge Flooring
In general, the 5" depth (concrete filled to half depth) steel grid bridge flooring shall be specified. Bar spacing may vary as necessary to meet minimum section modulus requirements. Main member spacing shall not exceed 10" and cross bar spacing shall not exceed 4". At present, the manufacturers of the following types have provided data to show they are acceptable:
- Greulich 5" Standard
- Foster 5" Standard
The section properties Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (n = 8)} and maximum span for HS20 loading have been computed for these types and are as follows:
| Company | (For Design Purpose only) Weight (PSF) (Steel & Conc.) |
Main bar Spacing |
Cross bar Spacing |
Moment of Inertia Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (in^4/Ft.)} | ||
| Mid Span | Over-Support | |||||
| Conc. | Steel | Steel | ||||
| Greulich | 48.0 | 7 1/2" | 3 3/4" | 99.41 | 12.43 | 9.03 |
| Foster | 48.0 | 8" | 4" | 128.1 | 16.01 | 12.25 |
| Company | Section Modulus Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (in^2/ft.)} | Maximum Span (*) | ||||||
| Mid-Span | Over-Support | Simple Span | Continuous Spans | |||||
| Conc. (Top) |
Steel (Bott.) |
Steel (Top) |
Steel (Bott.) |
ASTM A709 Gr. 36 |
ASTM A709 Gr. 50W |
ASTM A709 Gr. 36 |
ASTM A709 Gr. 50W | |
| Greulich | 59.5 | 3.53 | 3.90 | 3.14 | 4'-4" | 5'-10" | 5'-10" | 7'-1" |
| Foster | 72.5 | 4.68 | 5.25 | 4.30 | 5'-9" | 7'-5" | 7'-2" | 9'-4" |
The cross-section DETAILS used in computing the section properties are shown on the sketches on the following sheets. Maximum span determination included an allowance for a 35#/sq.ft. future
wearing surface and assumed a wheel load to be distributed, normal to the main bars, over a width of 4'-0".
(Place the following note on the Bridge Plans with the Steel Grid Details.
Note: The steel grid deck shall be electrically grounded.
(*) For main beams of grid either parallel or perpendicular to traffic.
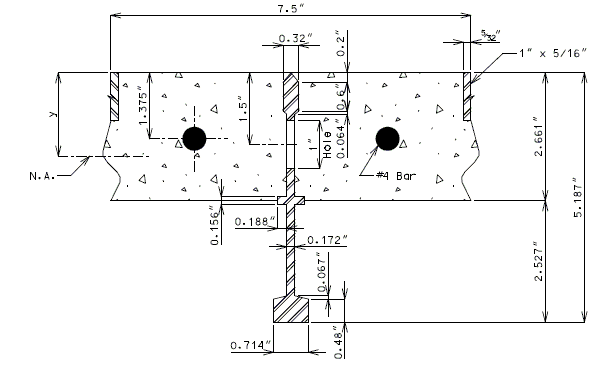
| Composite Section | Seel Section Only (net) | |
| y | 1.671" | 2.317" |
| Note: | Dimensions obtained form Greulich plans. |
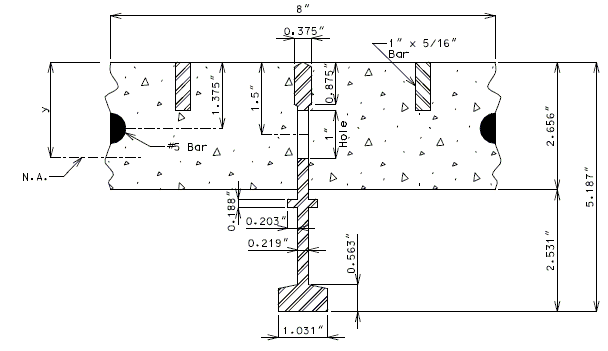
| Composite Section | Seel Section Only (net) | |
| y | 1.766" | 2.338" |
| Note: | Dimensions obtained form Foster Catalog. |
751.40.8.5.4 Longitudinal Diagrams
751.40.8.5.4.1 Hinged Beam Connections
The diagrams of various joints in steel structures are intended to be guides primarily for the determination of horizontal longitudinal dimensions for the plan view on the first sheet of plans.
These diagrams are not to be detailed on the design plans. However, the arrangement of the joints should be useful in detailing the longitudinal diagram for structural steel, particularly for bridges on grades and vertical curves.
Longitudinal dimensions for the plan of structural steel and for the plan of slab shall be horizontal from centerline bearing to centerline bearing.
For proper correlation of details when developing plans for widening or redecking bridges, match the method of dimensioning on the new plans with the method used on the originals.
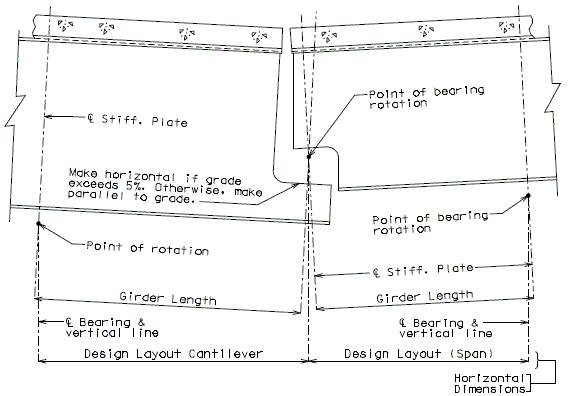
|
| Geometrics for Hinged Beam Connections for Bridges on Sag Vertical Curves |
|---|
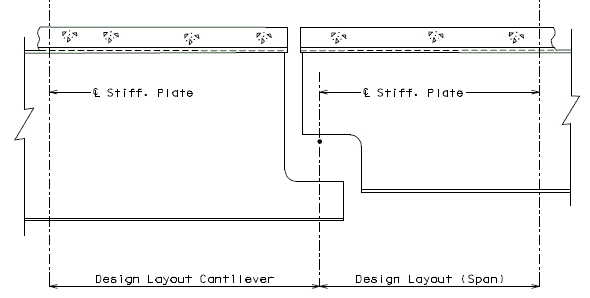
|
| Geometrics for Hinged Beam Connections for Bridges on Flat Grade |
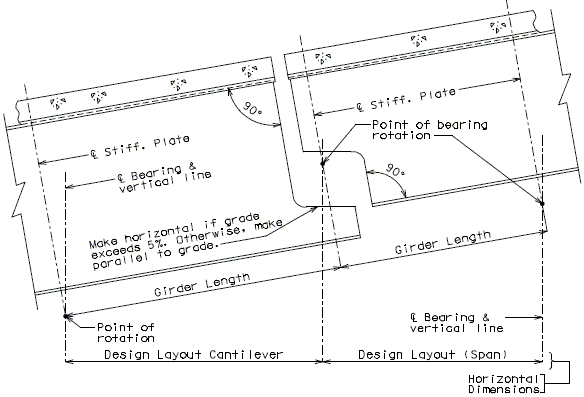
|
| Geometrics for Hinged Beam Connections for Bridges on Straight, Plus Grades |

|
| Geometrics for Hinged Beam Connections for Bridges on Crown Vertical Curves |
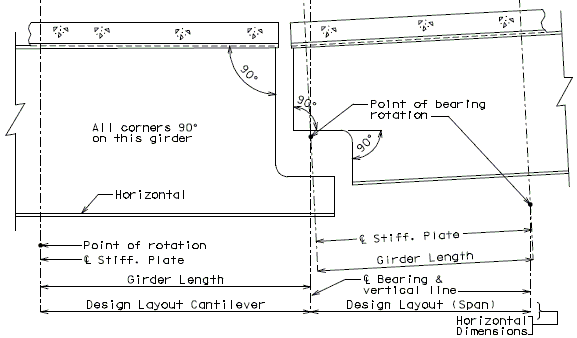
|
| Geometrics for Hinged Beam Connections for Bridges on Sag Vertical Curves |
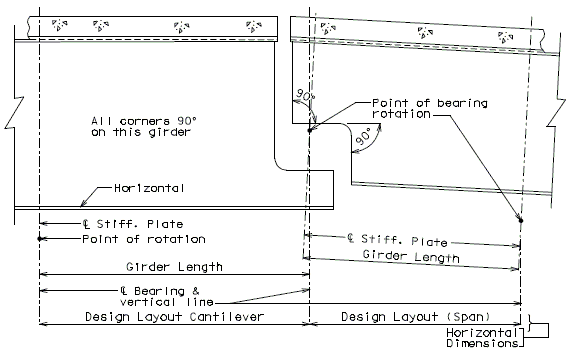
|
| Geometrics for Hinged Beam Connections for Bridges on Symmetrical Vertical Curves |
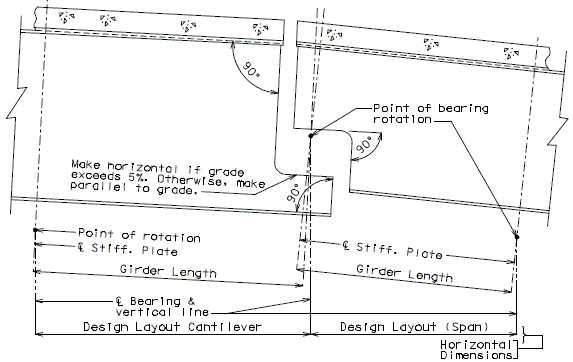
|
| Geometrics for Hinged Beam Connections for Bridges on Crown Vertical Curves |
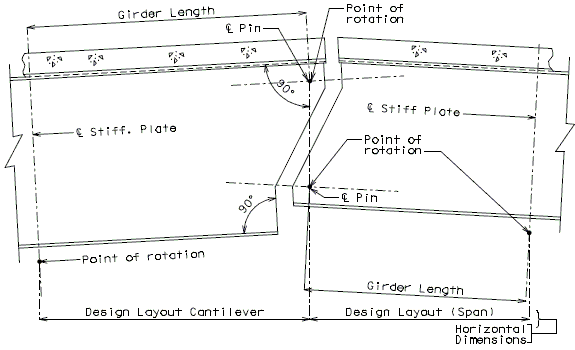
|
| Geometrics for Hanger Beam Connections for Bridges on Crown Vertical Curves |
|---|
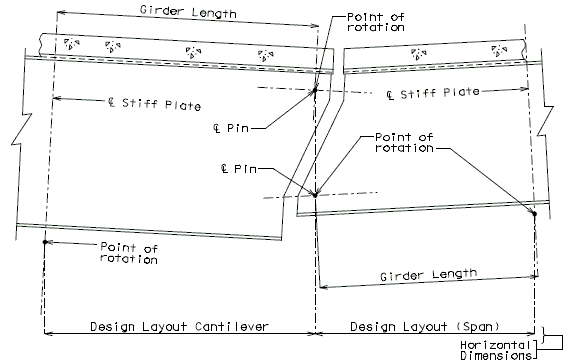
|
| Geometrics for Hanger Beam Connections for Bridges on Sag Vertical Curves |
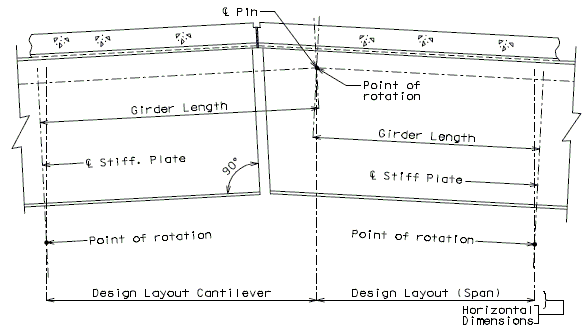
|
| Geometrics for Pin Plate Connections for Bridges on Crown Vertical Curves |
|---|
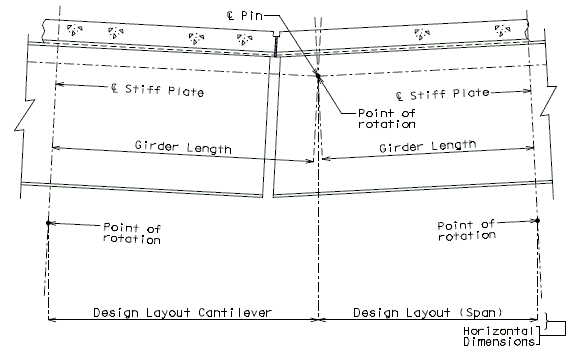
|
| Geometrics for Pin Plate Connections for Bridges on Sag Vertical Curves |
751.40.8.5.4.2 Longitudinal Sections
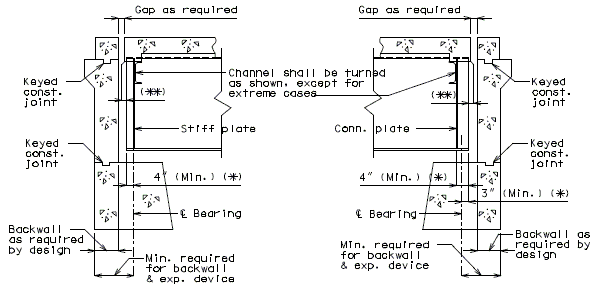
| |
| Bearing Stiffener | Connection Plate |
|---|---|
| Structures Not on Grade (Typical) | |
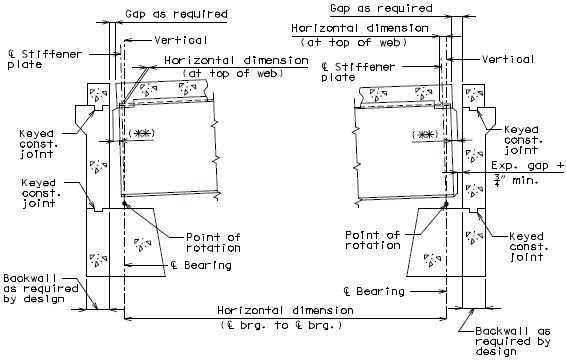
| |
| Structures on Grade (Typical) | |
| (*) | Parallel to Girder. All other dimensions shown are normal to backwall. |
| (**) | See EPG 751.13 Expansion Devices for dimension of overhang from end of stringer or girder to face of plate, edge of concrete or face of vertical leg of angle. |
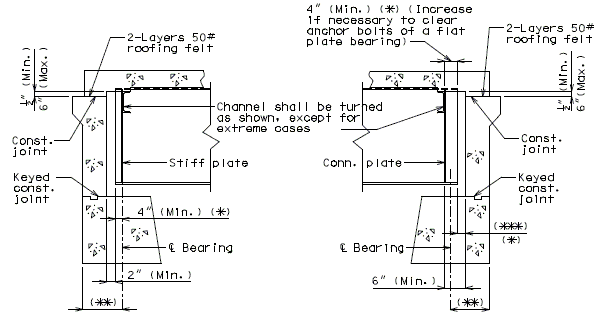
| |
| Bearing Stiffener | Connection Plate |
|---|---|
| Structures Not on Grade (Typical) | |

| |
| Structures on Grade (Typical) | |
| (*) | Parallel to Girder. All other dimensions shown are normal to backwall. |
| (**) | 18" min. (Use same dimension as the expansion device end on 3-span continuous, if it is not more than 2" greater.) |
| (***) | 3" min. for type C, D and E bearing, and 2" min. for an elastomeric bearing. |
|
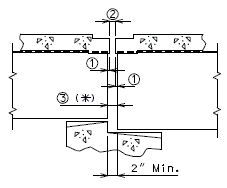
|
| No Expansion Device | Expansion Device |
|---|
| 1/2" minimum overhang from end of stringer to face of plate, edge of concrete or face of vertical leg of angle. | |
| Gap as required for a particular type of expansion device. | |
| Expansion device gap plus 1 1/2" minimum (taken parallel to centerline stringer). | |
| (*) | Parallel to Girder. All other dimensions shown are normal to centerline Bent. |
| Blockout shown is for Elastomeric Expansion Joint Seal. Check Design Layout for type of device for a particular structure. | |
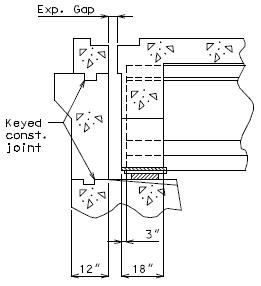
|
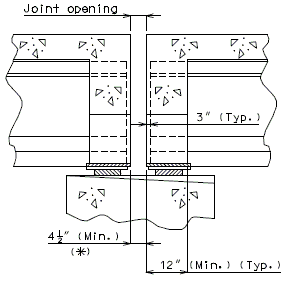
|
| Structures Not on Grade (Typical) | |
|---|---|
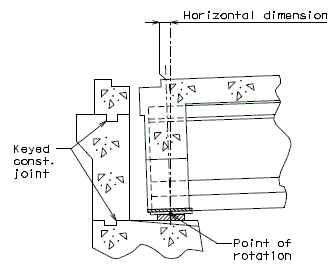
| |
| Structures On Grade (Typical) | |
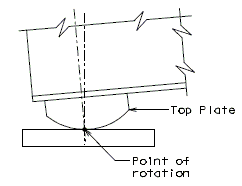
|
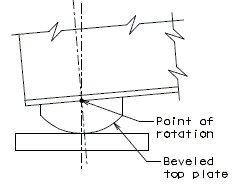
|
| Type "C" Bearing | Type "C" Bearing (Grade 4% and Greater) |
|---|---|
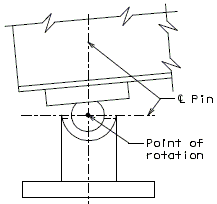
|
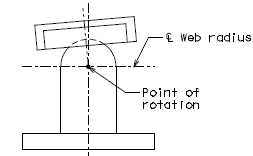
|
| Type "D" Bearing | Type "E" Bearing |

|

|
| Flat Plate Bearing (For Grade 2% and Greater) |
Prestressed Structure Bearing Pad |
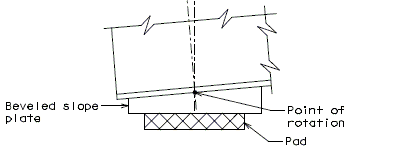
| |
| Steel Structure Bearing Pad | |
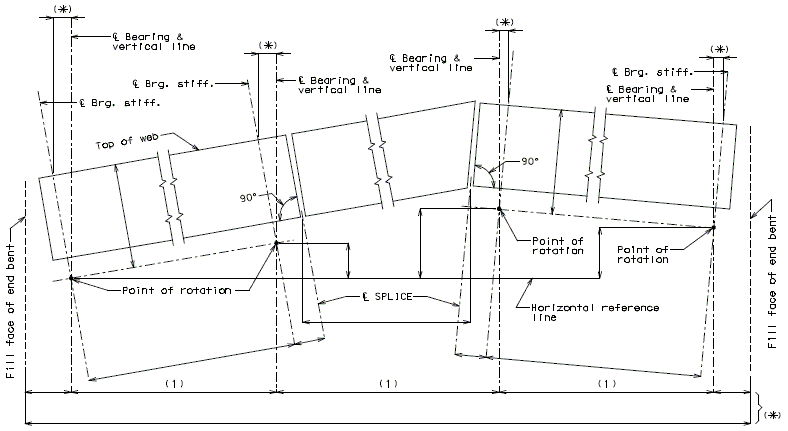
|
| Elevation of Longitudinal Steel Diagram |
|---|
| Note: | The typical elevation shown above should be detailed on the plans for all steel structures that are on vertical curve grades. |
| (1) | Longitudinal dimensions are horizontal from centerline Brg. to centerline Brg. |
| (*) | Horizontal dimensions. |
| BLOCKING DIAGRAM SHOULD NOT BE USED FOR CAMBERED GIRDERS. | |
|---|---|
751.40.8.5.5 Miscellaneous Bearing Connections
751.40.8.5.5.1 Typical Details of “Hinged Connection"
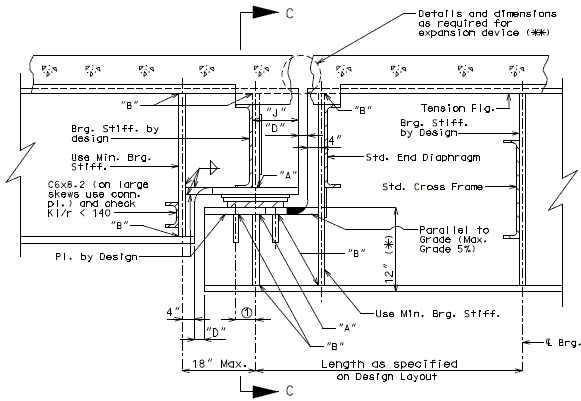
| ||||||||||||||||
| Section Showing Hinged Beam Connection | ||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
|
| ||||||||||||||
| Plan of Brg. Plate | ||||||||||||||||
| Detail of Web at Radius Transition |
Typical Welding Details for Stiff. Plates | |||||||||||||||
|
| |||||||||||||||
| Section C-C | ||||||||||||||||
| |||
| Section Showing Hinged Beam Connection | |||
|---|---|---|---|
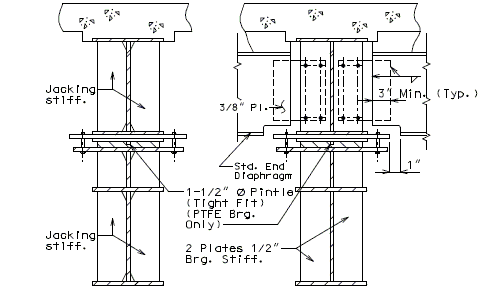
| |||
| Section D-D | Section E-E | ||
| |||
| Typical Welding Details for Stiff. Plates | |||
| (*) | See below for dimension "G". |
| (**) | See EPG 751.13 Expansion Devices |
| "F" | = Gap as required for expansion (3" Min.). |
| "H" | = 10 3/4" Min. (12" preferred.) |
| "J" | = 5" for bearing with 3" web thickness. Use 6" for all others. |
| All dimensions shown are minimum, increase, as necessary. | |
| Web Thickness |
Depth "G" |
(*) Allowable Dead Load Reactions, Kips (At 150% Overstress) |
Web Thickness |
Depth "G" |
(*) Allowable Dead Load Reactions, Kips (At 150% Overstress) | |
|---|---|---|---|---|---|---|
| 5/16" | 8" | 45.0 | 7/16" | 8" | 63.0 | |
| 5/16" | 9" | 50.6 | 7/16" | 9" | 70.8 | |
| 5/16" | 10" | 56.2 | 7/16" | 10" | 78.7 | |
| 5/16" | 11" | 61.8 | 7/16" | 11" | 86.6 | |
| 5/16" | 12" | 67.5 | 7/16" | 12" | 94.5 | |
| 5/16" | 13" | 73.1 | 7/16" | 13" | 102.3 | |
| 5/16" | 14" | 78.8 | 7/16" | 14" | 110.2 | |
| 5/16" | 15" | 84.3 | 7/16" | 15" | 118.1 |
| 3/8" | 8" | 54.0 | 1/2" | 8" | 72.0 | |
| 3/8" | 9" | 60.7 | 1/2" | 9" | 81.0 | |
| 3/8" | 10" | 67.5 | 1/2" | 10" | 90.0 | |
| 3/8" | 11" | 74.2 | 1/2" | 11" | 99.0 | |
| 3/8" | 12" | 81.0 | 1/2" | 12" | 108.0 | |
| 3/8" | 13" | 87.7 | 1/2" | 13" | 117.0 | |
| 3/8" | 14" | 94.5 | 1/2" | 14" | 126.0 | |
| 3/8" | 15" | 101.2 | 1/2" | 15" | 135.0 |
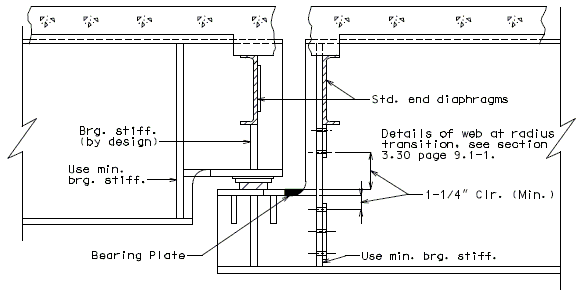
|
| Section Showing Hinged Beam Connection |
|---|
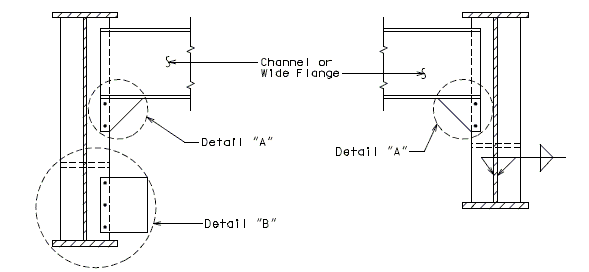
| |
| Plate Girder 42" Thru 46" Also 48" and Over |
Plate Girder 36" Thru 40" |
|---|---|
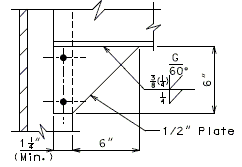
|
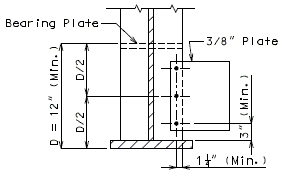
|
| Detail "A" | Detail "B" |
|---|
| Note: | Modify standard end diaphragm connections as shown above, if clearance problems exist between bearing plate and end diaphragm connection bolts. |
751.40.8.6 Composite Design
751.40.8.6.1 General
GENERAL
This portion of the article pertains to structures composed of steel girders with concrete slab connected by shear connectors. The stresses of composite girders and slab shall be computed based on the composite cross-section properties and shall be consistent with the properties of the various materials used. The regions subjected to positive moment are considered as composite and the regions subjected to negative moment are considered as non-composite. For the initial girder design, composite/non-composite regions can be approximately assumed as:
SECTION PROPERTIES
Cross-section properties of the composite section shall include concrete slab and steel section.
Cross-section properties of the non-composite section shall include steel section only.
Use composite property for positive moment section.
Use non-composite property for negative moment section. The effect of reinforcing steel in the section is not considered.
The ratio of modulus of elasticity of steel to that of concrete, n, shall be assumed to be eight. The effect of creep shall be considered in the design of composite girders which have dead loads acting on the composite section. In such structures, n=24 shall be used.
DESIGN UNIT STRESSES (also see note A1.1 in Section 4)
Reinforcement Concrete
| Reinforcing Steel (Grade 60) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 24,000 psi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 60,000 psi |
| Class B-2 Concrete (Substructure) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_c} = 1,600 psi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 4,000 psi |
Structural Steel
| Structural Carbon Steel (ASTM A709 Grade 36) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 20,000 psi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 36,000 psi |
| Structural Steel (ASTM A709 Grade 50) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 27,000 psi | = 50,000 psi |
| Structural Steel (ASTM A709 Grade 50W) | = 27,000 psi | = 50,000 psi |
751.40.8.6.2 Design
751.40.8.6.2.1 Shear Connector Design
The shear connectors shall be designed for fatigue and checked for ultimate strength (AASHTO Article 10.38.5.1).
Step 1:
Compute Vr, the range of shear in kips, from the structural analysis, due to live loads and impact, for entire span.
At any section, the range of shear shall be taken as the difference in the minimum and maximum shear envelopes (excluding dead loads).
Step 2:
Use the average Vr per span, for the section of the span that is assumed to act compositely (from end of span to point of contraflexure for end spans, or from point of contraflexure to point of contraflexure for int. spans).
Step 3:
Using the average Vr from step 2, compute the range of horizontal shear load per linear inch, Sr in kips per inch, at the junction of the slab and stringer from the following equation:
(AASHTO Article 10.38.5.1.1 Eq. 10-58)
where:
= static moment of the transformed compressive concrete area about the neutral axis of the composite section, in cubic inches (*);
= moment of inertia of the transformed composite girder in positive moment regions in inches to the fourth power (*).
(*) In the formula, the compressive concrete area is transformed into an equivalent area of steel by dividing the effective concrete flange width by the modula ration n=8.
Step 4:
Compute the allowable range of horizontal shear, Zr, in pounds on an individual connector, welded stud, by use of the following formula:
where:
=height of stud in inches;
=diameter of stud in inches;
=13,000 for 100,000 cycles
- 10,600 for 500,000 cycles
- 7,850 for 2,000,000 cycles
- 5,500 for over 2,000,000 cycles.
Step 5:
Compute the number of additional connectors required at point of contraflexure, N , from the following formula:
Pitch =
Where: Pitch = required pitch, in inches;
= the resistance of all connectors at one (1) transverse girder cross-section as a shear connector unit.
Note:
The pitch is to be constant and spaced in the composite section (round to the nearest inch).
Step 6:
Compute the required pitch of the shear connector units, pitch by the following formula:
(AASHTO Article 10.38.5.1.1 Eq. 10-69)
where:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, N_c} = number of additional connectors required at the point of contraflexure;
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, {A_r}^s } = total area of longitudinal slab reinforcing steel for each girder over interior support;
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_r} = range of stress due to live load plus impact, in the slab reinforcement over the support (in lieu of more accurate computations, f may be taken as equal to 10,000 psi);
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Z_r} = the allowable range of horizontal shear on an individual connector.
This number of additional connectors, N , shall be placed adjacent to the point of dead load contraflexure within a distance equal to 1/3 of the effective slab width, if it is possible. If it is impossible, use minimum pitch of 6".
Step 7: Check connectors for ultimate strength
The number of connectors provided for fatigue must be checked to ensure that adequate connectors are provided for ultimate strength.
To check for ultimate strength, proceed as follows:
(1) Compute the force in the slab (P), which is defined as: at the point of maximum positive moment, the force in the slab is taken as the smaller value of the following two formulae:
(AASHTO Article 10.38.5.1.2 Eq. 10-63)
or
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P_2 = 0.85 f'_{cbt_s} P} = (AASHTO Article 10.38.5.1.2 Eq. 10-62)
Where:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A_s} = total area of the steel section including cover plates (if used);
= specified minimum yield point of the steel being used;
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = compressive strength of concrete at age of 28 days;
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, b} = effective flange width given in AASHTO Article 10.38.3;
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, t_s} = thickness of concrete slab.
Note:
If it becomes impractical to place the number of shear connectors required by ultimate strength in the specified distance (structures with span ratios greater than 1.5); base the number and spacing of shear connectors on the fatigue analysis only.
Increase the haunch by 1/2"± more than what is required to make one size shear connector work for C.I.P. or S.I.P. option.
751.40.8.6.2.2 Shear Connector Spacing
If it becomes impractical to place the number of shear connectors required by ultimate strength in the specified distance (structures with span ratios greater than 1.5); base the number and spacing of shear connectors on the fatigue analysis only.
For a typical 3-spans bridge, the shear connector units can be approximately arranged as below:
751.40.8.6.3 Details
751.40.8.6.3.1 Shear Connector Details
Use precast prestressed panels on all tangent steel structures. Evaluate the viability of the use of P/S panels on curved structures on a case by case basis and use or include as an option to a CIP slab where deemed appropriate.
Whenever panels are used, the minimum top flange width shall be 12" for Plate Girders and 10" for Wide Flange Beams.
Steel girders shall be cambered when using P/S Panels. Minimum joint filler thickness is 3/4", except over splice plates, in which case use 1/4" minimum. Maximum joint filler thickness is 2".
Shear connectors shall have a minimum height equal to the top of panel.
Shear connectors shall be spaced by units and shear connectors in each unit shall be placed along of girder. On wide flange widths, two lines of connectors may be used if spacings and clearances allow.
Additional shear connectors, Nc, at point of contraflexure may be placed in units normal to girder as space allows or in a single row along girder as shown below:
P/S strands shall extend 3" minimum and 6" maximum past edge of precast prestressed panel and not closer than 1" to the adjacent panels.
Panel end at splices shall be notched to avoid bolt heads as shown below:
![]() 3/4" min. wide bearing edge for panel at splice, typ. (*)
3/4" min. wide bearing edge for panel at splice, typ. (*)
![]() 4 x (Stud diameter) preferred minimum, may be reduced if necessary for a more economical design; 2-1/4" absolute minimum.
4 x (Stud diameter) preferred minimum, may be reduced if necessary for a more economical design; 2-1/4" absolute minimum.
(*) In order to meet ![]() and
and ![]() above, it is necessary to have an edge bolt distance of 2" or greater for splice plate. For unusual cases, which would require field splices for flange widths 14" or 15" for P/S precast panel option, it will be necessary to change the top flange width to either 13" or 16" of equal area to maintain the 3/4" minimum panel bearing edge on the splice plates.
above, it is necessary to have an edge bolt distance of 2" or greater for splice plate. For unusual cases, which would require field splices for flange widths 14" or 15" for P/S precast panel option, it will be necessary to change the top flange width to either 13" or 16" of equal area to maintain the 3/4" minimum panel bearing edge on the splice plates.
Minimum joint filler thickness is 3/4" except over splice plates in which case use 1/4" minimum. When joint filler is less than 1/2" thick over splice plate, make the width of joint filler at splice the same width as panel on splice (maximum 1-1/2" wide).
Maximum difference in top of flange thickness should be checked so that joint filler thickness does not exceed 2".
751.40.8.6.3.2 Deflection
Allowable Live Load Deflection
| 1. | Composite Design: | Defl. | = 1/1000 of span; |
| 2. | Non-composite Design: | Defl. | = 1/800 of span |
- Where:
- Defl. = allowable deflection due to service live load plus impact.
Dead Load Deflection
Compute at 1/4 point for bridge with spans less than 75’, at 1/10 points for spans 75’ and over.
751.40.8.7 Wide Flange Beam Spans
751.40.8.7.1 Design
751.40.8.7.1.1 Design Data
Slabs
| Reinforcing Steel | = 60,000 psi | ||||
| Concrete | = 1,600 psi | = 4,000 psi | |||
| = 8 |
Simple Design Span
Design Span = Center to Center of Bearings.
Dead Load
Live Load Distribution Factors
See EPG 751.40.8.2.2 Distribution of Live Load
Live Load Deflection Allowable
| Composite | |
| Non-Composite |
Live Load Reaction
Live Load Wheel Line is the Live Load Reaction per Wheel Line, no distribution, no impact; Maximim Live Load Impact is the Live Load Reaction x Distribution Factor = Impact.

Typical Continuous Steel Structures - Integral End Bents:
751.40.8.7.1.2 Stringer Design
Stresses:
| Steel: | AASHTO - Article 10.2, 10.32 | ||
| ASTM A709 Grade 36 | = 36,000 psi | ( Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 20,000 psi) | |
| ASTM A709 Grade 50 & Grade 50W | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 50,000 psi | ( Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 27,000 psi) | |
| Superstructure Concrete: | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_c} | = 1,600 psi |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} | = 4,000 psi | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} | = 8 |
| Reinforcing Steel: | = 60,000 psi |
Physical Properties of Spans
- Composite Design - See Widening and Repair - Composite Design.
- Non-Composite Design - Use "Constant I" analysis.
When the neutral axis of a composite section falls in the concrete fange, the section shall be designed as Non-Composite (21" Wide Flange is the smallest beam generaly made conposite).
Deflection
Live Load Deflection: AASHTO - Article 10.6 Composite - Allowable Deflection L/1000 Non-Composite - Allowable Deflection l/800 Dead Load Deflection: Compute at 1/4 points for bridges with spans less than 75', at 1/10 points for spans 75' and over. Give percentage of deflection due to weight of structural steel.
Fatigue Stress
AASHTO - Article 10.3 Case I, Case II or Case III (as specified on design layout generally within the following limitations).
Case I: Bridges with the TRUCK traffic count of 2500 or more vehicles per day (one direction). Case II: Bridges with traffic count of 750 or more vehicles per day, and less than 2500 TRUCK traffic count (one direction) per day. Case III: Bridges with traffic count of less than 750 vehicles per day, except when Live Loading is H20 or greater. No Fatigue: Bridges with traffic of less than 75 vehicles per day.
Economic Comparison
When comparing cost of low-alloy steels (A-572, Gr.-50, and A-588) to the cost of A-36 steel, the low-alloy steels shall be figured a t 3 1/2 cents for A-572, Gr.-50 and 5 1/4 cents for A-588 per pound more than A-36 steel. Cost comparisions will be based on current average bid prices that may be obtained from the CHIEF DESIGNER, for comparable bridges.
No overstressed will be permitted in the design.
Total Capacity of Exterior Griders (Dead Load and Live Load)
In no case shall an exterior stringer have less carrying capacity than an interior stringer.
751.40.8.7.1.3 Flange Plate Lengths
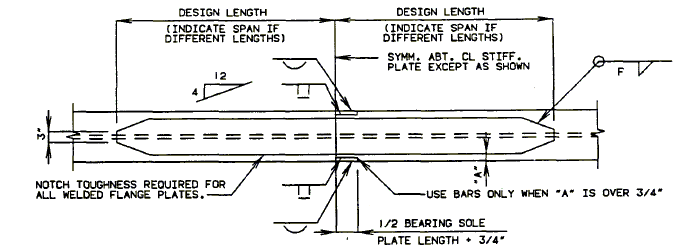
| |
| Top Flange | Bottom Flange |
|---|---|
| Details of Flange Plates at Intermediate Bents | |
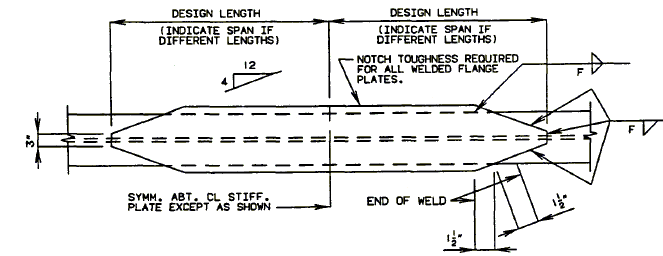
| |
| Details of Flange Plates at Intermediate Bents (Top and Bottom Flanges) | |
Allowable flange plate sizes are as shown with the section properties. Different plate sizes may be used on adjacent stringers.
Lengths to be shown on the bridge plans are those required as follows:
- Lengths each side of the bearing shall be the larger of:
- Theoretical End + Terminal Distance (***) or
- Point where the stress range (tension or reversal) in the beam flange is equal to or less than allowable fatigue stess range (Cat. E or E') or where the beam flange is in compression, whichever is smaller.
- Use Cat. E when the flange is less than or equal to 0.8 inch thick.
- Use Cat. E' when the flange is greater than 0.8 inch thick.
(***) Where the theoretical end = the point where the flange stress without cover plate less than or equal to base allowable stress. Terminal distance = 1 1/2 times nominal cover plate width.
The total length of the cover plate greater than or equal to (2D + 3'-0"). Where "D" = Depth of beam in feet.
When required lengths of plates vary by 12" or less on adjacent stringers or on each side of the centerline stiffener plate, use greater length for all such positions.
Plate lengths taken form the computer programs should be rouned up to at least the nearest 6".
751.40.8.8 Welded Plate Girders
751.40.8.8.1 Design
751.40.8.8.1.1 Design Assumptions & Procedures
Design Unit Stresses
| Reinforcement Concrete | ||
| Reinforcing Steel (Grade 60) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 24,000 psi, | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 60,000 psi |
| Class B2 Concrete (Superstructure) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_c} = 1,600 psi, | = 4,000 psi |
| Structural Steel: | ||
| Structural Carbon Steel (ASTM A709 Grade 36) | = 20,000 psi, | = 36,000 psi |
| Structural Steel (ASTM A709 Grade 50) | = 27,000 psi, | = 50,000 psi |
| Structural Steel (ASTM A709 Grade 50W) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 27,000 psi, | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 50,000 psi |
Design Procedure:
Moments and shears by "Variable I" analysis:
- use computer program.
Trial sections from "Preliminary analysis":
- Combination of web depth, flanges and length of plates used shall be the most economical section available with depths compatible with vertical clearance requirements. Web depths in 6" increments are preferred, however other increments may be used when required by the Design Layout. (See Structural Project Manager)
Flanges:
Minimum flange dimensions = 3/4" x 12" (*).
Increments:
- Thickness 1/8"
- Width 1"
Maximum flange dimensions:
- Reference AASHTO - Table 10.32.1A)
- maximum thickness = 4"
Note: It is preferred office practice to maintain the same flange thickness at as many locations as practical. This can be accomplished by varying the flange width.
(*) For shipping and erection purposes, minimum width of both compression and tension flanges shall not be less than L/85 where L is the shipping length of the girder. This limitation is for preventing out-of-plane distortion of the girder.
Webs:
Web dimensions:
- (Reference AASHTO - Articale 10.34 & 10. 48)
- ASTM A709 Grade 36 = 3/8" minimum thickness for curved girders and for continuous straight girders.
- ASTM A709 Grade 50W = 3/8" minimum thickness.
- AASHTO - Article 10.3 Case I, Case II or Case III.
- Case I
- Bridges with the truck traffic count of 2500 or more vehicles per day. (One direction)
- Case II
- Bridges with traffic count of 750 or more vehicles per day, and less than 2500 truck traffic count (One direction) per day.
- Case III
- Bridges with traffic count of less than 750 vehicles per day, except when live loading is H20 or greater.
- No Fatigue:
- Bridges with traffic count of less than 75 vehicles per day.
Total Capacity of Exterior Girders:
(Dead Load and Live Load)
- In no case shall an exterior girder have less carrying capacity than an interior girder.
Horizontal Curved Girders Design Procedures (*)
Curved plate girders are to be designed using load factor design criteria. The 1980 AASHTO Guide Specifications for Horizontally Curved Highway Bridges as revised by Interim Specifications for Bridges 1981, 1982, 1984, 1985 and 1986 is to be applied with the USS Highway Structure Design Handbook (\) V-Load method to be used as a working example.
The following procedure may be followed to determine the required cross-section for any system of curved girders with skews less than 46°.
1. Determine the primary moments by the same procedures as for a system of straight girders, using the developed lengths of the curved girders.
2. From primary moments, compute shear loads, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V}
, using the formula:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V = \frac{\sum M}{Coeff. * K}} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V}
= Shear loads. M = Primary moments. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K = \frac{RD}{d}} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, R}
= Radius of curvature (outside girder). Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, D} = Radial distance between inside and outside girders. Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, d} = Distance between diaphragms measured along axis of outside girder. |
The following coefficients may be applied to "Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K} " for the various multiple-girder systems with equal spacing between girders.
| SYSTEM | COEFFICIENT FRACTION |
COEFFICIENT DECIMAL |
| 2 girders | 1 | 1.00 |
| 3 girders | 1 | 1.00 |
| 4 girders | 10/9 | 1.11 |
| 5 girders | 5/4 | 1.25 |
| 6 girders | 7/5 | 1.40 |
| 7 girders | 14/9 | 1.56 |
| 8 girders | 12/7 | 1.72 |
| 9 girders | 15/8 | 1.88 |
| 10 girders | 165/81 | 2.04 |
3. Compute Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V-Load} moments
- Reference: USS "Highway Structures Design Handbook" 1965 Edition. (Updated 1986 Volume II Section 6) developed by Richardson, Gordon and Associates in cooperation with Dr. John Scalzi is to be used as a working example.
4. Compute lateral bending moments using the approximate formula:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_L = \frac{Hd}{10} = \frac{Md^2}{10Rh}} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, ML } = Lateral bending moment | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, H } = The Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, H} values are approximately equal to the reactions at the supports. | ||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, h } = Depth of girder between centers of gravity of flanges. | ||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M } = Primary moment + Secondary moment. |
5. Determine cross-section required to provide for vertical and lateral forces computed under Items 1 to 4 inclusive. As with any statically indeterminate system it is necessary to make an initial assumption of the required cross-sections and to repeat the calculations one or more times to obtain reasonable agreement between the assumed and required sections.
6. The non-compact section requirement that Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y > (f_b + f_w)}
is to be applied to all sections with the tension flange and the compression flange as to ensure conservative design.
In computing , use to be actual diaphragm spacing for compression and tension stresses.
The value of is to be selected as plus or minus in the equations for to give the worst possible case.
Design and Detail Guides
1. Economic Arrangement of Spans and Depth-to-Spans Ratios
Where there is flexibility in span arrangement, the same guides that apply to economic arrangement of straight girders are equally applicable to curved girders. Similarly the rules used to establish depth-to-span ratios for straight girders usually will apply to curved girders.
2. Spacing of Girders
Spacing depends on the arrangements of diaphragms and bracing. In general, however, it will be found that the most economical arrangement for straight girders will accord very well with the best arrangement for a system of curved girders. The effect of curvature increases in proportion to the square of the span length and decreases in proportion to the radius of curvature and the spacing of girders.
3. Arrangement and Spacing of Diaphragms
The diaphragms shall be placed radially, with a maximum spacing of 15'-0". In order to minimize lateral bending of the girder flanges, the flanges should be as wide as practical.
Sway frame bracing is selected for curved girder system, by same methods as for straight girders.
4. Effect of Lateral Bracing
made in a similar manner as for straight bridges. If lateral Provision for lateral loading on curved girders may be bracing is used in a system of curved girders, the forces resulting from the radial components of flange stress may be carried partially or entirely by the bracing system; when both diaphragms and lateral bracing are used, radial reaction components may be divided between the two systems.
5. Approximate Estimate of Curvature
The following formula may be used in making preliminary approximations of the effect of curvature:
| Note: For "r" refer to paragraph No. 7 | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, r = \frac{(R_2)^2}{(R_2)^2} \times \bigg( \frac {Inside \ girder \ loading)}{Outside \ girder \ loading} \bigg)} | (*) |
| (*) | May be omiteed if supports are on radial lines. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P } | % increase in positive moment due to effect of curvature. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, R_2} | Radius of inside girder. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, R_1} | Radius of outside girder. |
| Distance between points of contraflexure in any pisitive moment area. | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, D } | Spacing between inside and outside girders. |
In the above form, the formula applies to a two-girder system, but it may be modified by reference to the table of coefficients for multiple-girder systems shown on page 1.1-5 of this section.
The formula applies particularly to positive moment, but for preliminary approximation it may be assumed that the curvature effect on negative moments will be about the same.
6. Design of Diaphragms and Connections
Where the degree of curvature is equal to or under 1° - 30' and when spans are equal to or under 75'-0" in length, the diaphragm and connections shall be the same as for Bridges with straight girders. Where the degree of curvature is over 1°- 30' to 3° or with a span length of more than 75'-0", the diaphragm must be attached to the tension flange. Where the degree of curvature is over 3°, a special design will be required for connection of intermediate diaphragms to flanges.
The maximum allowable diaphragm spacing is 15'-0", regardless of the amount of curvature, or span lengths.
The following applies to those bridges where the special design is to be considered:
Since diaphragm moments due to effect of curvature are a function of the radial component of flange stress, they are directly proportional to the vertical bending moment in the girders.
For exterior girders the moment in the diaphragm equals , in which = vertical bending moment in girder for any particular condition of loading; = diaphragm spacing; Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, R}
= Radius of curvature of girder.
For negative moment over the support, the value used in this equation should be the average moment between a point at the support and a point at the first adjacent diaphragm.
Diaphragm connections may be made directly to the flanges of the girders or through stiffeners, provided details are arranged to adequately transfer radial components of flange stress into the diaphragms.
7. Supports positioned other than on radial lines.
If field conditions permit, the most orderly arrangement for curved girders will be attained by placing the supports on radial lines.
It may be necessary to treat each line of girders independently, first finding the direct loading moments and then correcting for curvature by applying the separate .
8. Transverse stiffeners
The maximum transverse stiffener spacing for curved plate girders is Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, D}
, the web height.
Transverse stiffeners should be placed along the girder length only as far as required by design.
The maximum spacing of the first transverse stiffener at the simple support end of a curved plate girder is Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, D/2} .
Reference:
- AASHTO - Article 10.5
- Limit radius of heat curved girders according to AASHTO Article 10.15.
Where the distance between field splices of curved girders exceeds that given by the following formula, a special note shall be placed on the plans.
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \sqrt{\frac{0.667 \ x \ f_s \ x \ SM}{W}}} | (*) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L} = | Allowable distance between field splices, in feet. | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = | Allowable fs of flange steel, in psi. e.g. use 20,000 psi for Grade 36 steel. | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W} = | Weight of girder (flanges and web), in pounds per foot. | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, SM} = | Section Modulus of girder about x-x axis as shown, in inches cubed. |
Note:
If flanges are of different sizes, use smaller Section Modulus.
See Structural Project Manager for allowable overstress.
(*) Derivation
- Positive moment at centerline, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Mom. = \frac{WL^2}{8} \times 12}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fs = \frac{Mom.}{SM}}
- Substitute mom. in fs equation.
- solve for L
Design Example
ASTM A709 Grade 36 Steel
| |||||||||||
 |
| ||||||||||
| SECTION A-A | |||||||||||
| Weight per Foot of Girder |
| PL 12" x 3/4" = 30.6 lbs./ft. |
| PL 70" x 3/8" = 89.3 lbs./ft. |
| PL 13" x 3/4" = 33.2 lbs./ft. |
| Total = 153.1 lbs./ft. |
From Formula: (Use 57.5')
Special Note:
Heat curving of girders (Identify) (*) will not be allowed shile in the horizontal position.
(*)Complete underlined portion as required.
Maximum Plate Lengths:
- 80 feet. See Structural Project Manager for use of longer lengths up to 85' for ASTM A709 Grade 50 or ASTM A709 Grade 50W and 100' for ASTM A709 Grade 36.
Minimum Plate Lengths:
- 10 feet. Shop flange splices should be eliminated and extra plate material used when :economy indicates and span lengths permit.
Preliminary Analysis:
- (1) Compute moments from influence lines on basis of "Constant I" analysis and apply the following percentage increase or decrease to non-composite dead load moments.
References may be used in lieu of the above.
| = 1.2 to 1.5 | = 1.2 to 1.5 | ||||||||||||||
|
|
(2) Determine trial sections and plot a rough moment curve to determine location of flange plate cutoffs, if any.
(3) Complete analysis by using computer programs to obtain actual moments and stresses.
Design Stress investigation for Positive Moment Area of Plate Girder Structure
The design stresses are to be checked at the top of flange (steel) and the top of concrete slab in the composible area of Plate Girder Structures to insure that they are within the allowable stresses.
SECTION A-A
Structure Length
Typical Continuous Steel Structures- Integral End Bents:
Estimated Girder Depth
Based on Three Spans With Ratio N = 1.3±
Continuous Plate Girders HS20 Loading Load Factor
(ASTM A709 Grade 50 or ASTM A709 Grade 50W)
| Initial Estimate (Feet) |
Girder Depths (*) (Inches) |
Structure Depth (**) (Feet) |
| 85 to 104 | 42 | 4.50 |
| 105 to 124 | 48 | 5.00 |
| 125 to 134 | 54 | 5.50 |
| 135 to 144 | 60 | 6.00 |
| 145 to 159 | 66 | 6.50 |
| 160 to 174 | 72 | 7.00 |
| 175 to 184 | 78 | 7.50 |
| 185 to 194 | 84 | 8.00 |
| 195 to 204 | 90 | 8.50 |
Trial steel plate girder depths use program BR109 to check designs and deflections. Web depths may be adjusted by two inch increments.
(*) Bethlehem steel economic study (N = 1.3±). Bethlehem steel provided an economic study of multiple steel girder depths. The study indicated that cheaper designs are obtained by reducing the plate girder depths and reducing the number of stiffeners. The recommended initial estimates above are based on these designs.
(**) Structure depth includes slab and haunch.
A general rule of thumb is to determine the minimum web thickness without stiffeners; then, use a web thickness of one-sixteenth inch less. Match MoDOT requirements for web increments of one-sixteenth inch only.
If two-span structures are used, a deeper web is required. A good estimate is to use six inches additional depth than the above tables for two-span structures.
751.40.8.8.2 Details
751.40.8.8.2.1 Field Flange Splice – Bolted
General
Splices shall be designed using the Service Load Design Method and in accordance with AASHTO Articles 10.18,10.24 and 10.32 except as noted.
Splices shall be designed to develop 100% of the flange strength by the flange splice plate strength. When the flange section or steel grade changes at a splice, the smaller flange strength shall be used to design the splice. Splice plates shall then match the lower grade used in the flanges.
Minimum Yield Strength Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (Fy)}
and Minimum Tensile Strength Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (Fu)}
| ASTM A709 Grade 36 | = 36 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} = 58 ksi |
| ASTM A709 Grade 50 | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 50 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} = 65 ksi |
| ASTM A709 Grade 50W | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 50 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} = 70 ksi |
Allowable Steel Stresses Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_t)}
Allowable stresses are determined by AASHTO Table 10.32.1A.
| Allowable tensile stress |
| ASTM A709 | Grade 36 | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_t} = 20 ksi |
| ASTM A709 | Grade 50 | = 27 ksi |
| ASTM A709 | Grade 50W | = 27 ksi |
Allowable Bolt Stresses
Splices shall be designed as slip critical connections with Class B surface preparation and oversized holes. Although standard holes are used in the fabrication of flange splices, designing the splices for oversize holes allows for some fabrication and erection tolerances. All splice bolts shall be 7/8" diameter ASTM A325 high strength bolts.
AASHTO Table 10.32.3C specifies = 19 ksi for a class B slip-critical connection. Tables shown in this manual are based on 19 ksi that should also be used to design splices not listed in the table.
Although slip-critical connections are theoretically not subject to shear and bearing, they must be capable of resisting these stresses in the event of an overload that causes slip to occur. The allowable shear stress per bolt Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (Fv)}
for bearing is 19 ksi with the threads included and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 1.25 \times 19 = 23.75}
ksi for threads not included.
Flange Strength
The flange strength shall be determined by multiplying the allowable stress of the flange by the area of the flange. The area of the flange shall be taken as the gross area of the flange, except that if more than 15 percent of each flange area is removed, that amount removed in excess of 15 percent shall be deducted from the gross area. Bolt holes are considered to be 1" diameter for the purpose of determining flange area.
Splice Plate Strength
The splice plate strength shall be determined by multiplying the allowable stress of the splice plates by the area of the splice plates. The area of the splice plates shall be taken as the gross area of the splice plates, except that if more than 15 percent of the splice plate area is removed, that amount in excess of 15 percent shall be deducted from the gross area.
Two Row Splices
Splices with two rows of bolts are used with flanges 12 to 13 inches wide. The inner and outer plates may either be the same length or the inner plate may be shorter. This is the case if the end bolts in the splice are only needed to be in single shear. All other bolts will be in double shear. (See Figure 3.42.2.2-1)
Figure 3.42.2.2-1
Four Row Splices
When the width of the flange being spliced is 14 inches or greater, four longitudinal rows of bolts are used. Three variations of the end bolts positioning may be used. In each of these variations, the last two bolts shall be located in the outer rows closest to the edge of the splice plate.
Figure 3.42.2.2-2
Flange Width Transitions
When the width of the flanges being spliced differs by more than 2", the larger flange shall be beveled as shown in Figure 3.42.2.2-3
Figure 3.42.2.2-3
Weight of Splice
When calculating the weight of splice, the following simplified weights shall be used.
Weight of High-Strength bolts (diameter 7/8") = 0.95 lbs/bolt
Unit weight of Structural Steel = 490 lbs/ft3
751.40.8.8.2.2 Field Web Splice – Bolted
General
Splices shall be designed using the Service Load Design Method and in accordance with AASHTO Articles 10.18,10.24 and 10.32 except as noted.
The web splice consists of 2-Plates:
- Thickness = 5/16" minimum.
- Width = 12-1/2" (18-1/2" if 3 rows of bolts are required).
When the web section or steel grade changes at a splice, the smaller web strength should be used to design the splice.
Minimum Yield Strength Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_y)}
and Minimum Tensile Strength Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_u)}
| ASTM A709 Grade 36 | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 36 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} = 58 ksi |
| ASTM A709 Grade 50 | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 50 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} = 65 ksi |
| ASTM A709 Grade 50W | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_y} = 50 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_u} = 70 ksi |
Allowable Steel Stresses Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_b, F_w)}
Allowable stresses are determined by AASHTO Table 10.32.1A.
| Allowable bending stress | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_b = 0.55 \times F_y} |
| Allowable shear stress | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_v = 0.33 \times F_y} |
| ASTM A709 Grade 36 | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_b} = 20 ksi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_v} = 12 ksi |
| ASTM A709 Grade 50 | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_b} = 27 ksi | = 17 ksi |
| ASTM A709 Grade 50W | = 27 ksi | = 17 ksi |
Allowable Bolt Stresses
Although standard holes are used in the fabrication of web splices, designing the splices for oversize holes allows for some fabrication and erection tolerances. Web splices required to resist shear between their connected parts are designated as slip-critical connections. Shear connections subjected to stress reversal, or where slippage would be undesirable, shall be slip-critical connections. Potential slip of joints should be investigated at intermediate load stages especially those joints located in composite regions. The resultant force shall be less than the allowable bolt shear force. All splice bolts shall be A325 7/8" diameter High Strength Bolts.
= 19 ksi
Bolt Arrangement
The minimum distance from the center of any fastener in a standard hole to a sheared or thermally cut edge shall be 1-1/2 inches for 7/8" diameter fasteners. The minimum distance between centers of fasteners in standard holes shall be three times the diameter of the fastener, but shall not be less than 3 inches for 7/8" diameter fasteners.
Splice Plate Strength
The strength of the splice plates shall be determined by multiplying the allowable stress of the splice plates by the net area of all splice plates. The splice plates net area shall be taken as the gross area of the splice plates minus the bolt holes. Bolt holes are considered to be 1 inch diameter for the purpose of determining splice plate net area. Web splices are designed to develop 75% of net section of the web.
Web Strength
The strength of the web should be determined from the allowable web stress at the "top of web" to account for hybrid sections. Otherwise, the allowable web stress is based on a linear distribution of stress from outside face of flange to "top of web".
Weight of Splice
When calculating the weight of splice, the following simplified weights shall be used.
Weight of High-Strength bolts (diameter 7/8") = 0.95 lbs/bolt
Unit weight of Structural Steel = 490 lbs/ft3
751.40.8.9 Continuous Concrete Slab Bridges
751.40.8.9.1 Slabs
751.40.8.9.1.1 Design Assumptions
| Stresses - | FC | = | 1600 psi | N | = | 8 | (Slab, Integral Column) |
| FC | = | 1200 psi | N | = | 10 | (Open Bent, Footing) | |
| FY | = | 60,000 psi reinforcing steel | |||||
Use "Variable I" analysis for all structures except solid slabs without drop panels.
Use "Constant I" analysis for solid slabs without drop panels.
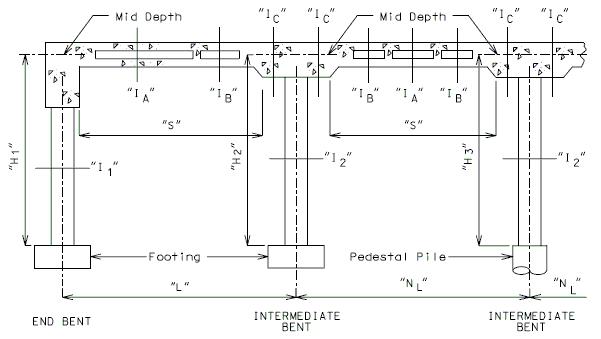
| "L" | = | Design Span |
| "H" | = | Design Height |
| "I" | = | Gross moment of inertia of the full cross-section (Slab minus voids - integral wearing surface not included) ("I1", "IA", etc. suggested I's to be considered.) |
| "S" | = | The effective span length for the use in determining minimum slab thickness under load factor design (AASHTO 8.9). |
Use the same column diameter and spacing for all Intermediate Bents.
Use the same slab thickness for all spans.
| DEGREE OF RESTRAINT - LONGITUDINAL | ||||
| Column Type | Footing Type | Top Column | Bottom Column | |
| INT. BENTS | Integral Column | Spread or Pile | Integral | (**) |
| Integral Column | Pedestal Pile | Integral | (**) | |
| END BENTS | Pinned Column | any | Pinned | (**) |
| Integral Pile | (*) Pinned | |||
| Open Bent with Column | any | Simple | ||
| INT. BENTS | Open Bent with Pile | Simple | ||
| (*) | See "Integral Piles" this Manual Section. | |
| (**) | Use "Pinned" for Seismic Performance Category A and "Fixed for Seismic Performance Categories B, C & D. (See Structural Project Manager or Liaison) |
751.40.8.9.1.2 Slab Design and Drop Panel
The Slab Depth is based on the following limitations:
| 1. | Vertical Clearance Requirements: see the Design Layout. | ||||
| 2. | Allowable Depths: | ||||
| A. | Positive Moments - | ||||
| see table of "Available Slab Depths", of this Section. | |||||
| B. | Slab Depth controlled by the minimum thickness formula - | ||||
| (Integral wearing surface is included with the total depth provided.) | |||||
| Continuous Spans - AASHTO 8.9 = (S + 10)/30 | |||||
| "S" | may be used as the clear distance between drop panels. | ||||
| Bridges may have two adjacent spans averaged if S2/S1 < 1.5 | |||||
| Simple Spans - AASHTO 8.9 = 1.2 (S + 10)/30 | |||||
| C. | Negative Moments - | ||||
| DROP PANEL DEPTHS | ||
| MIN. | MAX. | |
| Bents in median of dual roadway | 0" or 3" | 13" |
| Other Bents | 0" or 3" | 9" |
| INCREMENTS OF 1" | ||
| APPROXIMATE DROP PANEL WIDTH (FEET) (PARALLEL TO THE CENTERLINE OF ROADWAY) | |||||||
| Bents | Drop Panel Depth | ||||||
| 4" | 6" | 7" | 8" | 9" | 12" | ||
| 3 Span Bridge | 2 & 3 | 6' | 6' | 10' | 8' | 6' | |
| 4 Span Bridge | 2 & 4 | 6' | 6' | 10' | 8' | 6' | |
| 3 | 8' | 10' | 12' | 12' | 12' | 12' | |
| THESE WIDTHS ARE SUGGESTED ONLY AS TRIAL DIMENSIONS FOR DESIGN AND ARE NOT TO BE USED AS LIMITS FOR THE FINAL DESIGN. | |||||||
| 3. | Reinforcing Steel: | ||||
| A. | Positive Moments = Maximum #11 @ 5" cts. | ||||
| B. | Negative Moments = Maximum #11 @ 5" cts., except #14s @ 6" cts., may be used for long spans. | ||||
| 4. | Live Load Deflection - AASHTO 10.6 | ||||
| The deflection due to service live load plus impact shall not exceed 1/800 of the span, except on bridges in urban areas used in part by pedestrains whereon the ratio preferably shall not exceed 1/1000. | |||||
751.40.8.9.1.3 Slab Longitudinal Sections
HOLLOW SLABS

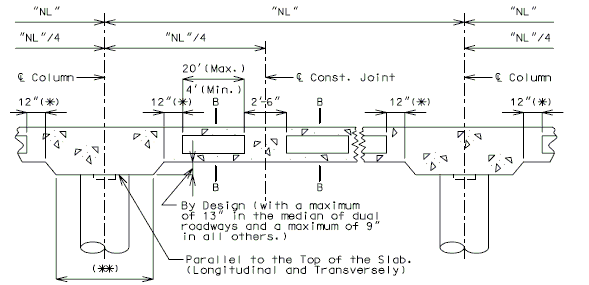
|
(*)Increase to maintain 6" minimum on skews (see detail) |
| (**) By Design (6" increments measured normal to the centerline of bent) (The minimum is equal to the column diameter + 2'-6") | |
| Note: All longitudinal dimensions shown are horizontal (Bridges on grades and vertical curves, included). For Sections A-A and B-B see this Manual Section. |
SOLID SLABS
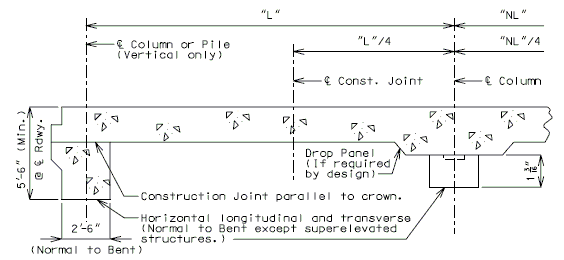
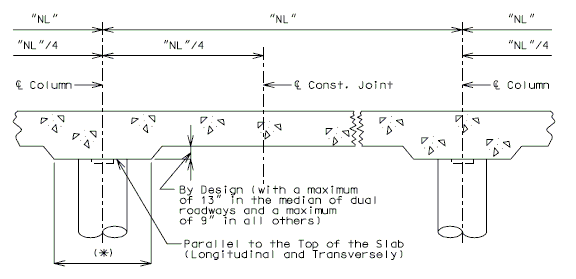
(*) By Design (6" increments measured normal to the centerline of Bent) (The minimum is equal to the column diameter + 2'-6")
Note:
All longitudinal dimensions shown are horizontal (Bridges on grades and vertical curves, included).
751.40.8.9.1.4 Slab Cross Section and Section Properties
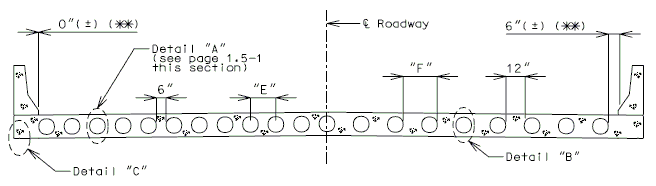
| HALF SECTION A-A CENTER OF SPAN |
HALF SECTION B-B NEAR INTERMEDIATE BENT |
|
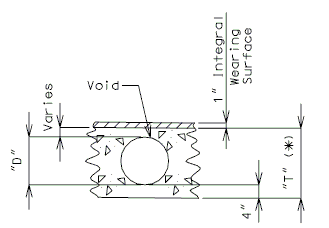
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| PART SECTION THRU VOID | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| DETAIL "C" | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Notes: | |
| (*) | Increase the Dimension "T" by 1/2" for #14 bars placed in the top or bottom of the slab. |
| Increase the Dimension "T" by 1" for #14 bars placed in the top and bottom of the slab. | |
| ("T" and "D" are based on 3" clearance which includes the integral wearing surface to the top of the longitudinal bar.) | |
| (**) | For Roadways with slab drains, use 10" minimum. For Roadways that require additional reinforcement for resisting moment of the edge beam 20" minimum, refer to this section. |
| (***) | Preferred minimum (Consult the Structural Project Manager prior to the use of a thinner slab.) |
Voided Slab Spans
| Void Dia. (in.) |
Area (sq.ft.) |
Area (sq.in.) |
Moment of Inertia (ft.4) |
Moment of Inertia (in.4) |
Weight (lb./ft.) |
| 8.00 | 0.3490 | 50.2656 | 0.0096 | 201.0624 | 52.35 |
| 9.00 | 0.4417 | 63.6174 | 0.0155 | 322.0630 | 66.26 |
| 10.00 | 0.5454 | 78.5400 | 0.0236 | 490.8750 | 81.81 |
| 12.00 | 0.7854 | 113.0976 | 0.0490 | 1017.8784 | 117.81 |
| 14.00 | 1.0690 | 153.9384 | 0.0909 | 1885.7454 | 160.35 |
| 15.70 | 1.3443 | 193.5932 | 0.1438 | 2982.4242 | 201.66 |
| 16.70 | 1.5211 | 219.0402 | 0.1841 | 3818.0075 | 228.17 |
| 18.70 | 1.9072 | 274.6465 | 0.2894 | 6002.5789 | 286.09 |
| 20.85 | 2.3710 | 341.4310 | 0.4473 | 9276.7336 | 355.65 |
751.40.8.9.1.5 Slab Reinforcement
HOLLOW SLABS
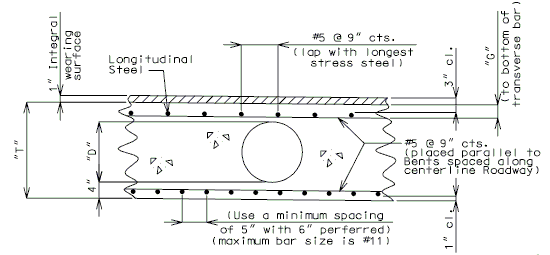
(POSITIVE MOMENT)
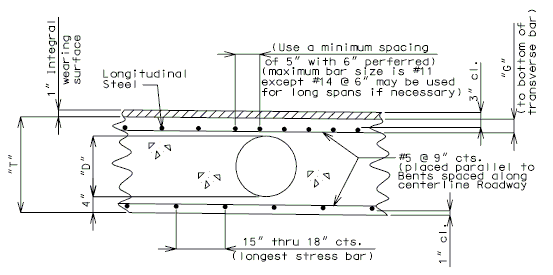
(NEGATIVE MOMENT)
| Longitudinal Reinforcement (Largest Bar) |
"G" |
| #8 | 3-5/8" |
| #9 | 3-3/4" |
| #10 | 3-7/8" |
| #11 | 4" |
| #14 | 4-3/8" |
Moment Curves
| 1. | Determine reinforcing steel from the sum of the dead loads and the live loads + impact (working stress design) or design in accordance with AASHTO Article 8.16 and 8.9 (load factor design). |
| 2. | Determine the cut-off points for the stress bars in sets of 2 or 3. Maximum length = 60'-0", see AASHTO Article 8.24 for extension of reinforcement. |
| 3. | Determine the drop panel width: |
| Minimum width = Column diameter plus 2~6". Maximum width = (Parallel to the centerline of roadway) as determined by deign). | |
| In general, the width of the drop panel normal to centerline bent should be adjusted to 6" increments. |
SOLID SLABS (BOTTOM)
Use AASHTO 3.24.10 Distribution Reinforcement shall be a percentage of positive moment reinforcement (% = 100/√S, with a maximum of 50%).
EDGE BEAM
| Positive Moment: | ||
| The bridge curb is not to be used in determining the resisting moment of the edge beam. | ||
| Dead Load: | Use the same distribution as for the slab design. Use for simple spans 0.1 PS. | |
| Live Load + I: AASHTO Article 3.24.8 | ||
| Use for negative moment on continuous spans 0.1 PS. Use for positive moment on continuous spans 0.08 PS. | ||
| Where | P = Wheel load in pounds, see this Section. | |
| S = Span in feet | ||
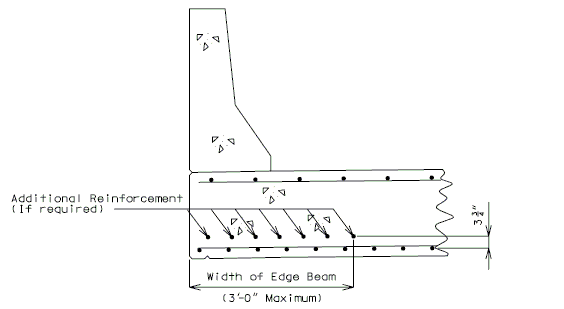
751.40.8.9.1.6 Shear
Shear Loads
The shear in the Hollow Slab should be computed for all loadings H20 and over.
Distribution of Loads
Use the same distribution for the dead and live load as was used for the moment.
Unit Shear Stress
| Load Factor: | |||
| Shear Stress | = | ||
| Working Stress: | |||
| Shear Stress | = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, v = \frac{v}{(Bd - Area~of~voids)}} | |
| Where "d" = effective depth, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi} = 0.85 for shear | |||
Allowable Shear Stress
| Load Factor: | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Vc = 2.0\sqrt{f'c}} | |
| Where Vc = shear strength provided by concrete | |
| Working Stress: | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Vc = 0.95\sqrt{f'c}} | |
| Where Vc = Allowable shear stress carried by concrete | |
If shear stress (load) exceeds the allowable shear use one or more of the following solutions.
- Eliminate some voids and replace remainder.
- Shorten alternate voids
- Use shear reinforcing in the critical zone.
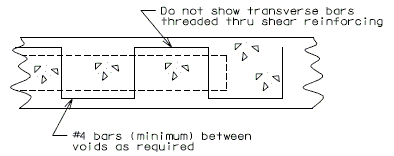
Note:
Consider a voided slab the same as a regular slab as it pertains to the minimum stirrups (AASHTO - Article 8.19).
i.e. The minimum stirrups are not required if the shear stress is less than allowable.
751.40.8.9.1.7 Camber Deflection
Ultimate Deflection:
Compute the "ultimate deflection" at 0.2 points of the spans for the dead loads without the 35# future wearing surface.
Ultimate deflection (long term) = elastic deflection x 3
| Ec (Elastic Modulus) = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 4 \times 10^6} psi (districts 1 and 4) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 6 \times 10^6} psi (remainder of districts) |
The modulus of elasticity for the use in a continuous structure analysis computer program should be determined as follows:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Delta_{ULT}} | = | |
| = | ||
| = | ||
| Where: | ||
| = | deflection. | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Delta_{ULT}} | = | Ultimate deflection |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Delta_{ELASTIC}} | = | Elastic deflection |
Example No. 1
(Assume bridge is in District 8)
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c} | = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 6 \times 10^6 psi} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Delta_{ULT}} | = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Coeff. / (6/3) = Coeff./2} |
Therefore, use 2 \times 106 psi for modulus of elasticity in the structure analysis computer program to get ultimate deflection. (*)
Example No. 2
(Assume bridge is in District 1)
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c} | = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 4 \times 10^6 psi} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Delta_{ULT}} | = |
Therefore, use Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 1.333 \times 10^6} psi for modulus of elasticity in the structure analysis computer program to get ultimate deflection. (*)
(*) Gives long term deflection as output.
751.40.8.9.1.8 Slab Construction Joint Details

(FOR SLAB DEPTHS 17" OR MORE)

(FOR SLAB DEPTHS 16½" OR LESS)
751.40.8.9.2 End Bents
751.40.8.9.2.1 Pile Cap Bents
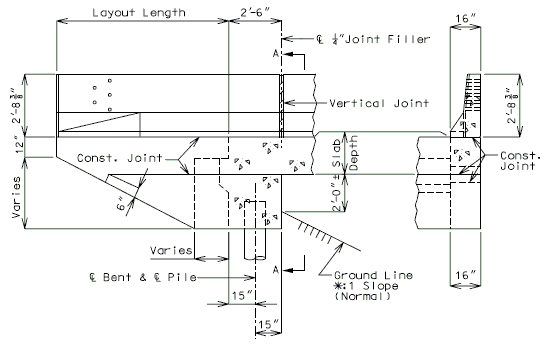
| SECTION THRU WING | SECTION A-A |
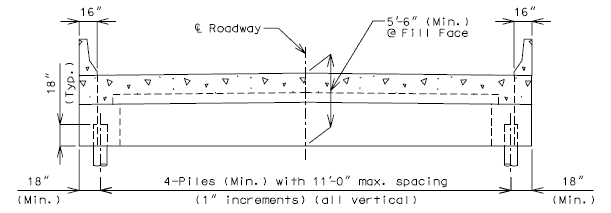

| (1) Wing brace details. | 
|
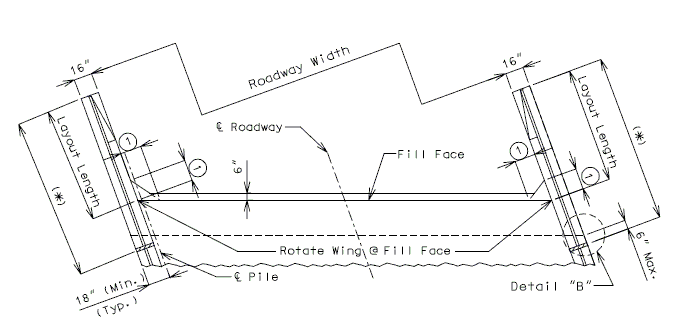
751.40.8.9.2.2 Integral Column Bents
SEISMIC PERFORMANCE CATEGORY A
(PINNED COLUMN AT TOP AND BOTTOM)
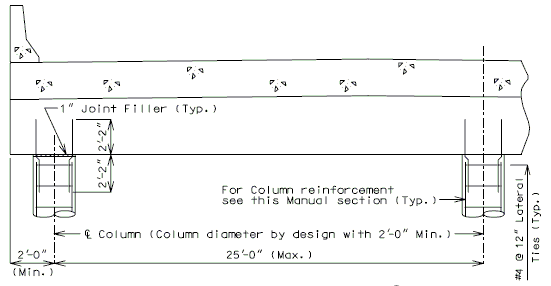
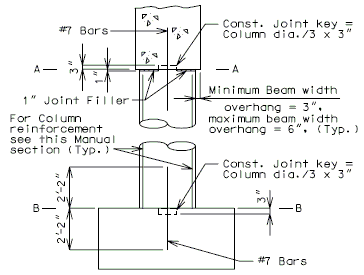 |
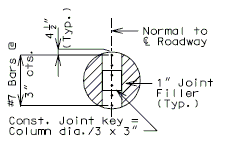
|
| SECTION A-A | |
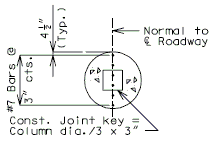
| |
| PINNED COLUMN | SECTION B-B |
Note: If the columns at an end bent have excessive moments due to shortness of the Column or length of the span, they should be detailed as "pinned" and designed for vertical reactions only.
SEISMIC PERFORMANCE CATEGORIES B, C & D
(PINNED COLUMN AT TOP, FIXED COLUMN AT BOTTOM)
For pinned column conditions at the top, see the above details.
For fixed column conditions at the bottom and column reinforcement details.
Note: For details not shown, see integral pile cap details.
751.40.8.9.2.3 Reinforcement - Pile Cap Bents

- SECTION THRU END BENT
(Slab depth less than 16")
- SECTION THRU END BENT

- SECTION THRU END BENT
(Slab depth 16" or more)
- SECTION THRU END BENT
(**) Development length for top bar minimum.
751.40.8.9.2.4 Reinforcement - Wing
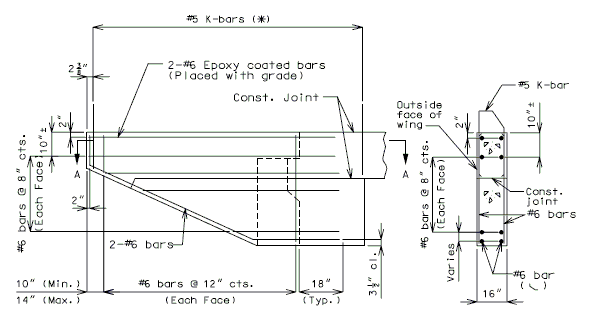
| ELEVATION OF WING | PART SECTION THRU WING |
| (*) Clip K bars as required to maintain minimum clearance at bottom of wing. | |

| SECTION A-A (K-bars not shown for clarity) |
PART SECTION THRU END OF WING |
Note: See _____ for barrier curb details and spacing of K-bars.
751.40.8.9.2.5 Design Assumptions for Integral Piles
Seismic Performance Category A
Piles may be considered as "pinned" (for superstructure) at the pile cap and designed for vertical loads only unless they fall under the following general conditions in which case they should be checked for the loadings as specified for columns.
| 1. | Height from centerline of slab to "pin" is less than 15'. |
| The location of the pinned joint is arbitratily taken as about 1/3 of the length of long piles or at a point about 10' below the natural ground line. | |
| 2. | Piles having a large gross moment of inertia (cast-in-place concrete) gross I of steel BP = I x n. |
| 3. | The number of piles used on a fairly long structure is small. |
Seismic Performance Categories B, C & D
Piles shall be checked for combined axial and bending stresses for seismic loading conditions. For AASHTO group loads I thru VI as applicable, follow criteria noted above for seismic performance category A.
751.40.8.9.3 Intermediate Bents
751.40.8.9.3.1 Integral Bents
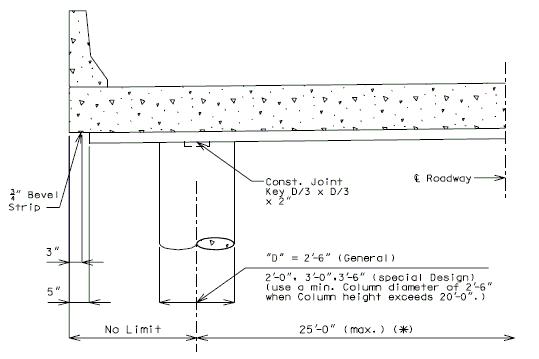
(*) 25'-0" is the max. column spacing allowed. However, the footing pressure may be the controlling factor for the column spacing. It is suggested that a rough check be made of the footing pressure before the spacing is definitely established.
In congested areas, when it is desired to keep the number of columns to a min., larger column spacings may be desirable. (consult the Structural Project Manager).
In general, use two 2'-6" columns for Roadways thru 44'-0" and additional 2'-6" columns for wider Roadways.
SEISMIC PERFORMANCE CATEGORY A


751.40.8.9.3.2 Integral Column Bent with Drop Panel
 |
ATTENTION DETAILER: When detailing Int. Bents on SPS the Section thru drop panel shall be drawn to appropriate grade. |
| PART SECTION |

| PART SECTION A-A (FLAT) |
PART SECTION A-A (GRADE OR V.C.) D = Diameter of Column |

| PART PLAN - SQUARE | PART PLAN - SKEWED |

|
 |
For Reference Only | ||||||||
|
(*) Based on 3" clearance and #6 stirrups, (includes Integral W.S.) to top longitudinal bar.
(1) Standard 90° Hook.
(2) Const. joint key D/3 x D/3 x 2", D = Diameter of Column
751.40.8.9.3.3 Integral Pile Cap Bents with Drop Panel

| PART SECTION | PART SECTION A-A (FLAT) |
| Bottom or drop panel to be parallel to top of slab both transversely and longitudinally. | 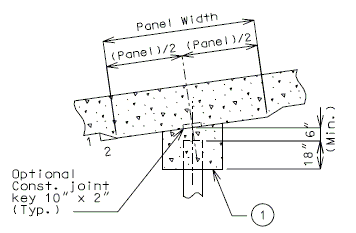
|
| (1)Horizontal except for superelevated structures. | |
| (2) Use 3" Min. clip on beam for skews above 35°. | |
| PART SECTION A-A (GRADE OR V.C.) |

| PART PLAN - SQUARE | PART PLAN - SKEWED |
REINFORCEMENT


(1) Use 5 1/4" for computing length of stirrup bar. Do not detail on plans.
(2) Standard 90° hook.
(3) Optional Const. Joint Key 10" x 2"
751.40.8.9.3.4 Integral Pile Cap Bents without Drop Panel
REINFORCEMENT


(1) Use 5 1/4" for computing length of stirrup bar. Do not detail on plans.
(2) Horizontal except for superelevated structures.
(3) Standard 90° hook.
751.40.8.9.3.5 Pile Footing Design and Details
(1) GENERAL
Number, size and spacing of piling shall be determined by computing the pile loads and applying the proper allowable overstresses.
Cases of Loading (AASHTO Article 3.22)
Group I maximum vertical loads.
Group IV temperature and shrinkage moments with applicable vertical loads.
1983 AASHTO guide specifications for seismic design of highway bridges. (See chapter 4 for earthquake loads combined with applicable vertical loads.) (*) (See Structural Project Manager or Liaison)
Internal stresses including the position of the shear line shall then be computed.
Long narrow footings are not desirable and care should be taken to avoid the use of an extremely long footing 6~0" wide when a shorter footing 8'-3" or 9'-0" wide could be used.
When using the load factor design method for footings, design the number of piles needed based on the working stress design method.
ASSUMPTIONS (Bents with 2 or more columns)
SEISMIC PERFORMANCE CATEGORY A
- Dead and live load moments will be 25% of the moments used for slab and top of Column design.
- Temperature moments shall be 50% of the moment at top of Column.
- Column reinforcement to be same as that required at top of Column. Footing dowel's to be #5 bars, same number as column bars.
- Footings to be proportioned for conditions as specified. Do not use ratio of bent height as specified for Intermediate Bents for longitudinal footings dimensions.
SEISMIC PERFORMANCE CATEGORIES B, C & D
- For Seismic Performance categories B, C & D, the connection between the bottom of Column and the footing is a fixed connection.
- Footing design is based on (Seismic Design of Beam-Column Joint).
(*) The design of all bridges in seismic performance B, C & D are to be designed by earthquake criteria in accordance with this bridge manual.
(2) PILE LOADS
- P = N/n ± M/S
- P = Pile Loads
- N = Vertical Loads
- n = number of piles
- M = overturning moment
- if minimum eccentricity controls the moment in both directions, it is necessary to use the moment in one direction (direction with less section modulus of Pile group) only for the footing check.
- S = Section Modulus of pile group
AASHTO GROUP I AND IV LOADS
Maximum P = Pile Capacity Minimum P = 0
Tension on a pile will not be allowed for any combination of forces.
Overstress reduction will not be used for loading minimums.
EARTHQUAKE LOADS
POINT BEARING PILES
(**) Maximum P = Pile capacity x 2
- (I.E. for HP 10 x 42 piles, maximum P = 56 x 2 = 112 tons/pile).
Minimum P = Use allowable uplift force specified for piles in Bridge Manual 3.71 under seal course design.
(**) Two (2) is our normal factor of safety. Under earthquake loadings only the point bearing pile and rock capacities are their ultimate capacities.
FRICTION PILES
Maximum P = Pile capacity
(3) INTERNAL STRESSES
- A) Shear Line
- B) Bending
- C) Distribution of Reinforcement
- D) Shear
751.40.8.9.3.6 Pedestal Pile
GENERAL
No concrete bell shall be used without approval of Structural Project Manager or Liaison.
SEISMIC PERFORMANCE CATEGORY A
- Assume column to be "pinned" for belled footing sitting on rock. All loads will be axial.
- Assume column to be fixed for pedestal pile embedded in rock.
- All earth loads within the diameter of belled footing, or pedestal pile if there is no bell, above ground line shall be included in footing design.

SEISMIC PERFORMANCE CATEGORY B, C & D
See (Seismic Design).
DETAILS
SEISMIC PERFORMANCE CATEGORY A

| Diameter of Shaft |
Minimum Bell Diameter |
Maximum Bell Diameter |
Minimum (*) Reinf. |
Cubic Yards Concrete per ft. |
| 2'-0" | 2'-4" | 6'-0" | 8-#7 | 0.1164 |
| 2'-6" | 2'-10" | 7'-6" | 8-#9 | 0.1818 |
| 3'-0" | 3'-6" | 9'-0" | 11-#9 | 0.2618 |
| 3'-6" | 4'-0" | 10'-6" | 14-#9 | 0.3563 |
| 4'-0" | 4'-6" | 12'-0" | 19-#9 | 0.4654 |
| 4'-6" | 5'-0" | 13'-0" | 24-#9 | 0.5890 |
| 5'-0" | 5'-6" | 14'-0" | 29-#9 | 0.7272 |
| 5'-6" | 6'-0" | 15'-0" | 35-#9 | 0.8799 |
| 6'-0" | 6'-6" | 16'-0" | 41-#9 | 1.0472 |
Concrete Quantities shown in table are per linear foot of shaft only. Bell Quantities are not included.
(*) Amount of reinforcing may be increased from that shown to meet the individual job requirements.
Minimum reinforcement meets AASHTO Spec. 8.18 for reinforcement of compression members.
DETAILS
SEISMIC PERFORMANCE CATEGORY B, C & D

| Diameter of Shaft |
Minimum (*) Reinf. |
Cubic Yards Concrete per ft. |
| 2'-0" | 8-#7 | 0.1164 |
| 2'-6" | 8-#9 | 0.1818 |
| 3'-0" | 11-#9 | 0.2618 |
| 3'-6" | 14-#9 | 0.3563 |
| 4'-0" | 19-#9 | 0.4654 |
| 4'-6" | 24-#9 | 0.5890 |
| 5'-0" | 29-#9 | 0.7272 |
| 5'-6" | 35-#9 | 0.8799 |
Concrete Quantities shown in table are per linear foot of shaft only.
(*) Amount of reinforcing may be increased from that shown to meet the individual job requirements.
Minimum reinforcement meets AASHTO Spec. 8.18 for reinforcement of compression members.
(**) Stay in place casing may be used in place of spirals for column diameters greater than 4 foot.
751.40.8.10 Prestressed Concrete I-Girders
751.40.8.10.1 Design
751.40.8.10.1.1 Girder Design
Geometric Dimensions
Girder Analysis (Continuous Span Series)
Stresses due to dead load weight of slab, girder, diaphragms, haunch and forms will be based on simple spans from centerline to centerline of bearings.
Stresses due to dead load weight of curbs, parapet, rails, future wearing surface and outlets will be based on continuous composite spans with loads equally distributed to all girders. The span lengths used in these computations will be based on the distance from the centerline of the bearing at the End Bent to the centerline of the Int. Bent, and from centerline of Int. Bent to centerline of Int. Bent.
Stresses due to live load plus impact will be based on continuous composite spans whose lengths are described above for curbs, etc.
The analysis will be made on the basis of transformed areas of all steel (both strands and bars) in the section using concrete with Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} = 6.
In composite design, allowances shall be made for the difference in modulus of elasticity of slab and girder by using the effective slab area as specified for concrete T-Beams as given in the current AASHTO Specifications, multiplied by the factor . The area shall include the transformed area of all longitudinal reinforcing bars within the effective width. The 1" integral wearing surface shall not be used in the effective slab depth.
Effective Flange Width
The effective flange width for Beam Types 2,3,4 & 6 should be calculated using AASHTO 8.10.1. For Beam Type 7, the effective flange width should be calculated using AASHTO 9.8.3.
Continuity at Intermediate Supports
Continuity will be obtained at intermediate supports by pouring a concrete diaphragm monolithic with the deck slab and encasing the prestressed girders. Reinforcing bars will tie the slab, diaphragms and girders together.
Reinforcing bars, = 60,000 psi, will be placed in the deck slab for tensile steel.
The ultimate negative moments should be 2.17 times the maximum live load moments including impact and 1.3 times moments for future wearing surface and dead load of curb, parapet or safety barrier curb and bridge rail.
The area of longitudinal reinforcing steel at the centerline of the intermediate bent should be determined on the basis of a cracked section. This area of reinforcing bars is to be provided by adding additional bars between the normal longitudinal bars at the top of the slab. #8 maximum bar size for additional bars over bents.
These special negative moment reinforcing bars should be ended by one of the following criteria (whichever is greater):
- Where the stress on the normal longitudinal reinforcing bars does not exceed 24,000 psi. as based on a cracked section, plus 15 bar diameters or development length.
- Not closer to the centerline of the intermediate bent than 1/10 of the span. (8' min.)
The concrete stress at the bottom of the girder should be checked at a point 70 strand diameters plus 9 inches from the centerline of the intermediate bent to see that the total compressive stress due to prestress and negative moment does not exceed 3,000 psi. (AASHTO. 9.7.2)
The positive moment at the intermediate bent should be provided for by extending the top two rows of the top strands (both straight or deflected) and if available, the number of bottom strands indicated in tables below bent to form a right angle hook.
Design of Negative Moment Reinforcement
Since most of the dead load moments are carried by the beam acting as a simple span, the negative design moment over piers is the live load plus impact moment. In most designs, the dead load applied after continuity is achieved should also be considered in the negative design moment. The effect of initial precompression due to prestress in the precast girders may be neglected in the negative moment computation of ultimate strength if the maximum precompression stress is less than and the continuity reinforcement is less than 1.5 percent.
It will usually be found that the depth of the compression block will be less than the thickness of the bottom flange of the precast girder. For this reason, the negative moment reinforcement required can be determined by assuming the beam to be a rectangular section with a width equal to the bottom flange width of the girder. Due to the lateral restraint of the diaphragm concrete, ultimate negative compression failure in the PCA tests always occurred in the girders, even though the diaphragm concrete strength was about 1000 psi less than that of the girder concrete for this reason, it is recommended that the negative moment reinforce-ment be designed using the compressive strength of the girder concrete.
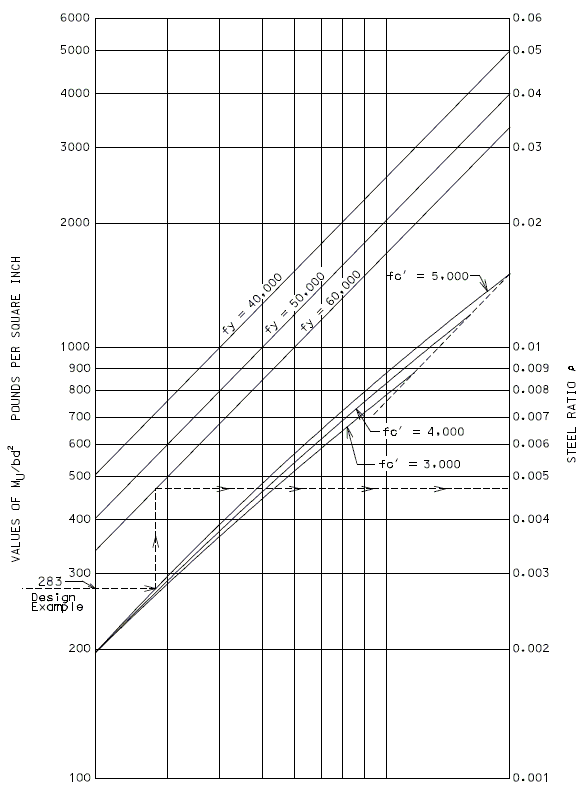
| Web Thickness (Inches) |
Number of Bottom Strands for Positive Moment Connection (C) for Closed Diaphragms | ||||
|---|---|---|---|---|---|
| Beam Type 2 or Modified |
Beam Type 3 or Modified |
Beam Type 4 or Modified |
Beam Type 6 or Modified |
Beam Type 7 or Modified | |
| 6 | 6 | 8 | 10 | -- | 18 |
| 6 1/2 | -- | -- | -- | 14 | -- |
| 7 (A) | 8 | 10 | 10 | -- | -- |
| 7 1/2 (B) | -- | -- | -- | 16 | -- |
| 8 (A) | 8 | 10 | 12 | -- | -- |
| 8 1/2 (B) | -- | -- | -- | 16 | -- |
| Web Thickness (Inches) |
Number of Bottom Strands for Positive Moment Connection (C) for Open Intermediate Diaphragms with Continuous Superstruecture | ||||
|---|---|---|---|---|---|
| Beam Type 2 or Modified |
Beam Type 3 or Modified |
Beam Type 4 or Modified |
Beam Type 6 or Modified |
Beam Type 7 or Modified | |
| 6 | 12 | 16 | 16 | -- | 22 |
| 6 1/2 | -- | -- | -- | 22 | -- |
| 7 (A) | 12 | 16 | 16 | -- | -- |
| 7 1/2 (B) | -- | -- | -- | 22 | -- |
| 8 (A) | 12 | 16 | 16 | -- | -- |
| 8 1/2 (B) | -- | -- | -- | 22 | -- |
| (A) | Modified Beam Type 2, 3 or 4. |
| (B) | Modified Beam Type 6. |
| (C) | If available, otherwise bend all bottom strands. |
Negative Moment Bar Cut-Off (Working Stress Controlling)
Area of slab bars required and stress in the slab bars are printed in program BR200.
Determine stress of the area of slab bars input into program at a point where the area required is larger than that input.
Interpolate along a straight line to where the stress is 24,000 psi.
Note: Negative moment bar computations use a cracked section analysis to determine stresses.
751.40.8.10.1.2 Allowable Concrete Stresses
The following criteria is shown for clarity and is in accordance with AASHTO 9.15.
- = 5,000 psi, = 4,000 psi
A. Temporary stresses before losses except as noted:
- Compression... (*)
- Tension
- Precompressed tensile zone ....................
- No temporary allowable stresses are specified. See paragraph "B" below.
- In tension areas with no bonded reinforcement...
- Where the calculated tensile stress exceeds this value, bonded reinforcement shall be provided to resist the total tension force in the concrete computed on the assumption of an uncracked section. The maximum tensile stress shall not exceed...
- Precompressed tensile zone ....................
B. Stresses at service loads after losses:
- Compression...
- Tension in the precompressed tensile zone...
- (a) For members with bonded reinf. (**)...
- (b) For members without bonded reinf...
- Tension in other areas
- Tension in other area is limited by the allowable temporary stresses specified in "A" above.
C. Cracking stress:
- Modulus of rupture from tests or (for normal weight concrete)...
D. Negative moment stresses in girders made continuous after deadload of slab is in place:
- Tension in negative moment reinforcement... = 60,000 psi, = 24,000 psi
- Compression in concrete at bottom of girder... = 5,000 psi,
(*) BR200 allows 2% overstress
(**)Strands qualify if not debonded at ends.
751.40.8.10.1.3 Prestress Loss and Prestress Camber
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Bigg[ \cfrac{ \overset{(SH)}{6,000} + \overset{(ES)}{ \frac {Es}{Ec_i}}fc + \overset{(CR_C)}{8.5 fc} + (5,000 - \overset{(CR_s)}{0.1 ES} - 0.05 (SH + CR_c))}{fs_i} \Bigg]}
| Reduce to: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \cfrac {10,700 + (0.9 \left( \frac{E_s}{Ec_i} \right) + 8.08) fc}{fs_i}} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, SH} | = Shrinkage |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, ES} | = Elastic Strain | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, CR_c} | = Concrete Creep | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, CR_s} | = Steel Creep |
| = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 12 fc_{ir} - 7 fc_{ds}} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, CR_c} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 12 fc - 7/2 fc = 8.5 fc} (Approximate Estimate) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Ec_i} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 150^{1.5} 33 \sqrt{f'c_i}} (Approximate Estimate) |
| = Concrete stress at centroid of P/S steel at point considered due to P/S and dead load at release. | |
| = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fc_{ir}} (Assume Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fc_{ir} = fc} ) | |
| = (Estimate average) | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fc_{ds}} | = Concrete stress at centroid of P/S Steel (due to dead load)(Assume fcds = 1/2 fc) |
| = Initial stress in P/S steel | |
| = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 270,000 psi \times 75% = 202,500 psi} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Ec_i} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 150^{1.5} 33 \sqrt{4,000} = 3,834,253.5 psi} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Es} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 28,000,000 psi} (AASHTO 9.16.2.1) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac {Es}{Ec_i}} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 7.30} |
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac {10,700 + (0.9 \times 7.30 + 8.08) 1,600}{202,500} = 16.9%}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 202.5ksi \times 16.9% = 34.22ksi}
Total loss due to all causes, except friction, is 34.22 ksi. (Friction losses are applied to post-tensioned girder only.) Use 8.84% for initial loss and 8.84% for final loss for design.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 202.5 ksi \times 8.84% = 17.90 ksi}
= initial loss
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 202.5 - 17.90 = 184.60ksi}
= final loss
= total loss
In the above design example, if tension exceeds AASHTO Specifications, (425 psi for 5,000 psi concrete) the girder will have to be modified to limit stress to 425 psi.
| = 6,000 psi | |
| = 4,500 psi | |
| Grade 270 low relaxation strands | |
| = (Estimated average) | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Ec_i} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 150^1.5 33 \sqrt{f'c_i} = 4,066.840 psi} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{Es}{Ec}} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{28,000,000}{4,066,840} = 6.89} |
| AASHTO 9.16.2.1.3: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, CRc = 12fc - 7/2fc = 8.5fc} (approximate estimate) | |
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \Bigg[ \overset{(SH)}{6,000} + \overset{(ES)}{ \frac {Es}{Ec_i}}fc + \overset{CR_c)}{8.5 fc} + (5,000 - \overset{(CR_s)}{0.1ES} - 0.05(SH + CR_c)) \Bigg]}
Reduce to: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \cfrac{10,700 + (0.9 (\frac{Es}{Ec_i}) + 8.08) fc}{fs_i}}
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fc} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 0.4(4,500) = 1,800 psi} (estimated average) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{Es}{Ec_i}} | = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 6.89} |
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fs_i} = Initial stress in low relaxation strands stressed to 75% of ultimate (*)
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fs_i} = 270,000 psi \times 75% = 202,500 psi
= total loss except friction
Use 9.44% for initial loss and 9.44% for final loss.
= initial loss
= final loss
= total loss
P/s force initial =
P/s force final = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, ((202.5 - 36.43) ksi)(0.153 in.^2/strand)(no.\ of\ strands)}
(*) Suggested by FHWA: when using 3/8" round strands, max. Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fs_i = 0.7 \times 250 ksi\ or\ 0.7 \times ultimate\ stress}
, whichever is smaller. Larger initial stresses will cause debonding.
Prestress Concrete Girder Formula for Stress Calculation
(-) Tension; (+) Compression
Temp. Stress
| Allow Top | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 7.5 \sqrt{f'c_i} = 0.474ksi} tension for | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'c_i = 4,000psi} |
| Bottom | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 0.6 f'c_i = 2.4 ksi} compression for |
- Temp. Top =
- Temp. Bottom =
Design Load Stress
| Allow Top | compression for | |
| Bottom | tension for |
- Top final =
- Bottom final =
- 0.153 sq. in. = Area of one 1/2 inch strand
- 270 ksi = f's = Ult, Str. P/S Strand
- 202.5 ksi = 0.75 (270) = Initial steel stress
- 0.0884 = 8.84% Initial loss - low relaxation
- 0.0884 = 8.84% Final loss - low relaxation
- 4 Str. 2 Draped
- 202.5 (0.153) = 30.98 kips/Str. P/s force
- 6 Strands (30.98) = 185.90 P/s force
| = Area Composite | |
| = Area Girder | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Ecc_c} | = Eccentricity of prestress force of composite section |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Ecc_{nc}} | = Eccentricity of prestress force of non-composite section |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_{DFLC}} | = Composite dead load moment |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_{Gdr}} | = Girder dead load moment |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, M_{LL+I}} | = Live load + impact moment |
| = Slab + diaphragm moment | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P/S\ F} | = Prestress forces in girder |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Sb_c} | = Composite section modulus at bottom of girder |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Sb_{nc}} | = Non-composite section modulus at bottom of girder |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, St_c} | = Composite section modulus at top of girder |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, St_{nc}} | = Non-composite section modulus at top of girder |
Prestress Camber
Reference: Computer Program BR139B
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \begin{Bmatrix} I4 = 107,888 in.^4\\ (non-transformed)\\ Beam\ wt. = 0.541\ (k/ft.) \end{Bmatrix} }
Used to resist uplift before beam is set on bent.
Used after beam is in place.
| Mult. factor | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, [1 + (1 - e^{-\phi})] = 1.77} |
| |||||||||||||||||
| = 1.77 | |||||||||||||||||||
| =2.718 | |||||||||||||||||||
| = \varepsilon\ creep \times E_{28\ days} | |||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \varepsilon\ creep} | = (See page 3 PCA design of precast prestressed concrete girders. Use 40% factor based on creep at erection for 28 days.) | ||||||||||||||||||
The following formulas are used to determine:
- Camber due initial strand stress (inch),
- deflection due beam weight (inch),
- camber due strands, beam weight and 28 day creep (inch),
- camber L/4 due strands, beam weight and 28 day creep (inch),
- deflection due to slab weight (inch),
- camber centerline due strands, beam weight, 28 day creep, slab and diaphragm (inch), and
- camber quarterpoint due strands, beam weight, 28 day creep, slab and diaphragm (inch).
Formulas used:
Positive deflect up
Negative deflect down Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \downarrow}
Beam weight camber
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \downarrow \triangle_2 = \frac{5W_B(L^4)}{384E_iI} (1728 \times 10^3)}
Slab weight camber
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \downarrow \triangle_s = \Bigg[ \frac{5W_s(L^4)}{384E_fI_{TR}} + \frac{P(L^3)}{48E_fI_{TR}} + \frac{2PsX(3L^2 - 4X^2)}{48E_fI_{TR}} \Bigg] (1728 \times 10^3)}
Force straight strands (1/2" low relaxation strands)
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_{01} = (no.\ of\ straight\ strands) \times \big[ 31.0 - (17.1 \times 0.153) \big] kips}
Force draped strands ( 1/2 " low relaxation strands)
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_{02} = (no.\ of\ draped\ strands) \times \big[ 31.0 - (17.1 \times 0.153) \big] kips}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 270 ksi \times 75% \times (0.153 sq.\ in.) = 31\ kips\ per\ strand}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 202.5 \times (1-0.0884) = 184.6 ksi}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 184.6 \times (1-0.0884) = 168.28 ksi}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 202.5 - 168.28 = 34.22 ksi = Total\ loss}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Average\ loss = Totalloss/2 = 34.22/2 = 17.1 ksi}
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e_1} | = dist. centroid beam to centroid straight strand (in.) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e_2} | = dist. centroid beam to low centroid draped at center of beam (in.) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e_3} | = dist. centroid beam to up centroid draped at end of beam (in.) |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L} | = length (ft.) (cneterline bearing to centerline bearing). |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle I} | = moment of inertia (in.2) non-transformed. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle I_{TR}} | = moment of inertia (in.2) transformed. |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Ps} | = concentrated loads due to variable slab thickness on each end. |
| = dist. from centerline brg. to Ps. | |
| = concentrated load due to diaphragm at center of span (kips) | |
| = uniform beam load (kips/ft.) | |
| = uniform slab load (kips/ft.) | |
| = factor for 28 day creep | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E_i} | = modulus of elasticity corresponding to initial girder concrete strength |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E_f} | = modulus of elasticity corresponding to final girder concrete strength |
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \triangle Centerline = F( \triangle_1 - \triangle_2) - \triangle_s}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \triangle\ at\ 0.10 = 0.314 ( \triangle\ at\ Centerline) }
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \triangle\ at\ 0.20 = 0.593 ( \triangle\ at\ Centerline) }
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \triangle\ at\ 0.25 = 0.7125 ( \triangle\ at\ Centerline) }
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \triangle\ at\ 0.30 = 0.813 ( \triangle\ at\ Centerline) }
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \triangle\ at\ 0.40 = 0.952 ( \triangle\ at\ Centerline) }
Note: Compute and show on plans camber at 1/4 points for bridges with spans less than 75', 1/10 points for spans 75' and over.
751.40.8.10.1.4 Superstructure Design
Live Load Distribution
The live load distribution to girders may be assumed to be the same as the AASHTO distribution for concrete floors on steel I-Beam stringers. These factors may be found in EPG 751.40.8.2 Distribution of Loads.
Ultimate Load Capacity
The ultimate load capacity shall be not less than 1.3 times (the weight of the girder plus the weight of the slab and diaphragms plus the weight of the future wearing surface) plus 2.17 times the design live load plus impact.
Ultimate Strength
The ultimate moment on a prestressed girder as determined in accordance with the ultimate load capacity indicated above, shall not be greater than the ultimate strength determined as follows:
| Where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, t \le 0.2d} | Where | |
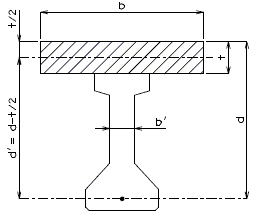 |
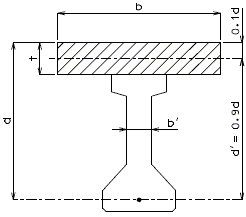
| |
or |
Use the lesser in each case |
or |
Where:
| = Area of p/s strands in bottom flange | |
| , , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, t} & Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, d} | = as shown above |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_s} | = Ultimate strength of p/s strands |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} | = Ultimate strength of slab concrete = 4,000 psi |
Maximum Prestressing Steel Area
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A_s = \frac{0.85 f'_c b t}{f'_s}} When Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, t \le 0.2d}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, a_s = \frac{0.85 f._c b (0.2d)}{f'_s}} When
In lieu of the above, AASHTO - Article 9.17 & 9.18 may be used. (This is the method used by computer program BR200)
751.40.8.10.1.5 Web Reinforcement
(5" Min. - 21" Max. bar spacing for #4 bars) (5" Min. - 24" Max. bar spacing for #5 bars)
(*) Prestressed concrete members shall be reinforced for diagonal tension stresses. Shear reinforcement shall be placed perpendicular to the axis of the member. The formula to be used to compute areas of web reinforcement is as follows:
- Where but not more than
- But shall not be less than .
(**) Since large moments and large shears occur in the same area of the girder near the interior supports, the AASHTO formula (AASHTO - 9.20 -Shear) for computing the area of web reinforcement has been modified. The formula to be used to compute areas of web reinforcement near interior supports is as follows:
The value "jd" is the distance from the slab reinforcement to the center-of-gravity of the compression area under ultimate loads.
Use #4 shear reinforcement when possible. Alternate B1 bar will not work with #5.
Anchorage Zone Reinforcement - AASHTO Article 9.21.3
The following detail meets the criteria for anchorage zone reinforcement for pretensioned girders (AASHTO Article 9.21.3) for all MoDOT standard girder shapes.
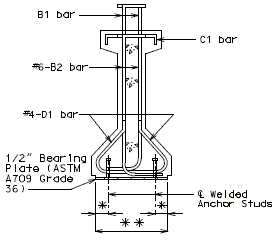
|

|
| * | 2 3/4" (Type 2, 3 & 4) 5 1/4" (Type 6) |
| ** | 15 1/2" (Type 2, 3 & 4) 22 1/2" (Type 6) |
Sole Plate Anchor Studs
The standard 1/2" sole plate will be anchored with four 1/2" x 4" studs.
Studs shall be designed to meet the criteria of AASHTO Div. I-A in Seismic Performance Category C or D.
Stud capacity is determined as follows:
Where:
| = no. of studs | |
| = area of stud | |
| = yield strength of stud (50 ksi) | |
| = Allowable Shear in Pins AASHTO Table 10.32.1A | |
| = seismic overload factor |
If required, increase the number of 1/2" studs to six and space between open B2 bars. If this is still not adequate, 5/8" studs may be used. The following table may be used as a guide to upper limits of dead load reactions:
| The minimum 3/16" fillet weld between the 1/2" bearing plate and 1 1/2" sole plate is adequate for all cases. |
| ||||||||||||||||||||||
751.40.8.10.1.6 Strands – Miscellaneous
Detensioning
In all detensioning operations the prestressing forces must be kept symmetrical about the vertical axis of the member and must be applied in such a manner as to prevent any sudden or shock loading.
General Information
Splicing:
One approved splice per pretensioning strand will be permitted provided the splices are so positioned that none occur within a member. Strands which are being spliced shall have the same "Twist" or "Lay". Allowance shall be made for slippage of the splice in computing strand elongation.
Wire failure:
Failure of one wire in a seven wire pretensioning strand may be accepted, provided that, it is not more than two percent of the total area of the strands.
Sand Blasting:
On structures where it is questionable as to the clarity of areas to be sandblasted: show limits of sandblasted area in a plan view of details on girder ends (bent sheet). However, generally, sandblasting is covered by Missouri Standard Specification 705.4.14.
751.40.8.10.2 Length
751.40.8.10.2.1 Structure Length
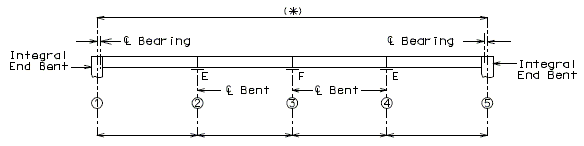
(Integral End Bents)
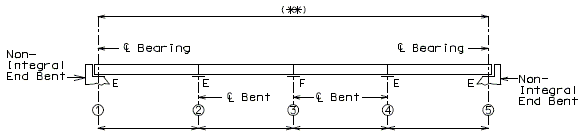
(Non-Integral End Bents)
751.40.8.10.3 Miscellaneous Details
751.40.8.10.3.1 Shear Blocks
A minimum of two Shear Blocks 12" wide x ![]() high by width of diaphragm, will be detailed at effective locations on open diaphragm bent caps when adequate structural restraint cannot be provided for with anchor bolts.
high by width of diaphragm, will be detailed at effective locations on open diaphragm bent caps when adequate structural restraint cannot be provided for with anchor bolts.
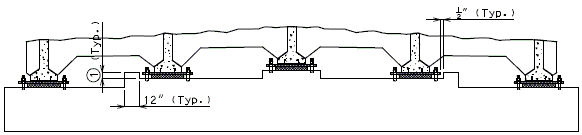
| Height of shear block shall extend a minimum of 1" above the top of the sole plate. |
751.40.8.10.3.2 Anchor Bolts
Simple Spans
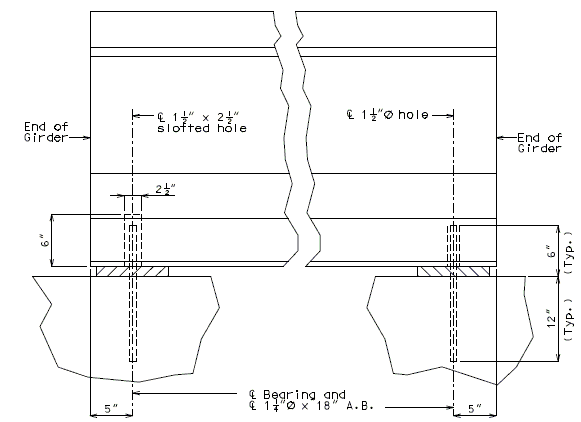
| |
| Expansion | Fixed |
|---|---|
| Part Elevation | |
| Note: | It is permissible for the reinforcing bars and or the strands to come in contact with the materials used in forming A.B. holes. |
| If A.B. holes are formed with galvanized sheet metal, the forms may be left in place. | |
| Hole (1 1/2" round) to be grouted with expansive type mortar. |
751.40.8.10.3.3 Dowel Bars
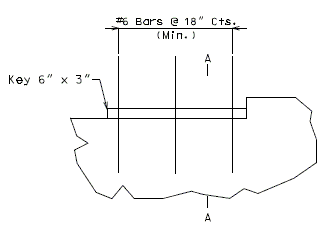
|
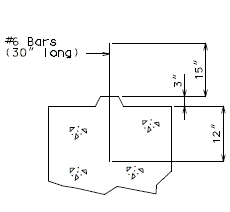
|
| Part Elevation (Fixed Bent) (*) |
Section A-A (*) |
|---|
| (*) | Details shown are for SPC A and B only. |
Dowel bars shall be used for all fixed intermediate bents under prestressed superstructures.
Seismic Performance Category A:
- Use #6 Bars @ 18" Cts. for dowel bars.
Seismic Performance Category B:
- Dowel bars shall be determined by design. (#6 Bars @ 18" Cts. minimum)
- Design dowel bars for shear using service load design.
- Allowable stresses are permitted to increase by 33.3% for earthquake loads.
Seismic Performance Categories C & D:
- See Structural Project Manager.
751.40.8.10.3.4 Expansion Device Support Slots
|
| Part Plan of P/S Concrete I-Girder @ Expansion Device End |
|---|
|
| Part Elevation of P/S Concrete I-Girder @ Expansion Device End |
| (*) | Show these dimensions on the P/S concrete girder sheet. |
751.40.8.11 Open Concrete Intermediate Bents and Piers
751.40.8.11.1 Design
751.40.8.11.1.1 General and Unit Stresses
GENERAL
Use Load Factor design method, except for footing pressure and pile capacity where the Service Load design method shall be used.
In some cases, Service Load design method may be permitted on widening projects, see Structural Project Manager.
The terms, Intermediate Bents and Piers, are to be considered interchangeable for this Manual Section.
DESIGN UNIT STRESSES
(1) Reinforced Concrete
Class B Concrete (Substructure) = 1,200 psi = 3,000 psi Reinforcing Steel (Grade 60) = 24,000 psi Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 60,000 psi n = 10 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c = W_1.5 \times 33 \sqrt{f'_c}} (AASHTO Article 8.7.1) (*)
(2) Reinforced Concrete (**)
Class B-1 Concrete (Substructure) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_c} = 1,600 psi Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f'_c} = 4,000 psi Reinforcing Steel (Grade 60) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 24,000 psi = 60,000 psi Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} = 8 Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c = W_1.5 \times 33 \sqrt{f'c}} (AASHTO Article 8.7.1) (*)
(3) Structural Steel
- Structural Carbon Steel (ASTM A709 Grade 36)
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 20,000 psi, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 36,000 psi
(4) Overstress
- The allowable overstresses as specified in AASHTO Article 3.22 shall be used where applicable for Service Load design method.
(*) Use Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, W = 150 \ pcf, \ E_c = 60,625 \sqrt{f'_c}}
(**) May be used for special cases, see Structural Project Manager.
751.40.8.11.1.2 Loads
(1) Dead Loads
(2) Live Loads
- As specified on the Design Layout.
- Impact of 30% is to be used for the design of beam, web supporting beam and top of columns. No impact is to be used for bottom of column, tie beam or footing design.
(3) Wind and Frictional Forces
(4) Temperature and Shrinkage
- The effect of normal and parallel components to the bent shall be considered. When bearings with high friction coefficients are used or for long bridge lengths, the columns and footings are to be analyzed for moments normal to the bent due to the horizontal deflection of the top of the bent.
(5) Buoyancy
- If specified by the Structural Project Manager, or by the Design Layout.
(6) Earth Pressure
- Bents are to be analyzed for moments due to equivalent fluid pressure on columns and web where the ground line at time of construction, or potential changes in the ground line, indicate.
(7) Earthquake
- See Structural Project Manager or Liaison.
(8) Special Stability Situations
- When indicated by the Design Layout or by the Structural Project Manager, piers must be analyzed for special loadings as directed (i.e., differential settlement).
(9) Collision
- Where the possibility of collision exists from railroad traffic, the appropriate protection system, for example Collision Wall, shall be provided (See the Design Layout).
(10) Collision Walls
- Collision walls are to be designed for the unequal horizontal forces from the earth pressure, if the condition exists (See the Design Layout). The vertical force on the collision wall is the dead load weight of the wall (*). If a bent has three or more columns, design the steel in the top of the wall for negative moment.
(*) For footing design, the eccentricity dead load moment due to an unsymmetrical collision wall shall be considered.
751.40.8.11.1.3 Distribution of Loads
(1) Dead Loads
- Loads from stringers, girders, etc. shall be concentrated loads applied at the centerline of bearing. Loads from superstructure, such as concrete slab spans, shall be applied as uniformly distributed loads.
(2) Live Loads
- Loads from stringers, girders, etc., shall be applied as concentrated loads at the intersection of centerline of stringer and centerline of bent.
(3) Wind and Frictional Forces
(4) Temperature
- Apply at the top of the substructure beam.
(5) Earth Loads
- (a) Vertical
- Vertical earth loads on tie beams shall be applied as uniform loads for a column of earth equal to 3 times the width of the beam. The weight of earth for footing design shall be that directly above the footing, excluding that occupied by the column.
- The earth above the seal courses shall be considered in computing pile loads. Refer to the Design and Dimension of the Pile Footings portion of this Manual Section.
- (b) Horizontal
(*) A factor of 2.0 is applied to the moment to allow for the possibility of the column esisting earth pressure caused by the earth behind the column twice the column width.
(6) Earthquake Loads
- See Structural Project Manager or Liaison.
(7) Seal Course
- The weight of the seal course shall not be considered as contributing to the pile loads, except for unusual cases.
751.40.8.11.1.4 Types of Design
TYPES OF DESIGN
Rigid frame design is to be used for the design of Intermediate Bents and Piers.
The joint between the beam and column, and web or tie beam and column,
is assumed to be integral for all phases of design and must be analyzed
for reinforcement requirements as a "Rigid Frame".
The joint between the column and footing is assumed to be "fixed",
unless foundation fexibility needs to be considered (consult Structural
Project Manager for this assessment).
If the distance from the ground line to the footing is large (*),
consideration shall be given to assuming the column to be "fixed" at a
point below the ground line.
(*) When the distance from the ground line to the top of footing is 10' or more, the unsupported height and the fixed point may be measured from the bottom of the beam to the ground line plus 1/2 of the distance from the ground line to the top of the footing.
UNSUPPORTED HEIGHT
Unsupported height is the distance from the bottom of the beam to the top of the footing.
SINGLE COLUMN
Use rigid frame design with the column considered "fixed" at the bottom for all of the conditions.
COLUMN DIAMETER CHANGE
Use a change in column diameter as required by the Design Layout or column design.
COLUMN SPACING (TRIAL)
(Except Web Supporting Beam type)
Estimate centerline-centerline column spacing for a two column bent as 72% of the distance from the centerline of the outside girder to the centerline of the outside girder.
A three column bent spacing estimation is 44% of the centerline-centerline outside girder spacing.
751.40.8.11.2 Reinforcement
751.40.8.11.2.1 Hammer Head Type
 |
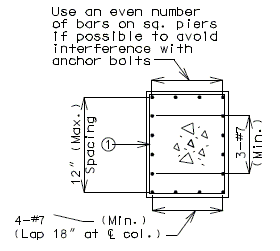
|
| PART PLAN | SECTION A-A |
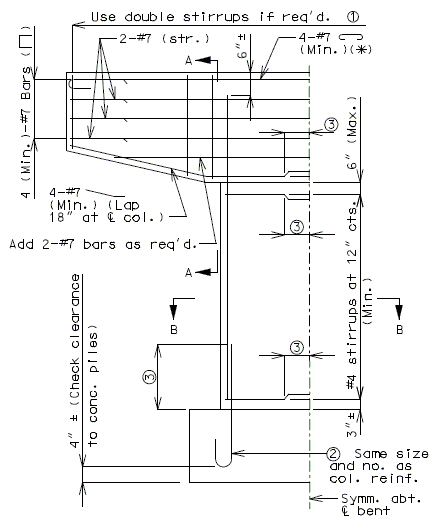 |
Note: When an expansion device in the slab is used at an intermediate bent, all reinforcement located entirely within the beam shall be epoxy coated. See details of protective coating and sloping top of beam to drain. |
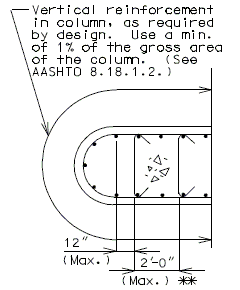
| |
| PART ELEVATION | PART SECTION B-B |
(*) Add hooked reinforcement as required by design.
(**) See AASHTO Article 8.18.2.3.4 for tie requirements.
![]() All stirrups in beam to be the same size bar. (Use a min. spacing of 5" (6" for double stirrups), minimum stirrups are #4 at 12" cts., and maximum
stirrups are #6 at 6" cts.)
All stirrups in beam to be the same size bar. (Use a min. spacing of 5" (6" for double stirrups), minimum stirrups are #4 at 12" cts., and maximum
stirrups are #6 at 6" cts.)
Locate #4 bars (┌─┐) under bearings if required. (Not required for P/S Double-Tee Girders.)
![]() See development length (Other than top bars) or standard hooks in
tension, Ldh.
See development length (Other than top bars) or standard hooks in
tension, Ldh.
751.40.8.11.3 Pile Footings
751.40.8.11.3.1 Design and Dimensions
GENERAL
- Number, size and spacing of piling shall be determined by computing the pile loads and applying the proper allowable overstresses.
- Cases of Loading: (AASHTO Article 3.22)
- Group I and Group II maximum vertical loads (refer to distribution of loads, this Section).
- Group III thru VI wind and/or temperature moments with applicable vertical loads.
- Internal stresses including the position of the shear line shall then be computed.
- Long narrow footings are not desirable and care should be taken to avoid the use of an extremely long footing 6~0" wide when a shorter footing 8'-3" or 9'-0" wide could be used.
- Footings are to be designed for the greater of the minimum moment requirements at the bottom of the column, or the moments at the bottom of the footing.
- When using the load factor design method for footings, design the number of piles needed based on the working stress design method.
PILE LOADS
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} - Pile Loads Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, N} - Vertical Loads - Number of Piles - Overturning Moment If minimum eccentricity controls the moment in both directions, It is necessary to use the moment in one direction (direction with less section modulus of pile group) only for the footing check. - Section Modulus of Pile Group
- (A) AASHTO Group I thru VI Loads as applicable
- Maximum = Pile Capacity
- Minimum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = 0} (zero)
- Tension on a pile will not be allowed for any combination of forces.
- Pile design force shall be calculated with consideration of AASHTO percentage overstress factors.
- (B) Earthquake Loads
See Structural Project Manager or Liaison before using the following seismic information.
- Point Bearing Pile
- Maximum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = Pile\ capacity\ \times 2} (**)
- (i.e., for HP 10 X 42 piles, Max. Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P = 56\ \times 2 = 112} tons/pile)
- Minimum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} = Allowable uplift force specified for piles in this
- Section under Seal Course Design.
- (**) Two "2" is our normal factor of safety. Under earthquake loadings only the point bearing pile and rock capacities are their ultimate capacities.
- Friction Piles
- Maximum = Pile Capacity
- Minimum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P} = Allowable uplift force specified for piles in this
- Section under Seal Course Design.
- See combined axial & bending stresses in Cast-In-Place friction piles in liquefaction areas.
(1) Shear Line
| If the shear line is within the column projected, the footing may be considered satisfactory for all conditions and standard #6 hairpin bars shall be used.
|
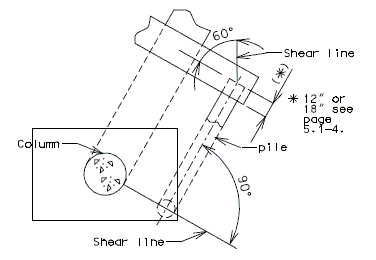
|
(2) Bending
The critical section for bending shall be taken at the face of the columns (concentric square of equivalent area for round columns).
The reinforcement shall be as indicated for reinforced footings, except that the standard #6 hairpin bars may be used for small footings if they provide sufficient steel area.
(3) Distribution of Reinforcement
Reinforcement in Bottom of Footing
Reinforcement shall be distributed uniformly across the entire width of footing in the long direction. In the short direction, the portion of the total reinforcement given by AASHTO Equation 4.4.11.2.2-1 shall be distributed uniformly over a band width equal to the length of the short side of the footing, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, B} .
- Band Width Reinforcement = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2(total\ reinforcement\ in\ short\ direction)/(\beta + 1)}
- where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \beta = the\ ratio\ of\ fooring\ lenth\ to\ width = L/B}
The remainder of the reinforcement required in the short direction shall be distributed uniformly outside the center band width of footing.
Reinforcement in Top of Footing
Reinforcement in the top of the footing shall be provided based on a seismic analysis for Seismic Performance Categories B, C and D. This reinforcement shall be at least the equivalent area as the bottom steel in both directions. The top steel shall be placed uniformly outside the column.
(4) Shear
(AASHTO Article 8.15.5 or 8.16.6)
The shear capacity of footing in the vicinity of concentrated loads shall be governed by the more severe of the following two conditions.
(i) Beam shear
Critical Section at "d" distance from face of column.
| b = Footing Width Service Load | 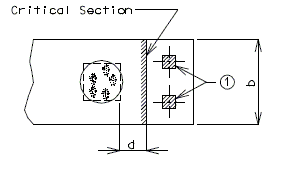
|
| Service Load | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, v = V / \left(b d \right)} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, v_c = 0.95 \sqrt{f'_c}} | |
| Load Factor | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V_u / \left(\omega b d \right)} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, v_c = 2.0 \sqrt{f'_c}} | PART PLAN OF FOOTING |
|---|
(ii)Peripheral Shear
Critical Section at "d/2" distance from face of column.
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, b_o = 4(d + Equiv.\ square\ column\ width)}
| Service Load | 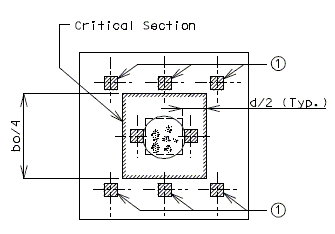
|
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, v = V / \left(b_o d \right)} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, v = 1.8 \sqrt{f'_c}} | |
| Load Factor | |
| PART PLAN OF FOOTING |
|---|
If shear stress is excessive, increase footing depth.
| Piles to be considered for shear. (Center of piles are at or outside the critical section.) |
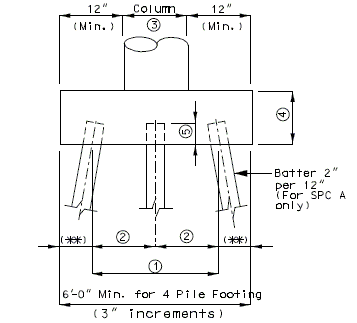 |
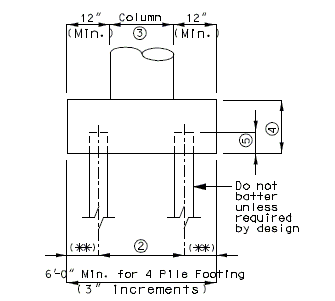
|
| SIDE ELEVATION | FRONT ELEVATION |
|---|
| Min. = 1/8 x (Distance from top of beam to bottom of footing.) | |
| 3'-0" (Min.) & 6'-0" (Max.) for steel HP piles, 14" CIP piles. AASHTO Article 4.5.6.4 shall be considered if piles are situated in cohesive soils. | |
| 3D (Min.) and 6D (Max.) for 20" and 24" CIP piles. (D = pile diameter) | |
| Indicates column diameter, or column length or width on a hammer head pier. | |
| Min. = 2'-6" or column diameter (*) (Or width) for friction piles for SPC A. | |
| Min. = 3'-0" or column diameter (*) (Or width) for steel piles for SPC A. | |
| Min. = 3'-0" or column diameter (*) (Or width) for friction piles for SPC B,C,& D. | |
| Min. = 3'-6" or column diameter (*) (Or width) for steel piles for SPC B, C & D. | |
| 12" for seismic performance category A and 18" for SPC B, C, & D. | |
| (*) | For column diameters 4'-0" and greater use a 4'-0" min. footing thickness. |
| (**) | Use 18" for steel HP piles, 14" CIP piles, prescase and prestress piles. |
TYPICAL PLAN OF
3 PILE FOOTINGS
(minimum pile spacings)
NOTES:
Use 3- piles on exterior foorings only.
Use only HP 10 x 42 or friction piles on three pile footings.
TYPICAL PLAN
STAGGERED PILE
(7 Pile footings shall not be used.)
| If horizontal thrust requires pile batter- consult the Structural Project Manager. |
(*) The maximum pile spacing is 4'-0".
751.40.8.11.3.2 Reinforcement
Unreinforced Footing - Use only in Seismic Performance Category A
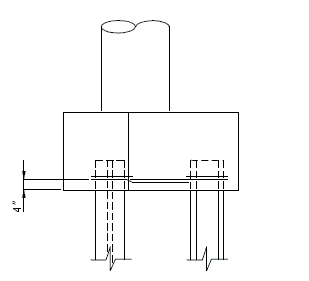
|
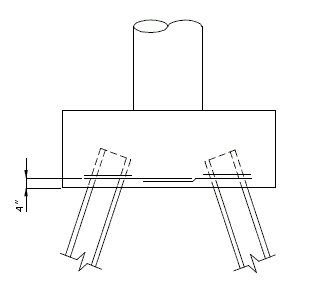
|
| Elevation (3 Pile Footing) |
Elevation (4 Pile Footing |
|---|---|
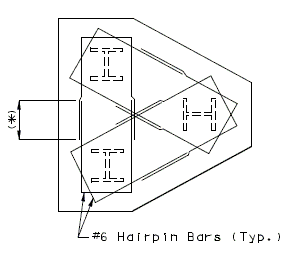
|
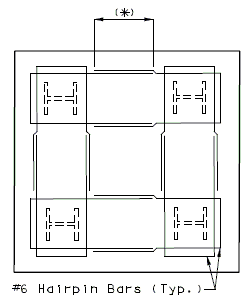
|
| Plan (3 Pile Footing) |
Plan (4 Pile Footing |
| (*) | See lap splice class C (Other than top bars). |
| Notes: | Reiforcement not required by design. Hairpins are sufficient for renforcing requirements. |
| The minimum percentage of reinforcement, "P" , is not required to be met, unless scour is anticipated. | |
| Use for all types of piling, except timber. |
Reinforced Footing - Seismic Performance Category A
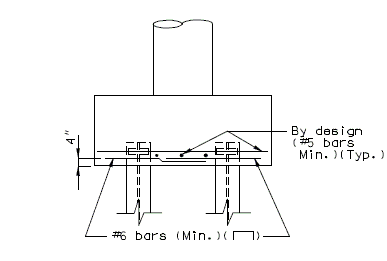
|
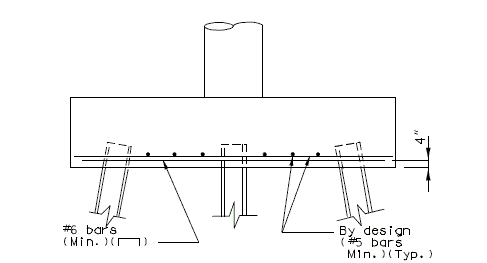
|
| Front Elevation | Side Elevation |
|---|---|
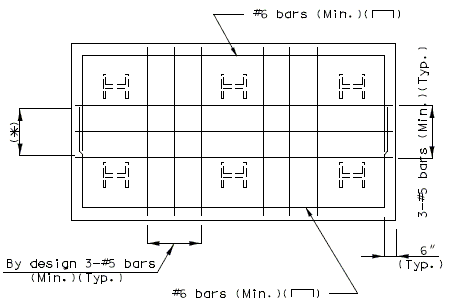
| |
| Plan | |
| (*) | See lap splice class C (Other than top bars). |
| Note: | The maximum size of stress steel allowed is #8 bars. |
Reinforced Footing - Seismic Performance Categorys B, C & D
See Structural Project Manager or Liaison before using the following seismic details.
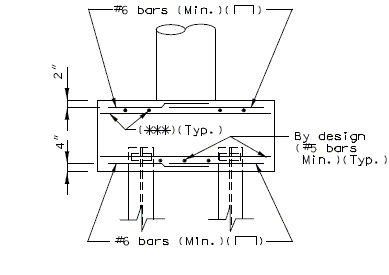
|
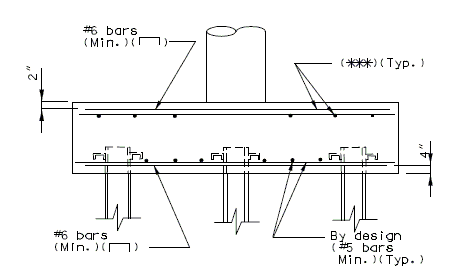
|
| Front Elevation | Side Elevation |
|---|---|
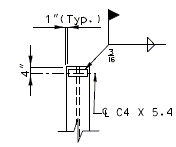
|
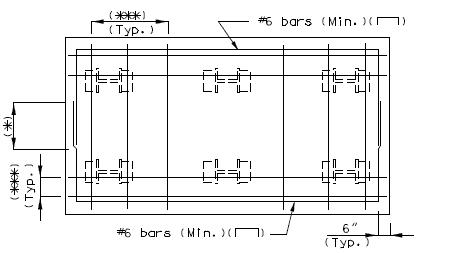
|
| Typical Detail Pile Channel Shear Connector | |
| Plan Showing Top Reinforcement | |
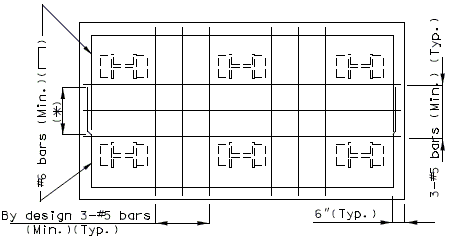
| |
| Plan Showing Bottom reinforcement | |
| (*) | For reinforcement in top of the footing, see lap splice class C (Top bars). |
| (**) | Place the top reinforcement uniformly outside the column. |
| (***) | Use same area of steel in the top of the footing as is required for the bottom. |
| Notes: | For reinforcement in bottom of the footing, see lap splice Class C (Other than top bars). |
| The maximum size of stress steel allowed is #8 bars. | |
| Unreinforced footings shall not be used in seismic performance categories B, C & D. |
751.40.8.11.4 Spread Footings
751.40.8.11.4.1 Design and Dimensions
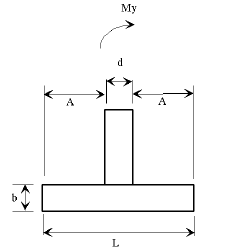
|
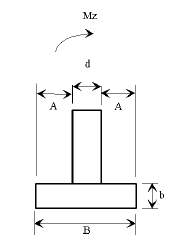
|
| Side Elevation | Front Elevation |
|---|
| d | = column diameter |
| L | = footing length |
| b | = footing depth |
| B | = footing width |
| A | = edge distance from column |
The calculated bearing pressure shall be less than the ultimate capacity of the foundation soil. The ultimate capacity of the foundation soil can be conservatively estimated as 2.0 times the allowable bearing pressure given on the Design Layout. The analysis method of calculating bearing pressures is outlined in the following information.
Dimensional Requirements
| L - | Minimum of 1/6 x distance from top of beam to bottom of footing (3" increments); |
| B - | Minimum footing width is column diameter + 2A, (3" increments); |
| A - | Minimum of 12"; |
| b - | Minimum of 30" or column diameter, Maximum of 72" at 3" increments; (for column diameters 48" and greater use a 48" minimum footing depth.) |
Size
The size of footing shall be determined by computing the location of the resultant force and by calculating the bearing pressure.
Long, narrow footings are to be avoided, especially on foundation material of low capacity. In general, the length to width ratio should not exceed 2.0, except on structures where the ratio of the longitudinal to transverse loads or some other consideration makes the use of such a ratio limit impractical.
Location of Resultant Force
The location of the resultant force shall be determined by the following equations.
The Middle 1/3 is defined as:
The Middle 1/2 is defined as: and
The Middle 2/3 is defined as: and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{e_B}{B} \le \frac{1}{3}}
The following table specifies requirements for the location of the resultant force.
| Soil Type | Resultant Location Group I - VI | Resultant Location Earthquake Loads Categories B, C and D |
|---|---|---|
| Clay, clay and boulders, cemented gravel, soft shale with allowable bearing values less than 6 tons, etc. | middle 1/3 | middle 1/2 |
| Rock, hard shale with allowable bearing values of 6 tons or more. | middle 1/2 | middle 2/3 |
Bearing Pressure
The bearing pressure for Group I thru VI loads shall be calculated using service loads and the allowable overstress reduction factors as specified in AASHTO Table 3.21.1A. The calculated bearing pressure shall be less than the allowable pressure given on the Design Layout.
The bearing pressure for Earthquake Loads in Categories B, C, and D shall be calculated from loads specified in AASHTO Division I-A Seismic Design, Sections 6.2.2, 7.2.1, and 7.2.2. The seismic design moment shall be the elastic seismic moment (EQ) divided by the modified response modification factor R'. The modified seismic moment shall then be combined independently with moments from other loads:
Group Load = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 1.0(D + B + SF + E + EQ/R')}
Where:
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, D} | = dead load |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, B} | = buoyancy |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, SF} | = stream flow pressure |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, EQ} | = elastic seismic moment |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E} | = earth pressure |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, R'} | = R/2 for category B |
| = 1 for categories C and D | |
| = Response Modification Factor | |
| = 5 for multi-column bent | |
| = 3 for single-column bent | |
The calculated bearing pressure shall be less than the ultimate capacity of the foundation soil. The ultimate capacity of the foundation soil can be conservatively estimated as 2.0 times the allowable bearing pressure given on the Design Layout. The analysis method of calculating bearing pressures is outlined in the following information.
| For | For |
|---|---|
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, q_{min} = L_1 = 3(L/2 - e_L)} | |
|
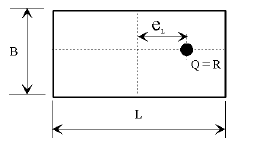
|
| Plan View | Plan View |
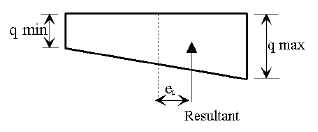
|
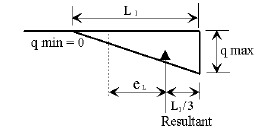
|
| Bearing Pressure | Bearing Pressure |
| CASE 1 | CASE 2 | |
|---|---|---|
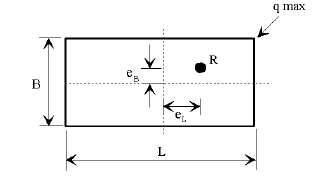
|
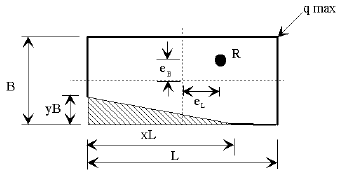
| |
| k, x and y from AASHTO chart | ||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, q_{max} = \frac{R}{BL} (1 + \frac{6e_L}{L} + \frac{6e_B}{B})} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, q_{max} = \frac{KR}{BL}} | |
| CASE 1 Plan View | CASE 2 Plan View | |
| CASE 3 | CASE 4 | |
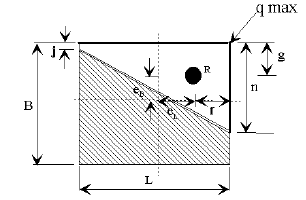
|
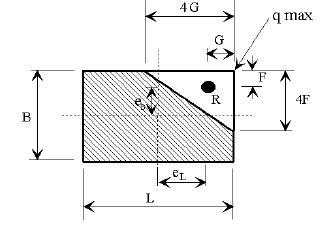
| |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, r = j/n} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, s = 1 + r + r^2} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, q_{max} = \frac{3R}{8FG}} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, g = \frac{n(1 + rs)}{4s}} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f = \frac{L(3s - r - 2)}{4s}} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, q_{max} = \frac{6R}{Lns}} | ||
| CASE 3 Plan View | CASE 4 Plan View | |
Loading Cases
Loads for Groups I thru VI shall be calculated for all bridges.
Earthquake loads shall be calculated when the bridge is in Seismic Zones B, C, and D.
Loads for other group loadings shall be used on a case by case basis.
Reinforcement
The footing is to be designed so that the shear strength of the concrete is adequate to handle the shear stress without the additional help of reinforcement. If the shear stress is too great, the footing depth should be increased.
Shear
The shear capacity of the footings in the vicinity of concentrated loads shall be governed by the more severe of the following two conditions.
Critical section at "d" distance from face of column:
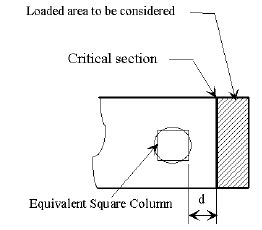
|
| Load Factor |
|---|
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V_n = V_u/(\phi bd)} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, V_c = 2 \sqrt{f'_c}} |
| = footing width |
Critical section at "d/2" distance from face of column:
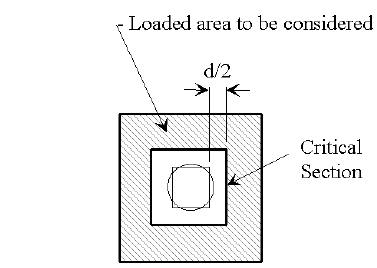
|
| Load Factor |
|---|
| If shear stress is excessive, increase footing depth. | |
Bending
If the shear line is within the projected equivalent square column, the footing may be considered satisfactory for all conditions. (minimum reinforcement required)
If the shear line is outside of the projected column, the footing must be analyzed and reinforced for bending and checked for shear stress.

The critical section for bending shall be taken at the face of the equivalent square column. The equivalent square column is the theoretical square column which has a cross sectional area equal to the round section of the actual column and placed concentrically.
Reinforcement in Bottom of Footing
The bearing pressure used to design bending reinforcement for Group I thru VI loads shall be calculated using Load Factor Loads.
The bearing pressure used to design bending reinforcement for Earthquake Loads in Categories B, C, and D shall be calculated from the same loads as specified in AASHTO Division 1-A Seismic Design for ultimate bearing pressure.
The bottom reinforcement shall be designed using ultimate strength design.
Distribution of Reinforcement
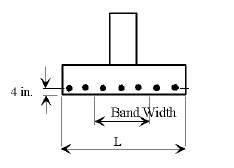
|
| L = Footing Length |
| B = Footing Width |
Reinforcement shall be distributed uniformly across the entire width of footing in the long direction. In the short direction, the portion of the total reinforcement given by AASHTO Equation 4.4.11.2.2-1 shall be distributed uniformly over a band width equal to the length of the short side of the footing, B.
Reinforcement in Top of Footing
Reinforcement in the top of the footing shall be provided for Seismic Performance Categories B, C, and D. This reinforcement shall be the equivalent area as the bottom steel in both directions. The top steel shall be placed uniformly outside the column.
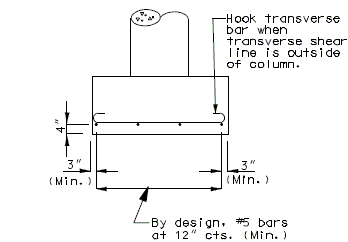
|
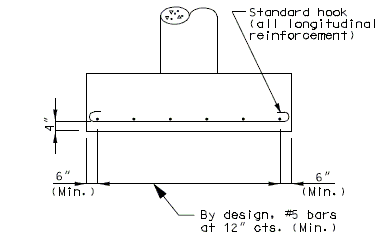
|
| Front Elevation | Side Elevation |
|---|
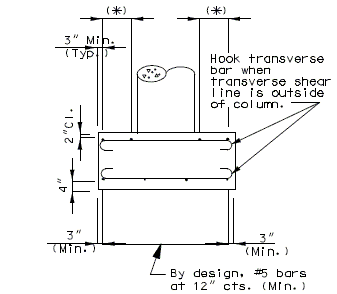
|
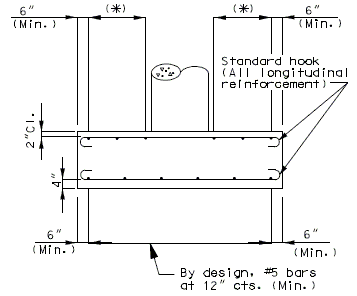
|
| Front Elevation | Side Elevation |
|---|
| (*) | Use same area of steel in the top of the footing as is required for the bottom. |
751.40.8.12 Concrete Pile Cap Intermediate Bents
751.40.8.12.1 Design
751.40.8.12.1.1 Unit Stresses
| (1) | Reinforced Concrete | ||
| Class B Concrete (Substructure) | = 1,200 psi | = 3,000 psi | |
| Reinforcing Steel (Grade 60 | = 24,000 psi | = 60,000 psi | |
| = 10 | |||
| (2) | Structural Steel | ||
| Structural Carbon Steel (ASTM A709 Grade 36) | = 20,000 psi | = 36,000 psi | |
| (3) | Piling | ||
| (4) | Overstress | ||
| The allowable overstresses as specified in AASHTO Article 3.22 shall be used where applicable for service loads. | |||
751.40.8.12.1.2 Loads
| (1) | Dead Loads |
| (2) | Live Load |
| As specified on Design Layout. | |
| Impact of 30% is to be used for design of the beam. No impact is to be used for design of any other portion of bent including the piles. | |
| (3) | Temperature, Wind and Frictional Loads |
751.40.8.12.1.3 Distribution of Loads
| (1) | Dead Loads |
| Loads from stringers, girders, etc. shall be concentrated loads applied at the intersection of centerline of stringer and centerline of bearing. Loads from concrete slab spans shall be applied as uniformly, distributed loads along the centerline of bearing. | |
| (2) | Live Load |
| Loads from stringers, girders, etc. shall be applied as concentrated loads at the intersection of centerline of stringer and centerline of bearing. For concrete slab spans distribute two wheel lines over 10'-0" (normal to centerline of roadway) of substructure beam. This distribution shall be positioned on the beam on the same basis as used for wheel lines in Traffic Lanes for Substructure Design. | |
| (3) | Temperature, Wind and Frictional Loads |
751.40.8.12.1.4 Design Assumptions
LOADINGS
| (1) | Beam | |
| The beam shall be assumed continuous over supports at centerline of piles. | ||
| Intermediate bent beam caps shall be designed so that service dead load moments do not exceed the cracking moment of the beam cap (AASHTO Article 8.13.3, Eq. 8-2). | ||
| (2) | Piles | |
| (a) | Bending | |
| Stresses in the piles due to bending need not be considered in design calculations for Seismic Performance Category A. | ||
| (b) | Dead Loads, etc. | |
| Dead load of superstructure and substructure will be distributed equally to all piles which are under the main portion of the bent. | ||
751.40.8.12.2 Reinforcement
751.40.8.12.2.1 General
PRESTRESS DOUBLE-TEE STRUCTURES

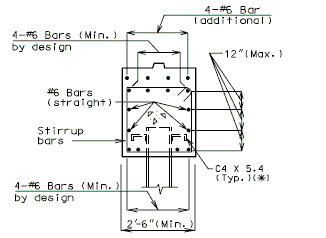
|
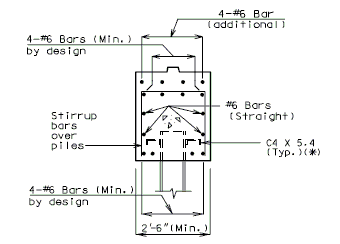
|
| SECTION A-A | SECTION B-B |
| (*) | Channel shear connectors are to be used in Seismic Performance Categories B, C & D. For details not shown, see this Section. |
| (**) | 2'-6" Min. for Seismic Performance Category A. 2'-9" Min. for Seismic Performance Categories, B, C & D. |
| Note: Use square ends on Prestress Double-Tee Structures. | |

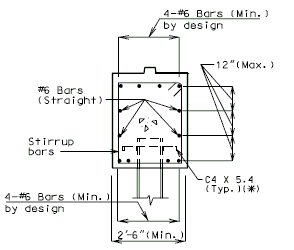 |

|
| SECTION A-A | SECTION B-B |
| (*) | Channel shear connectors are to be used in Seismic Performance Categories B, C & D. |
| (**) | 2'-6" Min. for Seismic Performance Category A. 2'-9" Min. for Seismic Performance Categories, B, C & D. |
| Note: Use square ends on Prestress Double-Tee Structures. | |
751.40.8.12.2.2 Anchorage of Piles for Seismic Performance Categories B, C & D
STEEL PILE

|
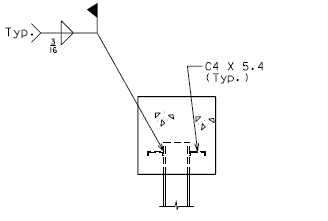
|
| PART ELEVATION | SECTION THRU BEAM |

| |
| PART PLAN |
CAST-IN-PLACE PILE
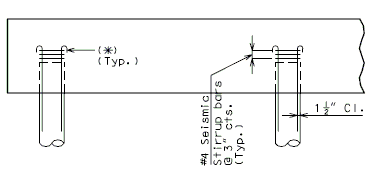
|
|
| PART ELEVATION | SECTION THRU BEAM |

| |
| PART PLAN |
751.40.8.12.2.3 Beam Reinforcement Special Cases
SPECIAL CASE I
If centerline bearing is 12" or less on either side of centerline piles, for all piles (as shown above), use 4-#6 top and bottom and #4 at 12" cts. (stirrups), regardless of pile size.
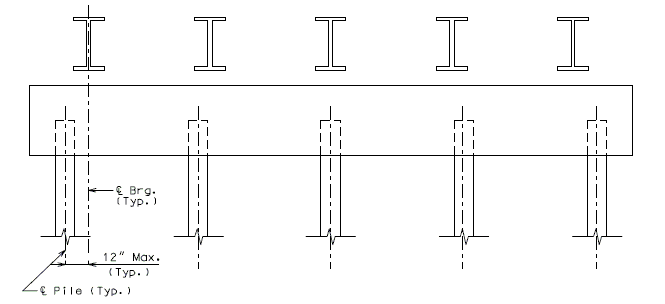
SPECIAL CASE II
When beam reinforcement is to be designed assuming piles to take equal force, design for negative moment in the beam over the interior piles.
(*) Dimensions shown are for illustration purposes only.
751.40.8.12.3 Details
751.40.8.12.3.1 Sway Bracing
(Use when specified on Design Layout)

| STEEL BEARING PILE | CAST-IN-PLACE PILE |

Attention Designers and Detailers:
| (1) | Omit sway bracing if less than 10'-0" (Cast-In-Place piles only). |
| (2) | When angle slope of bracing becomes less than 15° to the horizontal, omit the diagonal angles and use the horizontal angles only (Except on four pile bents, then use alternate shown above). |
Note: In case of a large number of piles, see the Structural Project Manager.
751.40.8.12.3.2 Miscellaneous Details for Prestressed Girder
PRESTRESSED GIRDERS (INTEGRAL INT. BENT)

(Continuous Spans - No Longitudinal Beam Steps)
| (*) | ¼ Joint Filler for a P/S Double Tee Structure |
| ½ Joint Filler for a P/S I-Girder Structure |
PRESTRESSED GIRDERS (NON-INTEGRAL INT. BENT)

Longitudinal Beam Step and Shear Blocks shown)
DETAILS OF CONST. JOINT KEY
|
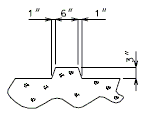
|
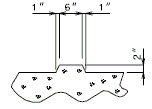
|
| PART ELEVATION | PART SECTION THRU KEYS (P/S I-GIRDERS) |
PART SECTION THRU KEYS (P/S DOUBLE TEE GIRDERS) |
751.40.8.13 Concrete Pile Cap Non-Integral End Bents
751.40.8.13.1 Design
751.40.8.13.1.1 Unit Stresses
| (1) | Reinforced Concrete | ||
| Class B Concrete (Substructure) | = 1,200 psi | = 3,000 psi | |
| Reinforcing Steel (Grade 60) | = 24,000 psi | = 60,000 psi | |
| = 10 | |||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c = W^{1.5} \times 33 \sqrt{f'_c}} AASHTO Article 8.7.1) (*) | |||
| (2) | Structural Steel | ||
| Structural Carbon Steel (ASTM A709 Grade 36) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 20,000 psi | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 36,000 psi | |
| (3) | Piling | ||
| (4) | Overstress | ||
| The allowable overstresses as specified in AASHTO Article 3.22 shall be used where applicable for Service Loads design method. | |||
| (*) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c = 57,000 \sqrt{f'c} W} = 145 pcf., Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Ec = 60,625 \sqrt{f'c}} for = 150 pcf. | ||
751.40.8.13.1.2 Loads
| (1) | Dead Loads |
| (2) | Live Load |
| As specified on the Design Layout | |
| Impact of 30% is to be used for design of the beam. No impact is to be used for design of any other portion of bent including the piles. | |
| (3) | Temperature, Wind and Frictional Loads |
| Wind and temperature forces can be calculated based on longitudinal force distribution. |
751.40.8.13.1.3 Distribution of Loads
| (1) | Dead Loads |
| Loads from stringers, girders, etc. shall be concentrated loads applied at the intersection of centerline of stringer and centerline of bearing. | |
| (2) | Live Load |
| Loads from stringers, girders, etc. shall be applied as concentrated loads at the intersection of centerline of stringer and centerline of earing. | |
| (3) | Temperature |
| The force due to expansion or contraction applied at bearing pads are not used for stability or pile bearing computations. However, the movement due to temperature should be considered in the bearing pad design and expansion device design. | |
| (4) | Wing with Detached Wing Wall |
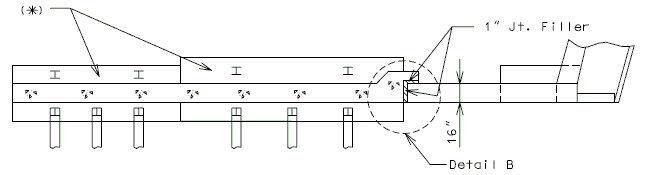
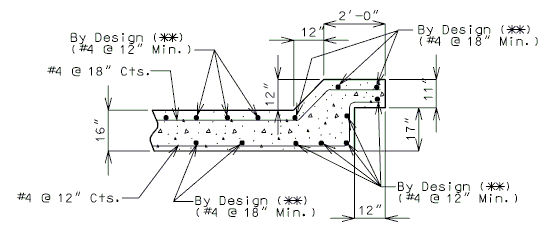
| (*) | Detached wing wall shown is for illustration purpose only. Design detached wing wall as a retaining wall. |
| (**) | See retaining wall design. |
751.40.8.13.1.4 Design Assumptions - Loadings
| 1) | Piles | ||||||||||||||||||||||
| a. | Stresses in the piles due to bending need not be considered in design calculations except for seismic design in categories B, C and D. | ||||||||||||||||||||||
| b. | The following four loading cases should be considered. | ||||||||||||||||||||||
| |||||||||||||||||||||||
| Where, | |||||||||||||||||||||||
| LL | = live load | ||||||||||||||||||||||
| DL | = dead load of superstructure, substructure and one half of the apporach slab | ||||||||||||||||||||||
| SUR | = two feet of live load surcharge | ||||||||||||||||||||||
| E | = dead load of earth fill | ||||||||||||||||||||||
| EP | = equivalent fluid pressure of earth | ||||||||||||||||||||||
| Maximum pile pressure = pile capacity | |||||||||||||||||||||||
| Minimum pile pressure = 0 (tension on a pile will not be allowed for any combination of forces exept as noted) | |||||||||||||||||||||||
| 2) | Analysis Procedure | ||||||||||||||||||||||
| a. | Find the lateral stiffness of a pile, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_\delta} : | ||||||||||||||||||||||
| With fixed pile-head (i.e., only translation movement is allowed but no rotation allowed): The lateral stiffness of a pile can be estimated using Figures 1 and 3 or 2 and 3 for pile in cohesionless or cohesive soil, respectively. The method of using Figures 1, 2, and 3 to find lateral stiffness is called Linear Subgrade Modulus Method. Usually the significant soil-pile interaction zone for pile subjected to lateral movement is confined to a depth at the upper 5 to 10 pile diameters. Therefore, simplified single layer stiffness chart shown in Figure 3 is appropriate for lateral loading. The coefficient Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f} in Figures 1 and 2 is used to define the subgrade modulus Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_s} at depth “z” representing the soil stiffness per unit pile length. For the purpose of selecting an appropriate Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f} value, the soil condition at the upper 5 pile diameters should be used. Since soil property, friction angle Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \phi} , or cohesion c, is needed when Figure 1 or 2 is used, determine soil properties based on available soil boring data. If soil boring data is not available, one can conservatively use value of 0.1 in Figure 3. Designer may also use soil properties to convert SPT N value to friction angle , or cohesion c, for granular or cohesive soil, respectively. Figures 1 and 2 were based on test data for smaller-diameter (12 inches) piles, but can be used for piles up to about 24 inches in diameter. In Figure 2, the solid line (by Lam et al. 1991) shall be used in design. | |||||||||||||||||||||||
| b. | Find the axial stiffness of a pile, : | ||||||||||||||||||||||
| For friction pile, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a} may be determined based on a secant stiffness approach as described in Bridge Manual Section 6.1 – Seismic Design or by the in-house computer program “SPREAD” where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a} is calculated as: | |||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{1}{K_a} = \frac{1}{AE / L'} + \frac{1}{K_{Q_f}} + \frac{1}{K_{Q_b}}} Equation (1) | |||||||||||||||||||||||
| Where: | |||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, A} | = cross sectional area of pile | ||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E} | = elastic modulus of pile | ||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L'} | = total length of pile | ||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_{Q_f}} | = secant stiffness due to ultimate friction capacity of the pile as described in Bridge Manual Section 6.1 | ||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_{Q_f}} | = secant stiffness due to ultimate bearing capacity of the pile as described in Bridge Manual Section 6.1 | ||||||||||||||||||||||
| For HP bearing pile on rock Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a} shall be calculated as: | |||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{1}{K_a} = \frac{1}{AE / L'} + \frac{1}{K_{Q_f}}} Equation (2) | |||||||||||||||||||||||
| Or Conservatively, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a} may be determined as: | |||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_a = \frac{AE}{L'}} Equation (3) | |||||||||||||||||||||||
| |||||||||||||||||||||||
| Recommended Coefficient Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} of Variation in Subgrade Modulus with Depth for Sand | |||||||||||||||||||||||
| |||||||||||||||||||||||
| Recommended Coefficient Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f} of Variation in Subgrade Modulus with Depth for Clay | |||||||||||||||||||||||
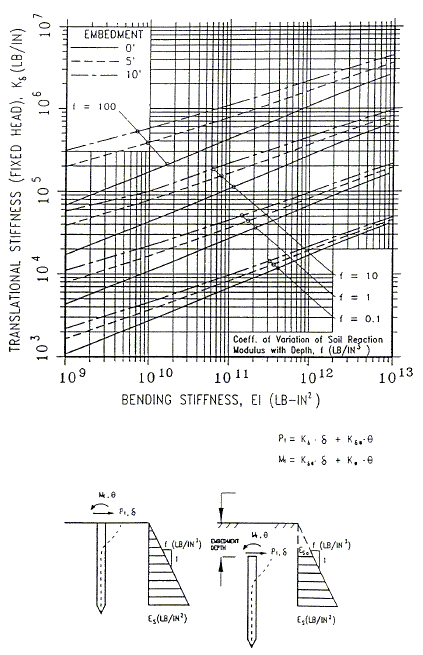
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| Lateral Embedded Pile-Head Stiffness | |||||||||||||||||||||||
| c. | Find the equivalent cantilever pile length, | ||||||||||||||||||||||
| For the structural model used in the structural analyses of loading cases I through IV. As shown in figure below, length L can be calculated as: | |||||||||||||||||||||||
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, L = \Bigg(\frac{12EI}{K_\delta}\Bigg)^{1/3}} Equation (4) | |||||||||||||||||||||||
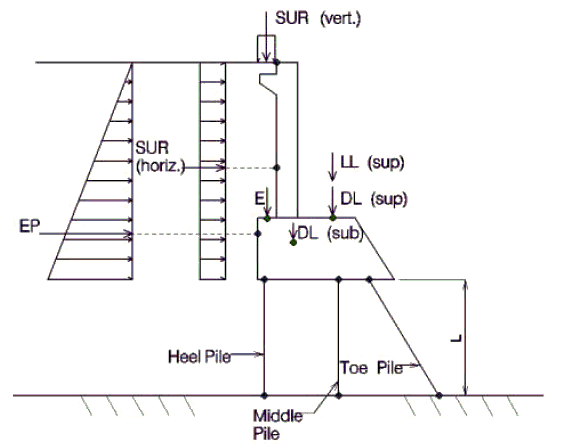
| |||||||||||||||||||||||
| Structural Model | |||||||||||||||||||||||
| d. | Find the equivalent pile area, : | ||||||||||||||||||||||
| Once the equivalent cantilever pile length has been determined from step (c) above, the equivalent axial rigidity of the pile, , can be calculated as . Then, the equivalent pile area, , is equal to | |||||||||||||||||||||||
| Equation (5) | |||||||||||||||||||||||
| e. | Perform structural analyses for loading cases I through IV. | ||||||||||||||||||||||
| Use computer programs STRUCT3D, SAP2000 or any other program capable of running static analysis. | |||||||||||||||||||||||
| f. | Check abutment movement at the top of backwall and at the bottom of beam cap | ||||||||||||||||||||||
| Maximum movement away from the backfill shall not be greater than 1/8". Maximum movement toward the backfill shall not be greater than 1/4". | |||||||||||||||||||||||
| g. | Check pile axial loads from the analysis with the allowable pile axial load capacity. | ||||||||||||||||||||||
| h. | Check overturning of bent | ||||||||||||||||||||||
| Conservatively, use the same equivalent cantilever pile length, . Check overturning of bent at the bottom of toe pile for loading cases I and II(Figure of Structural model). | |||||||||||||||||||||||
| |||||||||||||||||||||||
| 5) | Deadman Anchorage System | ||||||||||||||||||||||
| Deadman anchorage can be used when the abutment movement exceeds the allowable movement. | |||||||||||||||||||||||
| The size and location of deadman anchorage shall be designed appropriately to maintain the stability of the abutment. | |||||||||||||||||||||||
| The deadman forces may be used to resist overturning with the approval of the Structural Project Manager. | |||||||||||||||||||||||
| 6) | Passive Pressure Shear Key (if applicable) | ||||||||||||||||||||||
| Passive pressure shear key may be used when the abutment movement exceeds the allowable movement. | |||||||||||||||||||||||
| The passive resistance of soil to the lateral force at shear keys may be used with the approval of structural project manager. | |||||||||||||||||||||||
751.40.8.13.1.5 Deadman Anchors
Design Assumptions
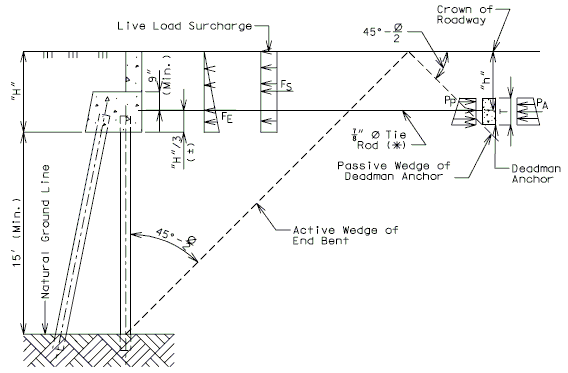
| Length of Deadman = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_E + F_S / (P_P - P_A)} | |
| Number of tie rods required = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_E + F_S) / F_R} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P_A} = Active earth pressure on deadman, in lb./ft. = (120 pcf) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_A hT} | |
| (**) | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, P_P} = Passive earth pressure on deadman, in lb./ft. = (120 pcf) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_P hT} |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_E} = Earth pressure on end bent, in lb. = 0.5(120 pcf) (length of beam) | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_S} = Surcharge on end bent, in lb. = | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, K_A = Tan^2 (45^\circ - \phi/2)} | |
| (***) | = 8.0 kips for 7/8" Ø tie rod and 10.50 kips for 1" Ø tie rods (Capacity of the tie rods based on a maximum skew of 30°.) |
| * | If the number of 7/8" Ø tie rods causes too long of a deadman, then try 1" Ø tie rods. |
| ** | For seismic loads only, use = 4 kips/sq.ft. as the ultimate capacity of compacted fill. |
| *** | For seismic loads only, the allowable stress in the tie rod may be taken as the yield stress of the rod. |
Notes:
No more than 20% of deadman may fall outside of the roadway shoulders. To prevent more than 20% limit, using a deeper deadman to reduce its length. If this is not possible, the total passive pressure resistance should be calculated by summing the resistance from the different fill depths.
When deadman anchors are to be used, design the piles for a factor of safety of 1.0 for sliding and design deadman anchors to resist all horizontal earth forces with a factor of safety of 1.0. This will result in a factor of safety for sliding of 2.0. For special cases, see the Structural Project Manager.
Design Example
| Assume: | |
| Roadway width = 36', Out-Out slab width = 36' + 2 x 16" = 38.67' | |
| Skew = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 15^\circ} , Length of Beam = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (38.67')/(Cos 15^\circ) = 40.03'} | |
| Beam depth = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 3^\prime-0^{\prime\prime} } , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \phi = 27^\circ} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, H = 8.20'} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{H}{3} = \frac{8.20'}{3} = 2.73'} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 3^\prime - 2.73^\prime = 0.27^\prime < 9^{\prime\prime}} , use Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 9^{\prime\prime}} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, h = H - (Beam\ depth) + 9^{\prime\prime} = 8.20^\prime - 3^\prime + 0.75 = 5.95^\prime} | |
| Assume Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, T = 2^\prime-0^{\prime\prime} } (Deadman anchor depth) | |
| Determine Earth and Surcharge Forces | |||
| = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Tan^2 (45^\circ - \varnothing/2) = Tan^2 (45^\circ - 27^\circ/2) = 0.3755} | ||
| = | |||
| = | |||
| = | |||
| = | |||
| = | |||
| = | |||
| = | |||
| = | |||
| = | |||
| = | |||
| = | |||
| = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (120 lb./cu.ft.)(2.6629)(5.95')(2.0')} | ||
| = | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 3,803 lbs.\,per\,foot\,of\,Deadman} | ||
| Determine number of Tie Rods required | |
| Try 7/8"Ø Rods: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, F_R = 8.0} kips | |
| Number of Rods required = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_E + F_S)/F_R = (60,642 + 29,582)/8,000 = 11.29} | |
| Use 12-7/8"Ø Rie Rods. | |
| Determine length of Deadman | |
| Length of Deadman required = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (F_E + F_S)/(P_P - P_A = {(60,642 + 29,582) lbs.}/{(3,803 - 536) lb/ft.} = 27.62'} | |
| Tie Rod spacing = minimum, ok. | |
| Length of Deadman provided = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (2'-4^{\prime\prime})(11) + 2.0^\prime = 27^\prime-8^{\prime\prime}} | |
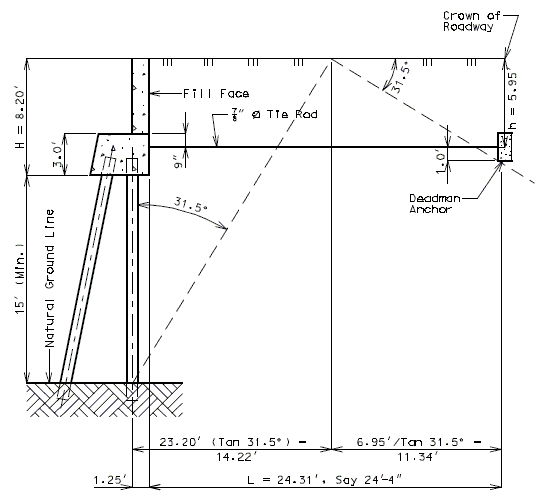
| 1) | Check tie rod skew angle at Fill Face of End Bent | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (5.5\ spacing)(30.5^{\prime\prime} - 28^{\prime\prime}) = 13.75^{\prime\prime}, tan} | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \phi = 13.75^{\prime\prime}/(24.33 \times 12^{\prime\prime}) = 0.471} | |
| , tie capacity ok. | ||
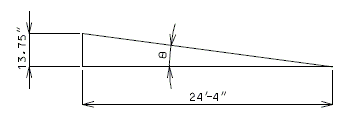
| ||
| 2) | Check criteria for Deadman Anchors extending into Fill Slope | |
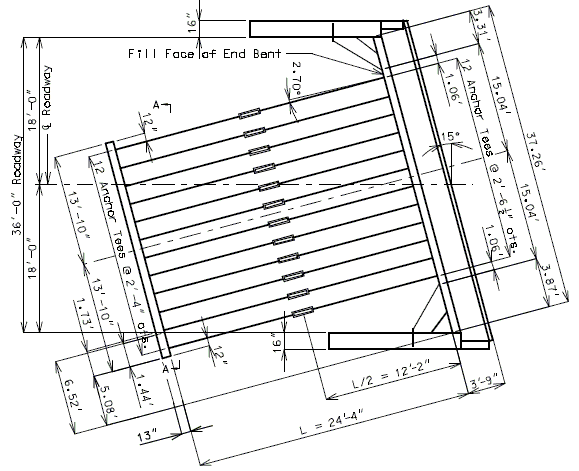
| ||
A) Extension of Deadman into Fill Slope Length of Deadman extending into Fill Slope = 0.2 (Length of Deadman) = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 0.2 (27.67^\prime) = 5.53^\prime} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 1.73^\prime < 5.53^\prime} Length of Deadman extending into Fill Slope Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, < 0.2} (Length of Deadman), ok Note: See below for Section A-A details. B) Cover of Deadman in Fill Slope
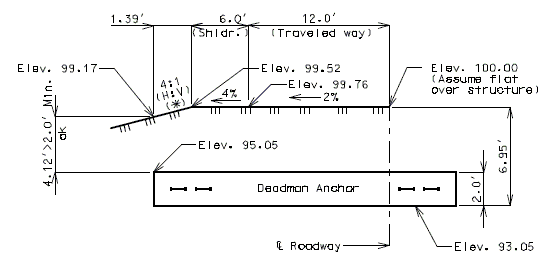
DETAIL AT FILL SLOPE
Note:
(*) Fill slope shown is for illustration purpose only, see roadway plans.
751.40.8.13.2 Reinforcement
751.40.8.13.2.1 Wide Flange Beams, Plate Girders and Prestressed Girders
END BENT WITH EXPANSION DEVICE

|
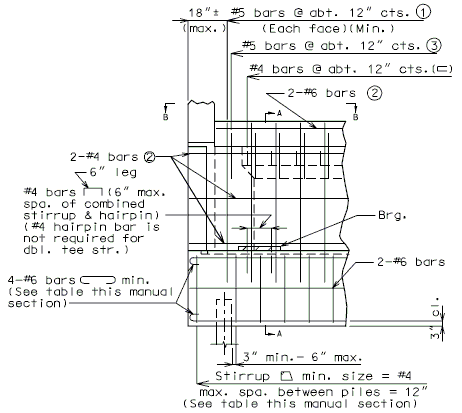
|
| SECTION A-A | |
| PART ELEVATION |
Notes:
(1) See details for reinforcement of end bent backwall.
(2) #6-H bars and #4-H bars in backwall of skewed bridges shall be bent in field if required.
(3) Center #5 bars in backwall.
Epoxy coat all reinforcing in end bents with expansion devices. See ______ for details of protective coating and sloping top of beam to drain.
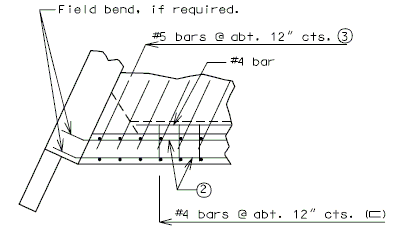
|
|
| DETAIL OF #5 BARS SHAPE 19 | |
| PART PLAN B-B |
END BENT WITHOUT EXPANSION DEVICE
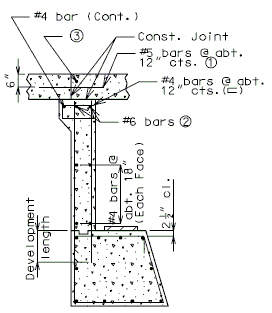
|
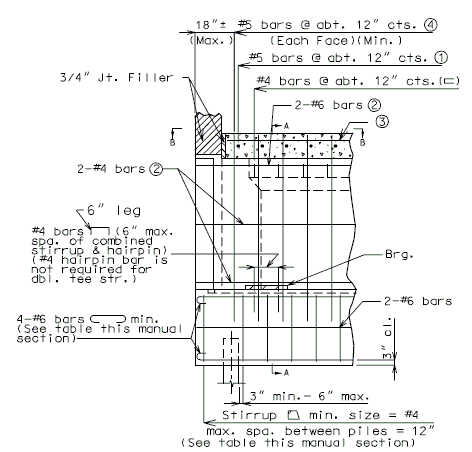
|
| SECTION A-A | |
| PART ELEVATION |
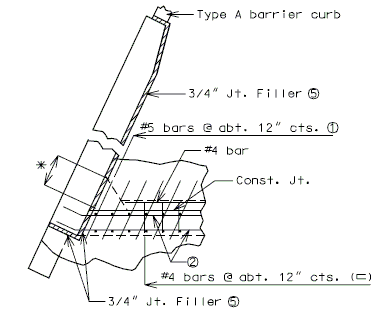
|
| ||||||||||||
| PART PLAN B-B | |||||||||||||
END BENT WING
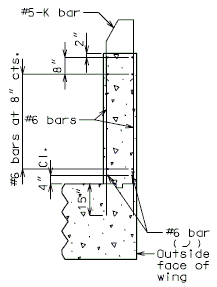 | |
|
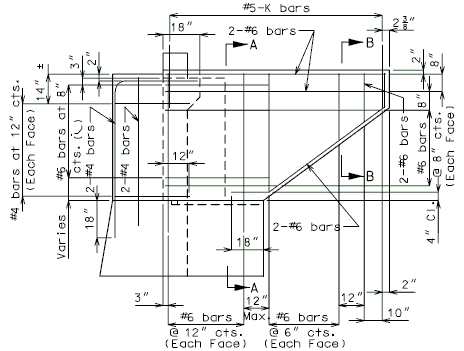
|
| SECTION A-A | |
| TYPICAL ELEVATION OF WING |
Note: (1) Development length
|
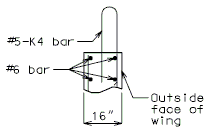
| ||||||||||||
| SECTION B-B | |||||||||||||

|
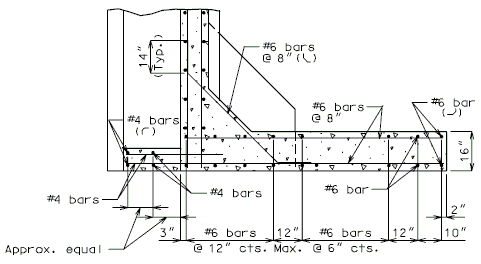
| ||||||||||||
| PART SECTION THRU BENTS WITH PASSIVE PRESSURE | |||||||||||||
| HORIZONTAL SECTION THRU WING (K bars not shown for clarity) |
END BENT BEAM HEEL
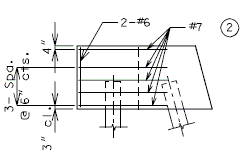
|
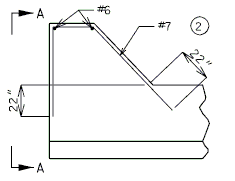
|
| ELEVATION A-A (TYP.) | PART PLAN OF BEAM (SQUARE) |
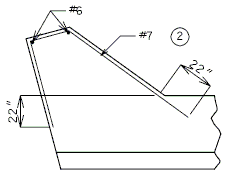
|
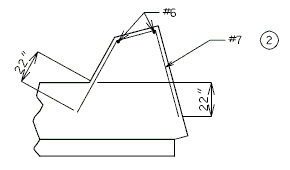
|
| PART PLAN OF BEAM - SKEWS THRU 15° - LEFT ADVANCE SHOWN | |
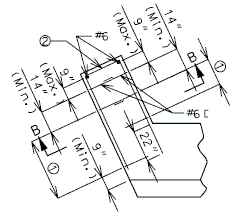
|
|
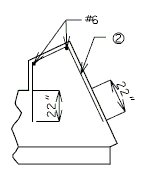
|
| SECTION B-B | ||
| PART PLAN OF BEAM - SKEWS OVER 15° - LEFT ADVANCE SHOWN | ||
Note:
Vertical spacing for #7 bars shown in Elevation A-A is typical for all types of end bent beams.
For a long distance between heel pile and bearing beam investigate for use of larger bars; e.g. larger skews where the shear line does not fall within the bearing beam.
| Pile Load Not Greater | (1) Hair-Pin Stirrups | (2) Horizontal Rebar around Heel Pile | |||
| Skew thru 30° | Skew 31° thru 45° | Skew 46° thru 60° | Skew over 60° | ||
| 140 kips | #6 @ 9" | 5-#7 | 5-#7 | 5-#8 | By Design |
| 194 kips | #6 @ 6" | 5-#7 | 5-#8 | By Design | By Design |
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle *}
Use 21" horizontal leg.
END BENT BACKWALL
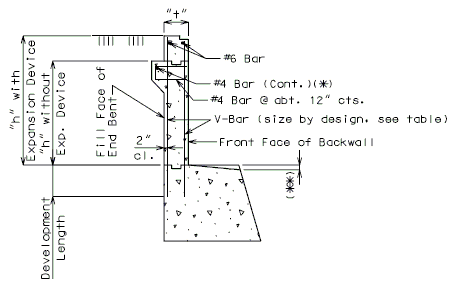
| V-BAR SIZE AND SPACING | |||
| h (feet) |
t (inch) |
Fill Face Reinforcement |
Front Face Reinforcement |
| 1-6 | 12 | #5 @ 12" | #5 @ 12" |
| 7 | 12 | #5 @ 12" | #5 @ 12" |
| 8 | 12 | #5 @ 12" | #5 @ 12" |
| 9 | 12 | #6 @ 12" | #5 @ 12" |
| 10 | 12 | #6 @ 10" | #5 @ 12" |
| 11 | 15 | #6 @ 10" | #5 @ 12" |
| 12 | 15 | #6 @ 8" | #5 @ 12" |
| 13 | 18 | #6 @ 8" | #5 @ 12" |
| 14 | 18 | #6 @ 6" | #5 @ 12" |
Note:
All reinforcement is grade 60.
Design is based on 45 lbs. per cu. ft. equivalent fluid pressure and 90 lbs. per sq. ft. live load surcharge.
Epoxy coat all reinforcing steel in beam and backwall on non-integral end bents with expansion devices.
751.40.8.14 Concrete Pile Cap Integral End Bents
751.40.8.14.1 Design
751.40.8.14.1.1 Design Unit Stresses
- Reinforced Concrete
- Class B Concrete (Substructure) = 1,200 psi, = 3,000 psi
- Reinforcing Steel (Grade 60) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 24,000 psi Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 60,000 psi
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, n} = 10
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c} =Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, w^{1.5} \times 33 \sqrt{f'_c}} (AASHTO Article 8.7.1)(*)
- Structural Steel
- Structural Carbon Steel (ASTM A709 Grade 36) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_s} = 20,000 psi Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f_y} = 36,000 psi
- Piling
- See the Design Layout if pile capacity is indicated.
- Overstress
- The allowable overstresses as specified in AASHTO Article 3.22 shall be used where applicable for Service Loads design method.
(*) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, E_c = 57,000 \sqrt {f'_c} for\ W = 145 pcf,\ E_c = 60,625 \sqrt{f'_c} for W = 150 pcf }
751.40.8.14.1.2 Loads
- Dead Loads
- Live Load
- As specified on the Design Layout.
- Impact of 30% is to be used for design of the beam. No impact is to be used for design of any other portion of bent including the piles.
- Temperature, Wind and Frictional Loads
751.40.8.14.1.3 Distribution of Loads
- Dead Loads
- Loads from stringers, girders, etc. shall be concentrated loads applied at the intersection of centerline of stringer and centerline of bearing. Loads from concrete slab spans shall be applied as uniformly, distributed loads along the centerline of bearing.
- Live Load
- Loads from stringers, girders, etc. shall be applied as concentrated loads at the intersection of centerline of stringer and centerline of bearing. For concrete slab spans distribute two wheel lines over 10'-0" (normal to centerline of roadway) of substructure beam. This distribution shall be positioned on the beam on the same basis as used for wheel lines in Traffic Lanes for Substructure Design.
- Wing with Detached Wing Wall
- When wing length, L, is greater than 17'-0", use maximum length of 10'-0" rectangular wing wall combined with a detached wing wall. When detached wing walls are used, no portion of the bridge live load shall be assumed distributed to the detached wing walls. Design detached wing wall as a retaining wall. (The weight of Safety Barrier Curb on top of the wall shall be included in Dead Load.)
751.40.8.14.1.4 Design Examples
Design H-bar and F-bar of an intermediate wing as shown in the figures below (wing length = 12.5', wing thickness = 24", wing height = 8'-4"), a Seismic Force of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \omega} = 12.21 kips/ft. is applied on the wall.
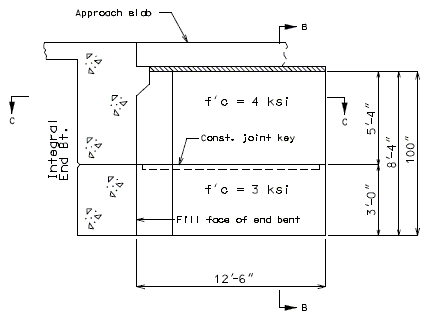
|
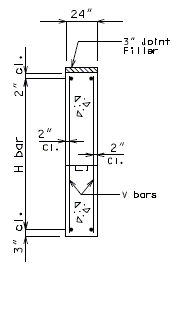
|
| Section Near Intermediate Wing | Section B-B |
|---|---|
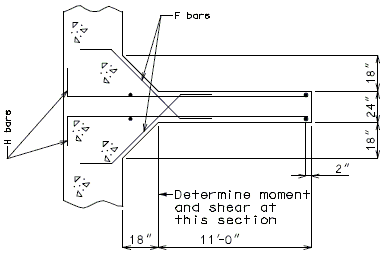
|

|
| Interior Wing Design | |
| Section C-C | |
Solve: Assume #6 V bar, #8 H bar, #6 F bar
| 1.) | Design H-bar for bending |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, d = 24in. - 2in. (clr.) - 0.75in. (V\ Bar) - 0.5 \times 1in. (H\ bar) = 20.75in.} . | |
| At Section A-A: | |
| Use Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, f-c = 3 kisi,} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, fy = 60 ksi} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, m = fy/(0.85 f'c) = 60/(0.85 \times 3) = 23.53} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \rho = (1/m)[1 - \sqrt{1 - 2 Rum/fy}] = (1 - \sqrt{1 - 2 \times 228.85 \times 23.53/60000})/23.53 = 0.004003} | |
| As (Req'd) = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \rho\ bd = 0.004003 \times 100in. \times 20.75in. = 8.31 sq.\ in.} | |
| Try No. 8 @ 9", USE Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \frac{100in - 3in. (clr.) - 2in. (clr.) - 1in (No.\ 8\ bar)}{9in} = 10.44\ spacing} | |
| Say 11 spacings, 12 bars (Each Face) | |
| Total Area = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, 12 (0.7854) = 9.42 sq.\ in. > 8.31 sq.\ in.,} USE 12-No. 8 H-bar (Each Face) |
| 2.) | Design F-bar for shear |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Vu \le \phi (Vc = Vs),\ \phi = 0.85} (AASHTO Article 8.16.6.1.1) | |
| At Section A-A: | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Vu = 1.0 \times (\omega \ell) = (12.21 kips/ft.)(11ft.) = 134.11 kips} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Vc = bd(\vartheta c) = bd(2 \sqrt{f'c} = (100in. \times 20.75in.)(2 \times \sqrt{3000})/1000 = 227.30 kips} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \phi\ Vc = 0.85 Vc = 0.85 \times 227.30 kips = 193.20 kips} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, \phi\ Vc = 193.20 kips > Vu = 134.11 kips,} No Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Vs} needed by AASHTO Article 8.16.6.3.1. | |
| Minimum shear reinforcement is required by AASHTO Article 8.19.1.1(a).(ACI 318-95 11.5.5.1) | |
| F-bar is a single group of parallel bars, all bent up at the same distance from support (no "spacing" along the "L" direction of the wing). | |
| Try No. 6 @ 12" F-bar (each face). | |
| Try Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, (100in. - 3in. - 2in. - 1in. )/12in = 7.83,} say 8 spacing, 9 bars (each face). | |
| Since seismic force is a cyclic loading, assume one bar works at any instance. | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Av (provided) = 1 \times 9 \times (0.4418 sq.\ in.) = 3.98 sq.\ in.} | |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \, Vs = Av (Fy\ Sin 45^\circ) = (3.98 sq.\ in.)(60 ksi)(Sin 45^\circ) = 168.7 kips} | |
| Check | |
| O.K. by AASHTO Article 8.16.6.3.4. | |
| USE 9 No. 6 F-bars (each face). |
751.40.8.14.2 Reinforcement
751.40.8.14.2.1 Earthquake Loads at End Bent – Intermediate Wing (Seismic Shear Wall)
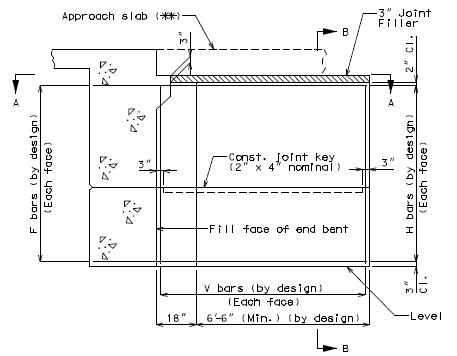
|
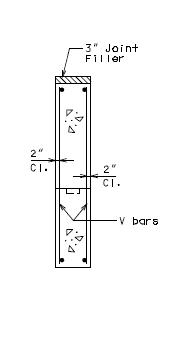
|
| Section Near Intermediate Wing | Section B-B |
|---|---|
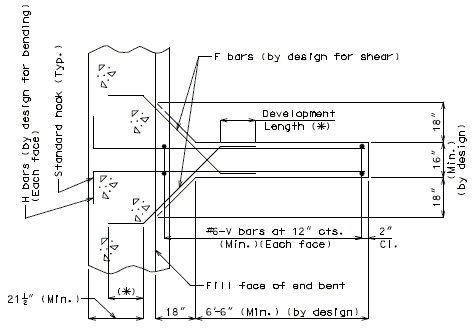
|
|
| Section A-A | |
| * | Use 1.25 x development length for seismic design. |
| ** | Additional reinforcing steel by design if required. |
| Note: | Make sure reinforcement does not interfere with girders. |
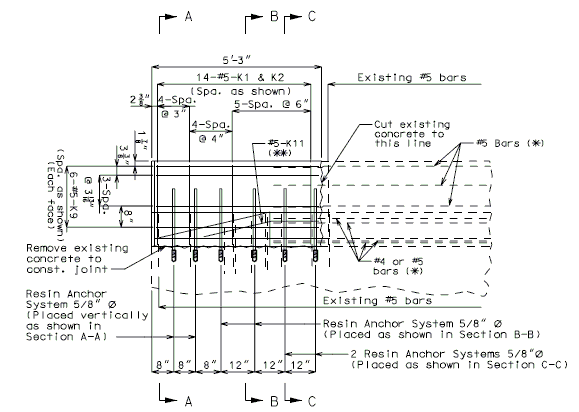
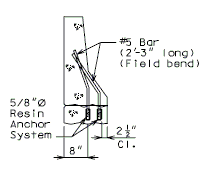









![{\displaystyle \,=1.3[\beta _{d}D+L+I+T]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d753b0f224f7b28cab71279d95e33d0cfec289d4)














































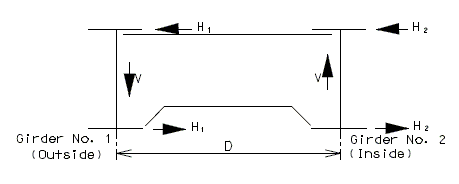






















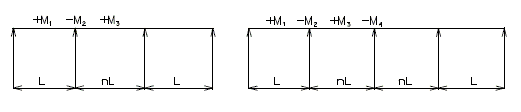



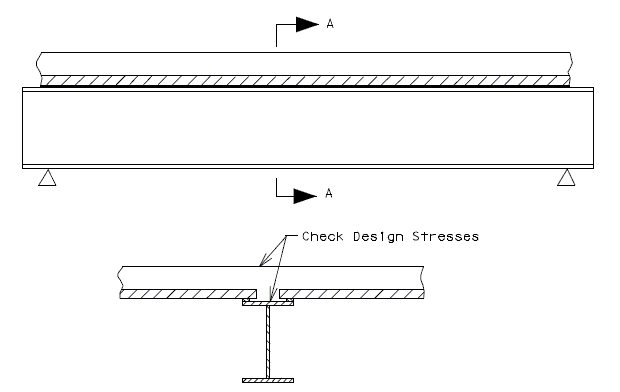
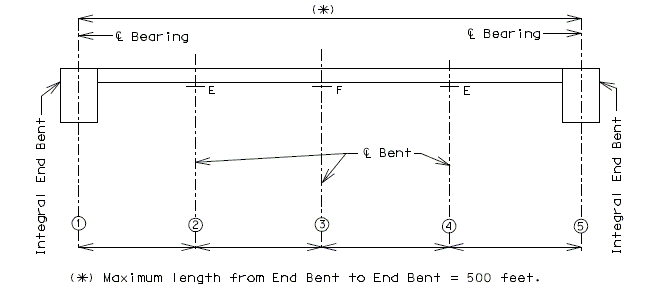



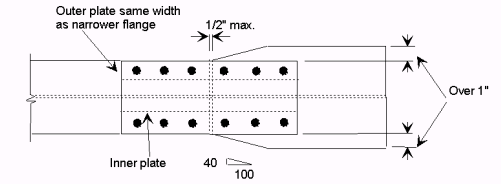























































![{\displaystyle \,\uparrow \triangle _{1}=144\times 10^{3}\times {\underset {(a={\big [}L-(centerline\ to\ centerline\ tie\ downs){\big ]}\div 2)ft.}{{\Bigg [}{\frac {F_{01}(e_{1})(L_{2}}{8E_{i}I}}+{\frac {F_{02}(e_{2}+e_{3}}{E_{i}I}}{\Bigg (}{\frac {L_{2}}{8}}-{\frac {a^{2}}{6}}{\Bigg )}-{\frac {F_{02}(e_{3}(L^{2})}{8E_{i}I}}{\Bigg ]}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d5b0b90ebeff8233eef007c81cf329d143049570)