751.22 Prestressed Concrete I Girders

Video

Concrete Girder

751.22.1 General

751.22.1.1 Material Properties

Concrete

Concrete strength utilized for prestressed girders may be conventional or high strength concrete (HSC) which shall be identified on the girder plans. HSC shall be concrete strengths in excess of 8.0 ksi and may only be used with the permission of the Structural Project Manager or Structural Liaison Engineer. Costs may increase due to production modifications necessary to obtain the required HSC strength.

Conventional concrete strength for P/S I-Girder shall be the following:

For MoDOT Standard Girders:

$\,f'_{ci}=4.5ksi$, $\,f'_{c}=6.0ksi$

Optional higher concrete strength shall be:

$\,f'_{ci}=5.0ksi$, $\,f'_{c}=7.0ksi$

OR

With the approval of the Structural Project Manager or Liaison:

$\,f'_{ci}=6.5ksi$, $\,f'_{c}=8.0ksi$

For NU Standard Girder:

$\,f'_{ci}=6.5ksi$, $\,f'_{c}=8.0ksi$

Modulus of Elasticity, $\,E_{c}=33,000\ K_{1}\ (w_{c}^{1.5}){\sqrt {f_{c}^{'}}}$

Where,

f'_c in ksi

K₁ = correction factor for source of aggregate

= 1.0 unless determined by physical testing

$w_{c}=0.145kcf\ {\mbox{for}}\ f'_{c}\leq 5.0ksi$

$w_{c}=0.140+0.001f'_{c}\ {\mbox{for}}\ f'_{c}>5.0ksi$

Prestressing strand
Type of strand:
AASHTO M203 (ASTM A416) Grade 270
Uncoated, seven-wire, low-relaxation strand
Ultimate tensile strength,		$\,f_{pu}=270ksi$
Yield strength,		$\,f_{py}=0.9f_{pu}ksi$
Strand modulus of elasticity,		$\,E_{p}=28500ksi$
For conventional concrete strengths:
	Strand diameter,	$\,d_{ps}=0.5in$
	Strand area,	$\,A_{ps}=0.153in^{2}$
OR:
	Strand diameter,	$\,d_{ps}=0.6in$
	Strand area,	$\,A_{ps}=0.217in^{2}$
Order of Material Use for Increasing Girder Capacity in Order of Increasing Costs
1. Increase concrete strength (readily producible by fabricator)
2. Increase strand size (readily available from fabricator but steel costs are high)
3. Modify MoDOT shape (most costly and inconvenient becasue of forming bed modifications required) (except NU shape)
Mild reinforcing steel
Minimum yield strength,		$\,f_{y}=60.0ksi$
Steel modulus of elasticity,		$\,E_{s}=29000ksi$
Welded Wire Reinforcement
Minimum yield strength,		$\,f_{y}=70.0ksi$
Steel modulus of elasticity,		$\,E_{s}=29000ksi$

751.22.1.2 Geometric Dimensions

The ratio of the depth of girder to span length will in general be not less than 1/18.

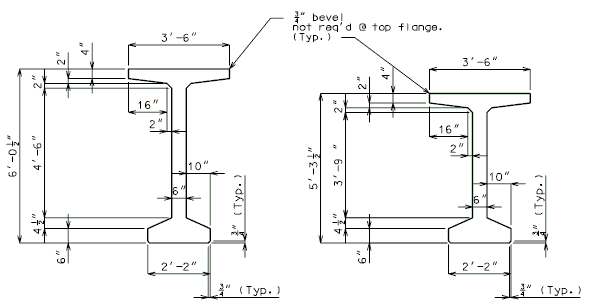
The cross sectional dimensions of the girder shall be one of the following:

MoDOT Standard Girders:

BEAM TYPE 2

BEAM TYPE 3

BEAM TYPE 4

BEAM TYPE 6

BEAM TYPE 7

BEAM TYPE 8

$*$ If the web is required to be increased, then the top and bottom flanges are to be increased by the same amount. (1" increments 2" max.).

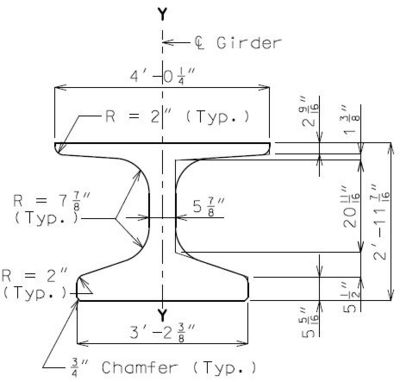
NU Standard Girders:

BEAM NU 35 A = 639.79 in.² Y_b = 15.96 in. Y_t = 19.48 in. I_xx = 108,498 in.⁴ I_yy = 60,086 in.⁴ V/S = 3.067 in.

BEAM NU 43	BEAM NU 53
A = 686.05 in.2	A = 743.88 in.2
Yb = 19.36 in.	Yb = 23.71 in.
Yt = 23.95 in.	Yt = 29.44 in.
Ixx = 179,343 in.4	Ixx = 297,512 in.4
Iyy = 60,219 in.4	Iyy = 60,385 in.4
V/S = 3.058 in.	V/S = 3.048 in.

BEAM NU 63	BEAM NU 70
A = 801.72 in.2	A = 847.98 in.2
Yb = 28.14 in.	Yb = 31.74 in.
Yt = 34.86 in.	Yt = 39.14 in.
Ixx = 451,306 in.4	Ixx = 601,931 in.4
Iyy = 60,551 in.4	Iyy = 60,684 in.4
V/S = 3.040 in.	V/S = 3.034 in.

751.22.1.3 Typical Span Ranges

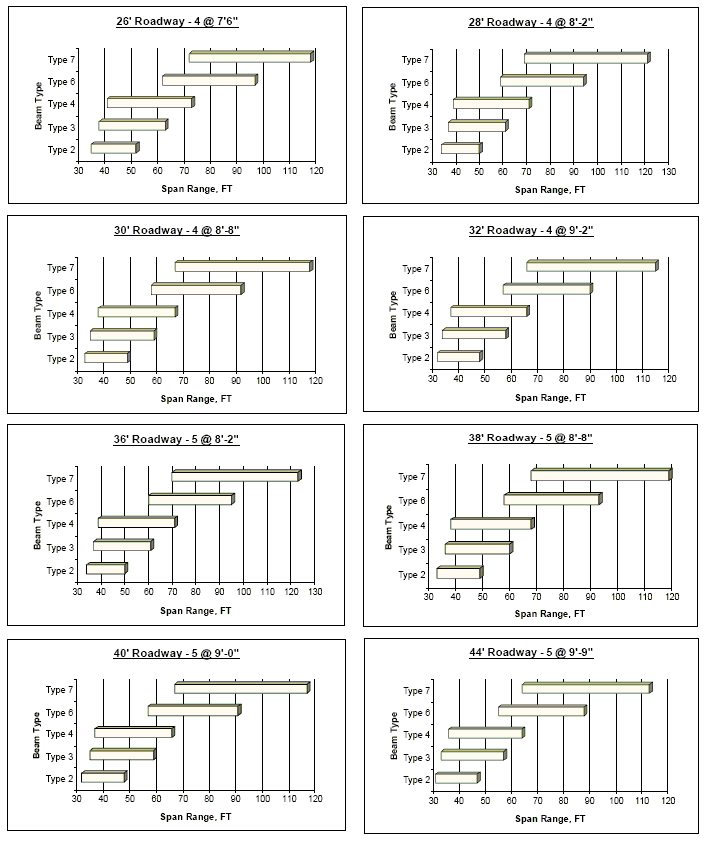
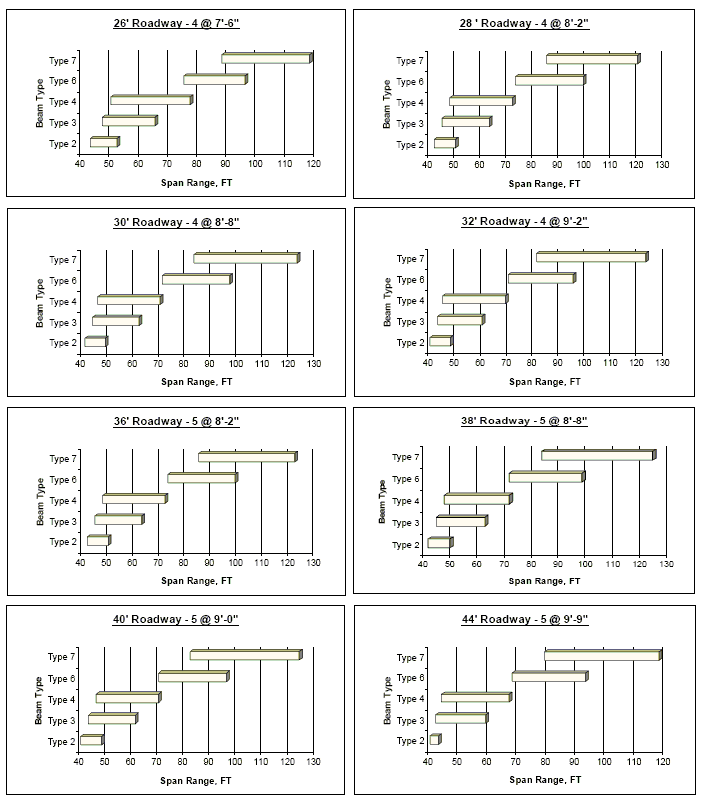
The following charts provide span ranges (limits) for P/S I-girders based on girder spacing and standard roadway widths.

Limitations of the Charts:

A. Standard Concrete Charts Only

Criteria used in determining maximum span lengths for lower conventional concrete strength:

1) Low-relaxation strand with 0.5” strand diameter

2) Concrete strengths, $\,f'_{ci}$ = 4.5 ksi and $\,f'_{c}$ = 6.0 ksi

3) 3-span bridge consisting of 3 equal length girders made continuous and composite

B. Optional Concrete Charts Only

Criteria used in determining maximum span lengths for greater conventional concrete strength:

1) Low-relaxation strand with 0.6” strand diameter

2) Concrete strengths, $\,f'_{ci}$ = 5.0 ksi and $\,f'_{c}$ = 7.0 ksi

3) 3-span bridge consisting of 3 equal length girders made continuous and composite

C. Both Standard Concrete and Optional Concrete Charts

Criteria used in determining span ranges for both Standard and Optional Concrete conventional strengths.

1) Minimum span lengths were determined by the positive moment capacity of the smallest strand arrangement per beam shape. Shorter span lengths are possible.

2) Based on 10 ft. design lanes. (Current design practice meets AASHTO LRFD and uses 12 ft. design lanes.)

3) Based on unrefined prestress loss equations. (Current design practice meets AASHTO LRFD and uses refined losses.)

Recommended Adjustments for Using the Charts:

Because the span limit charts were developed using older design criteria as noted above, increased span lengths are probable.

1) Span limits given in all charts should be increased 10 percent to account for current design practice. Ten percent can safely be used without a preliminary girder analysis.

2) Span limits given in all charts shall be increased when a preliminary girder analysis based on actual design conditions is performed which shall be noted on the Design Layout.

Span range charts are planned for future replacement. Use the recommended adjustments until implemented.

Standard Concrete ($\,f'_{c}$ = 6 ksi) P/S I Beam Span Ranges for
Given Roadway Widths and Girder Spacing

Optional Concrete ($\,f'_{c}$ = 7 ksi) P/S I Beam Span Ranges for
Given Roadway Widths and Girder Spacing

751.22.1.4 Span and Structure Lengths

Girder Length and Geometric Layout

Tangent Bridges

Girder lengths of exterior spans (i.e., end spans) shall be computed using the requirements shown below.

Girder lengths of interior spans shall be computed using the requirements shown below.

Curved Bridges

Layout of any curved structure may be done using any coordinate geometry programs available. To layout the bridge, use the following steps:

1. Start out by laying in the centerline (CL) of the survey curve.
2. Locate the tie point of the bridge. This point will usually be on the CL of the survey curve but may be on a baseline which is offset a certain distance to the CL of the survey curve.
3. A second tie point may be required if the skew is not measured to the CL of roadway at the bridge tie point. If this is the case, establish the tie point at the specified station and plot the skew line at the required angle.
4. Next, on the centerline of structure or baseline curve, locate the station of the CL of bent for each intermediate bent and the fill face for the end bents. Once these points are located, plot lines through these stations parallel to skew line. Normally the layout file will specify that all bents are parallel to the skew line; however, there may be times when the bents are radial or have varying skews.
5. When locating the stations in the preceding step, the distance between CL of intermediate bents are exactly the layout lengths specified on the file. However, the end spans need to follow the procedure for calculating length set forth in this section.
6. When the CL of the intermediate bents and the fill face lines have been added, chords should be drawn connecting these points sequentially. For example, if you have a three span bridge, chords should be drawn from the fill face of bent 1 to CL of bent 2, CL bent 2 to CL bent 3, and CL bent 3 to fill face bent 4.
7. When all the chords are in, offset each girder in each span parallel to this chord. The perpendicular distance between girders will be the same for all spans, but the skew distance between girders along the bent will vary from bent to bent depending on the skew to the CL at that point. The designer needs to be aware of the fact that at an intermediate bent the distance between bearings on the approaching and leaving span sides will be different distances. These bearings will not line up across the bent and will actually diverge more the farther away they are from the CL of the survey.
8. When establishing the CL of bearing points, the designer needs to allow for a minimum of seven (7) inches between ends of girders at the bents while keeping in mind that the girders will be offset and at different skews. If the offset is greater than half the girder bottom flange width, see Structural Project Manager. The distance from the end of girder to CL of bearing point should be half of the bearing length plus one inch minimum clearance. Once the distance for CL bent to CL of bearing is calculated, the designer should offset lines by that dimension on either side of the CL of bent. These lines will then be intersected with each of the girder lines to create the bearing points on each bent.
9. Between the bearing points at the ends of the girders, quarter points or tenth points need to be established, depending on the girder span. These points will be used in calculating the haunch and bottom of slab elevations for the bridge deck.
10. The bridge deck and barrier curbs can be laid in by offsetting the CL of roadway to each side by the proper distance. Curves should be laid in to designate both the inside and outside edges of the barrier curb. These will later be useful in laying in the wings and end bents.
11. After the outside edge of slab curves are plotted, the curve offsets need to be found. The intersection point of the outside edge of slab and the CL of each bent or fill face can be connected with chords. The distance between these chords and their partner curves need to be calculated at five foot intervals beginning at the center point of each chord.
12. Joints are placed in the barrier curb at each bent. These joints are placed perpendicular to the CL of the roadway through the intersection point of the CL bent and the inside of barrier curb.
13. Wing layout length is given on the profile sheets in the layout file. An arc should be struck so as to intersect the inside of barrier curb the specified length from a point at the intersection of the fill face and the inside of barrier curb. This point will mark the end of the wing which is perpendicular to the CL of the roadway.

The vertical curve information needs to be added so a program can calculate the elevations at the desired stations. After this is done, the designer can request any of the following information which will be needed:

• Stations and elevations of all points
• Offset distances to the chords
• Lengths of girders
• Distances between bearings
• Angles between girders and each bent
• Lengths of bents
• Lengths of barrier curbs between joints
• Minimum vertical clearance

INTEGRAL END BENTS

NON-INTEGRAL END BENTS

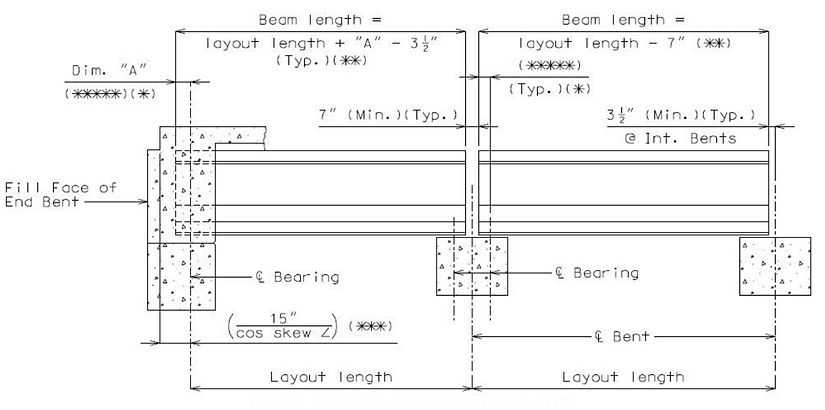
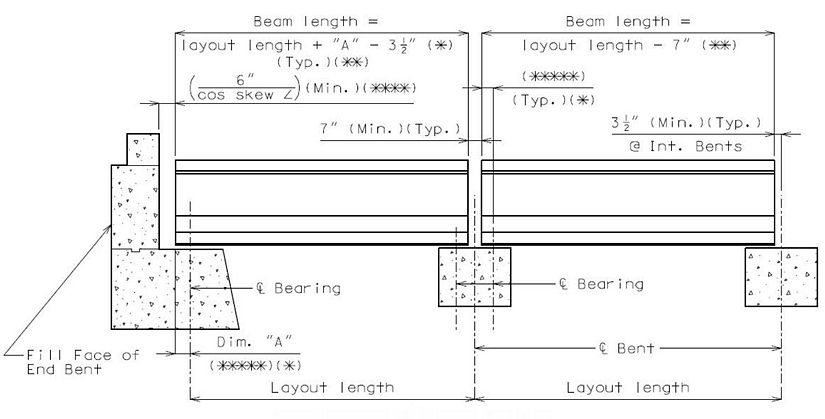
Note: Layout length for single span shall be measured from centerline of bearing to centerline of bearing. If the difference between layout length of the end span and interior span is within 1 ft., then layout length should be adjusted if possible so the girder lengths are equal for end span and interior span.

(*) Minimum dimension from edge of bearing pad to end of girder equals one inch.

(**) Design layout lengths are horizontal lengths. Girder lengths should be adjusted according to grade and shall be specified to the nearest 1/8 inch.

(***) For large skews, end bent beam caps may need to be larger to provide edge distance.

(****) Horizontal distance along certerline of girder.

(*****) = 1” (minimum) + ½ bearing pad length which equals:

5” (minimum) for MoDOT Standard Girders and Adjacent P/S Box Beams,

3 ½” (minimum) for NU Girders and P/S Spread Box Beams.

PART PLAN SHOWING COPING DETAIL (MoDOT Standard Girders and NU Girders)

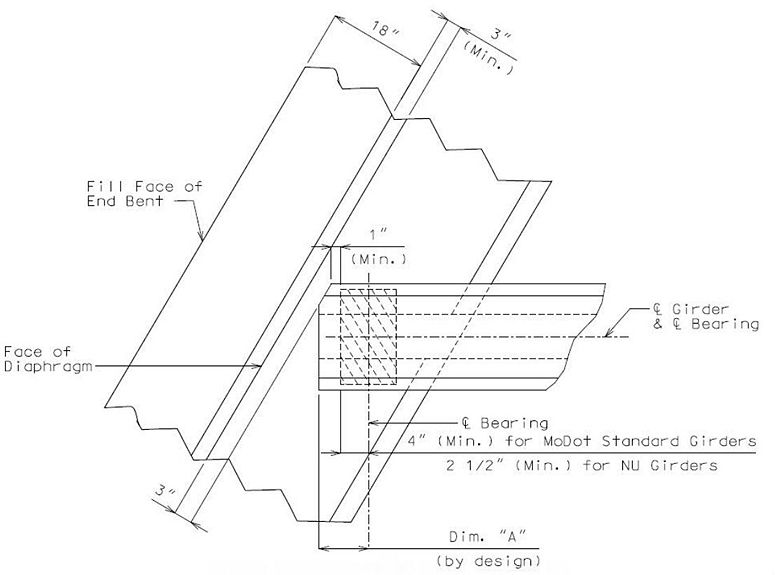
Note: Non-Integral end bents with skews greater than 40° shall always have girder ends coped. Skews less than 40° shall have girder ends coped on case by case basis. It is preferable to not cope across the web.

Check clearance from fill face of integral end bents to bottom flanges of NU standard girders. Maintain 3” (min.) clearance. Coping may be permitted with approval of the Structural Project Manager or Structural Liaison Engineer.

(*) Maximum length from End Bent to End Bent = 600 feet. TYPICAL CONTINUOUS PRESTRESSED STRUCTURE
(INTEGRAL END BENTS)

(**) Maximum length from End Bent to End Bent = 800 feet. TYPICAL CONTINUOUS PRESTRESSED STRUCTURE
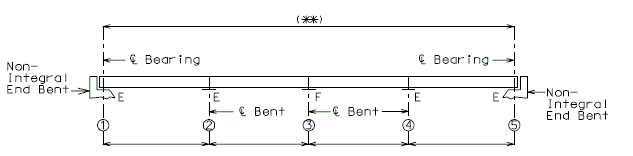
(NON-INTEGRAL END BENTS)

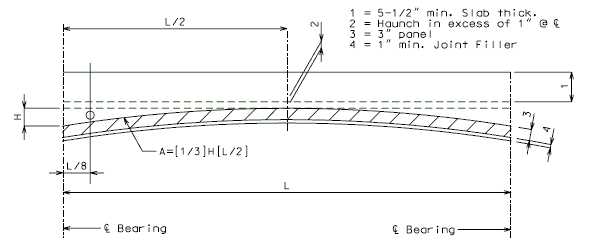
751.22.1.5 Constant and Varied Joint Filler Loads

Varied joint filler load

The prestressed I-girder should first be designed assuming that the contractor will vary the joint filler supporting the panels on the girder flange. This assumption will maintain the minimum slab/panel combination thickness of 8 1/2”, and will eliminate the possibility of increased load due to varying slab thickness.

Constant joint filler load

With the girder designed and the camber and haunching dimensions calculated, the girder should be checked assuming the contractor will use a constant 1” joint filler. This will cause the slab thickness to vary due to camber of the girder, increasing load. This additional load shall be placed as a concentrated load at 1/8 point from each end of the girder.

An example of how this concentrated load could be calculated is shown as follows:

Load $\,w=(A)(0.15kips/ft.^{3})$

Determine the concentrated load* to girders by distributing w transversely across the girders. If the minimum haunch is greater than 1” joint filler, the additional haunch shall be included in the slab thickness as a uniform load. If the use of these loads causes the girder design to change, it shall be the responsibility of the designer to determine if the camber and haunching should be recalculated.

$*$This load shall be positioned at the 1/8 point from centerline of bearing pad.

The girder and bearing designs should be checked for the constant joint filler option and constant joint filler load. However, camber, haunching and beam seat elevations shown on the plans should be based on the variable joint filler option.

JOINT FILLER LOADS

751.22.2 Design

751.22.2.1 Load Combinations

In general, each component shall satisfy the following equation:

$\,Q=\textstyle \sum$$\,\eta _{i}\gamma _{i}Q_{i}\leq \phi R_{n}=R_{r}$

Where:

$\,Q$	= Total factored force effect
$\,Q_{i}$	= Force effect
$\,\eta _{i}$	= Load modifier
$\,\gamma _{i}$	= Load factor
$\,\phi$	= Resistance factor
$\,R_{n}$	= Nominal resistance
$\,R_{r}$	= Factored resistance

Limit States

The following limit states shall be considered for P/S Girder design:

SERVICE I - for compressive stress

SERVICE III - for tensile stress

STRENGTH I

See LRFD Table 3.4.1-1 for Loads and Load Factors applied at each given limit state.

Resistance factors, $\,\phi$

STRENGTH limit states, see LRFD Article 6.5.4.2 & 5.5.4.2.1
For all other limit states, $\,\phi$ = 1.00

See EPG 751.2.3.1 Load Modifiers.

751.22.2.2 Prestressing Strands

Transfer Length of Prestressing Strands

The prestressing force may be assumed to vary linearly from zero at the point where bonding commences to a maximum at the transfer length. The transfer length may be taken as 60 times the strand diameter.

Development Length of Prestressing Strands

The development length for prestressing strands shall be taken as:

$\,l_{d}\geq 1.6{\Bigg (}f_{ps}-{\frac {2}{3}}f_{pe}{\Bigg )}d_{ps}$

Where: $\,d_{ps}$ = Nominal diameter of strand, (in.) $\,f_{ps}$ = Average stress in prestressing strand at the time for which the nominal resistance of the girder is required, (ksi)

Stress limits for prestressing strands

Strand stress at service limit state shall not exceed the following:

At jacking:

$\,f_{pj}\leq 0.75f_{pu}$ ksi

(For typical girders and fabrication economy, $\,f_{pj}=0.75f_{pu}$)

At service limit state after all losses:

$\,f_{pe}\leq 0.80f_{py}$ ksi

Where:

$\,f_{pj}$	= Stress in prestressing strand at jacking, (ksi)
$\,f_{pe}$	= Effective stress of strand after all losses, (ksi)
$\,f_{py}$	= Yield strength of strand, (ksi)
$\,f_{pu}$	= Ultimate tensile strength of strand, (ksi)

Prestress Losses

Refined estimates of time-dependent losses are used, based on AASHTO LRFD Article 5.9.5.4, as opposed to approximate lump sum estimate of losses in AASHTO LRFD Article 5.9.5.3.

The prestress losses shall be calculated to investigate concrete stresses at two different stages.

1. Temporary stresses immediately after transfer:
2. Final stresses

SERVICE I and SERVICE III Limit states shall be investigated at each stage.

Harped Strands

Harped strands, although they add to the shear strength of the girder, are primarily used to keep the girder stresses (both top and bottom) within allowable limits while developing the full capacity of the girder at midspan.

Harped strands should be held down at points of 0.4 of the distance from each end of the girder.

Example Harped Strand Layout

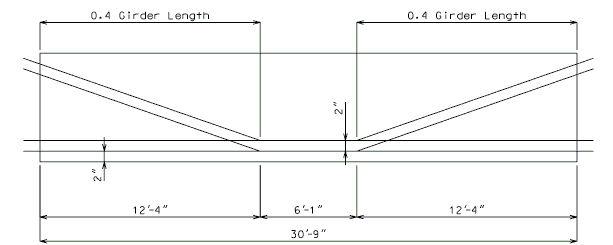
The jacking force applied to prestress strands produces an excessive vertical uplift in short spans on tall girders resulting in failure of harped strand hold-downs. The allowable limits for hold-downs are as follows:

1. 5 kip/strand
2. 10 kip/bolt
3. 42 kip/hold-down

Hold-Down Device

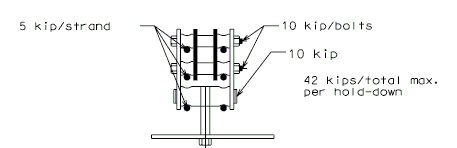
If necessary lower harped strand end location to meet criteria or use straight strands only. Investigate the possibility of using all straight strands when strength check of a hold-down device exceeds allowable.

Straight Strands.

Short spans (<40 ft.) are to use straight strands only for all girders greater than 2'-8" tall. Use at least two straight strands at the top of the girder when straight strands are used. Where straight strands only will not work a single hold-down point may be used. Note: A single point hold-down has twice the uplift force.

Strand Arrangement Optimizing

Using all straight strands for girder lengths less than 70 ft. should be investigated for MoDOT Standard Girder Types 6, 7, 8 and all NU Standard Girders in order to reduce risk of strand or hold-down breakage, increase safety by reducing risk of injury during fabrication and reduce cost.

Consider using the same section for all spans. This permits the use of shorter girders in the casting bed with longer girders, even if straight strands are needed, in the top flanges of the girders. They can be placed at either end of the bed and still optimize the usage of the bed.

Consider using the same number of draped strands for all spans and debond where needed. Strand patterns should be similar between long and short spans. For example, the designer should not use a single column of draped strands on the short spans and two columns of draped strands on the long spans. This will prevent optimization of the bed.

Debonding Strands

In all debonding operations the prestressing forces must be in such a manner as to prevent any sudden or shock loading.

Debonding a strand consists of wrapping the unnecessary strand(s) with a polyethylene plastic sleeve that prevents interaction of the strand with the concrete during casting and release which prevents any prestress force transfer.

751.22.2.3 Flexure

Flexure capacity of prestressed I-girders shall be determined as the following.

Flexural resistance at strength limit state

$\,M_{r}=\phi M_{n}\geq M_{u}$

Where:

$\,M_{r}$	=	Flexural resistance
$\,M_{n}$	=	Nominal flexural resistance
$\,M_{u}$	=	Total factored moment from Strength I load combination
$\,\phi$	=	Flexural resistance factor as calculated in LRFD 5.5.4.2

Negative moment reinforcement design

P/S I-girder shall be designed as a reinforced concrete section at regions of negative flexures (i.e., negative moments).

At least one-third of the total tensile reinforcement provided for negative moment at the support shall have an embedment length beyond the point of inflection not less than the specified development length of the bars used.

Slab longitudinal reinforcement that contributes to making the precast beam continuous over an intermediate bent shall be anchored in regions of the slab that can be shown to be crack-free at strength limit states. This reinforcement anchorage shall be staggered. Regular longitudinal slab reinforcement may be utilized as part of the total longitudinal reinforcement required.

Effective Slab Thickness

An effective slab thickness shall be used for design by deducting from the actual slab thickness a 1” integral, sacrificial wearing surface.

Design A1 reinforcement in the top flange

The A1 reinforcement shall resist the tensile force in a cracked section computed on the basis of an uncracked section. For MoDOT girders, A1 reinforcement shall consist of deformed bars. For NU girders, A1 reinforcement shall consist of strands for reinforcement support (4 – 3/8” Strands, Typ.) and WWR (6 – W8, Typ.) with deformed bars added only as needed. Reinforcement shall be designed and spliced using f’_ci in accordance with EPG 751.5.9.2.7 Development and Lap Splices.

Required steel area is equal to:

$\,A1={\frac {T_{t}}{f_{s}}}$

Where:

$\,f_{s}$	= $\,0.5f_{y}\leq 30KSI$, allowable tensile stress of mild steel, (ksi)
$T_{t}$	= Resultant of total tensile force computed on the basis of an uncracked section, (kips)

Limits for reinforcement

The following criteria shall be considered only at composite stage.

Minimum amount of prestressed and non-prestressed tensile reinforcement shall be such that the factored flexural resistance, M_r, is at least equal to the lesser of:

1) M_cr       LRFD Eq. 5.7.3.3.2-1

2) 1.33M_u

Where:

Mcr	=	Cracking moment, (kip-in.)
Mu	=	Total factored moment from Strength I load combination, (kip-in.)

751.22.2.4 Shear

Shear capacity of P/S I-girder should be checked along girder length and girder-slab interface.

Shear resistance at strength limit state

$\,V_{r}=\phi V_{n}\geq V_{u}$

Where:

$\,V_{r}$	=	Shear resistance
$\,V_{n}$	=	Nominal shear resistance
$\,V_{u}$	=	Total factored shear from Strength I load combination
$\,\phi$	=	Shear resistance factor

Nominal shear resistance

The nominal shear resistance, $\,V_{n}$, shall be lesser of:

• $\,V_{c}+V_{s}+V_{p}$, or
• $\,0.25f'_{c}b_{v}d_{v}+V_{p}$

Where:

$\,V_{c}=0.0316\beta b_{v}d_{v}{\sqrt {f'_{c}}}$

$\,V_{s}={\frac {A_{v}f_{y}d_{v}(cot\theta +cot\alpha )sin\alpha }{s}}$

Where:

$\,V_{c}$	=	Nominal concrete shear resistance, (kips)
$\,V_{s}$	=	Nominal shear reinforcement resistance, (kips)
$\,V_{p}$	=	Component of prestressing force in the direction of shear force, (kips)
$\,b_{v}$	=	Thickness of web, (in.)
$\,d_{v}$	=	Effective shear depth taken as the distance measured perpendicular to the neutral axis, between the resultants of tensile and compressive forces due to flexure, (in.)
$\,s$	=	Spacing of shear reinforcement, (in.)
$\,\beta$	=	Factor indicating ability of diagonally cracked concrete to transmit tension
$\,\theta$	=	Angle of inclination of diagonal compressive stress, (degree)
$\,\alpha$	=	90.0, Angle of inclination of shear reinforcement to a longitudinal axis, (degree)
$\,A_{v}$	=	Area of shear reinforcement, (in.2)
$\,f_{y}$	=	Minimum yield strength of tension shear reinforcement, (ksi)

Design sections near supports

Where a reaction force in the direction of the applied shear introduces compression into the end region of girder, the location of the critical section for shear is measured from the internal face of support a distance, d_v. Otherwise, the design section shall be taken at the internal face of the support.

Where:

$\,d_{v}$ = effective shear depth taken as the distance, measured perpendicular to the neutral axis, between the resultants of the tensile and compressive forces due to flexure; it need not be taken to be less than the greater of 0.9d_e and 0.72h.

Girder regions requiring shear reinforcement

Girder shear reinforcement, usually consisting of stirrups, shall be provided where:

$\,V_{u}>0.50\phi (V_{c}+V_{p})$

Where:

$\,V_{u}$	=	Factored shear force from Strength I load combination, (kips)
$\,V_{c}$	=	Nominal concrete shear resistance, (kips)
$\,V_{p}$	=	Component of prestressing force in the direction of shear force, (kips)
$\,\phi$	= =	Shear resistance factor 0.9 for normal weight concrete

Shear Reinforcement Limits

Minimum reinforcement

Area of shear reinforcement shall not be less than:

$\,A_{v}\geq 0.0316{\Bigg (}{\frac {b_{v}s}{f_{y}}}{\Bigg )}{\sqrt {f'_{c}}}$

Where:

$\,A_{v}$	=	Area of shear reinforcement, (in.2)
$\,b_{v}$	=	Thickness of web, (in.)
$\,s$	=	Spacing of shear reinforcement, (in.)
$\,f'_{c}$	=	Final concrete compressive strength, (ksi)

Maximum reinforcement

Maximum spacing of shear reinforcement shall be determined as:
If $\,v_{u}<0.125f'_{c}$, then $\,s_{max}=0.8d_{v}\leq 24.0^{\prime \prime }$

If $\,v_{u}\geq 0.125f'_{c}$, then $\,s_{max}=0.4d_{v}\leq 12.0^{\prime \prime }$

Where:

$\,d_{v}$	=	Effective shear depth taken as the distance measured perpendicular to the neutral axis, between the resultants of tensile and compressive forces due to flexure, (in.)
$\,v_{u}$	=	Shear stress on concrete, (ksi)
$s_{max}$	=	Maximum spacing of shear reinforcement, (in.)

Shear stress on concrete shall be determined as:

$\,v_{u}={\frac {V_{u}-\phi V_{p}}{\phi b_{v}d_{v}}}$

$\,d_{v}={\Bigg (}d_{e}-{\frac {a}{2}}{\Bigg )}\geq largerof{\begin{cases}0.9d_{e}\\0.72h\end{cases}}$

Where:

$\,v_{u}$	=	Shear stress on concrete, (ksi)
$\,V_{u}$	=	Factored shear from Strength I load combination, (kips)
$\,\phi$	= =	Shear resistance factor 0.9 for normal weight concrete
$\,b_{v}$	=	Thickness of web, (in.)
$\,V_{p}$	=	Component of prestressing force in the direction of shear force, (kips)
$\,d_{v}$	=	Effective shear depth taken as the distance measured perpendicular to the neutral axis, between the resultants of tensile and compressive forces due to flexure, (in.)
	=	$\,{\frac {M_{n}}{A_{s}f_{y}+A_{ps}f_{ps}}}$
$\,d_{e}$	=	Distance from extreme compression fiber to the centroid of tensile force in the tensile reinforcement, (in.)
$\,h$	=	Total height of girder including slab thickness, (in.)

Girder-Slab Interface

The horizontal shear between the girder and slab shall be determined as specified in LRFD 5.8.4.3. The nominal horizontal shear resistance of the interface plane shall be taken as specified in LRFD 5.8.4.1. Minimum interface shear reinforcement shall be provided as specified in LRFD 5.8.4.4. The parameters used in determining the nominal horizontal shear resistance shall be taken as specified for a “cast-in-place concrete slab on clean concrete girder surfaces, free of laitance with surface roughened to an amplitude of 0.25 inch.”

The interface shear shall be resisted by extending and anchoring the vertical shear reinforcement into the slab. If the resistance provided by extending the vertical shear reinforcement is inadequate then additional U-bars may be provided as shown for a MoDOT Standard Girder Type 7 in EPG 751.22.3.6 Girder Reinforcement.

For NU Girders the edges of the top of girder flange are intentionally debonded (see figure below) and shall not be included when determining the nominal horizontal shear resistance. See EPG 751.50 Standard Detailing Notes H2c2.10 for specifics about the debonded width for NU Girders. Similarly, for all other prestressed girders, the joint filler width supporting precast panels shall be considered debonded and excluded when determining the interface resistance.

NU Girder Debonding Limits

Pretensioned anchorage zones

The bursting resistance of anchorage zones provided by vertical reinforcement (i.e., B2 bars, WWF, G402 bars) in the ends of prestressed girders at the service limit state shall be taken as:

$\,P_{r}=f_{s}A_{s}\geq 0.04F_{po}$

Where:

$\,f_{s}$	=	Stress in mild steel not exceeding 20 ksi
$\,A_{s}$	=	Total area of vertical reinforcement located within a minimum distance of h/4 from the end of the girder where h is overall depth of precast member as shown below.
$\,F_{po}$	=	Prestressing force at transfer

MoDOT Standard Girder Anchorage Zone and Confinement Reinforcement

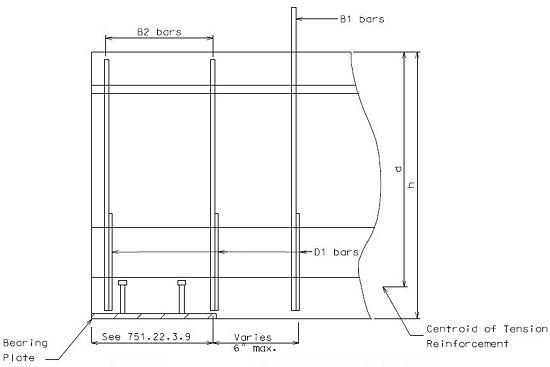
Confinement reinforcement

Reinforcement (i.e., D1 bars or G301 bars, not shown) shown in the figure above shall be placed to confine the prestressing strands in the bottom flange for a minimum distance of 1.5d from the end of beam.

The reinforcement shall not be less than #3 deformed bar, with spacing not exceeding 6.0 inches and shaped to enclose the strands.

MoDOT extends the use of D1 and G301 bars for the full length of girders.

751.22.2.5 Deformations

Criteria for deflection

For investigating maximum absolute deflection, all design lanes shall be loaded, and all supporting components should be assumed to deflect equally.

For composite design, the design cross-section should include the entire width of the roadway and the structurally continuous portions of railings, sidewalks, and median barriers. Note that safety barrier curbs are usually discontinuous over the bents. For skewed bridges, a right cross-section may be used.

Service I load combination shall be used. Dynamic load allowance shall be applied.

See EPG 751.2.4.2 Live Load Deflection Limits.

Calculation of deflection and camber

Deflection and camber calculations shall consider all internal loads (i.e., prestressing, concrete creep, and shrinkage) and external loads such as dead loads and live loads.

Camber is an upward displacement caused by moment due to prestressing forces. Deflection is a downward displacement due to external loads. Therefore, both camber and deflection shall be considered in making an appropriate adjustment for final profile grade on the bridge.

Initial camber at transfer at midspan

Total initial camber at transfer due to self-weight of girder and prestressing forces shall be determined as:

$\,\Delta _{IC}=\Delta _{g}+\Delta _{SS}+\Delta _{HS}$

Where:

$\,\Delta _{IC}$	= Initial camber at transfer
$\,\Delta _{g}$	= Deflection due to self-weight of girder
$\,\Delta _{SS}$	= Camber due to prestressing straight strands
$\,\Delta _{HS}$	= Camber due to prestressing harped strands

Note: Positive and negative values indicate downward and upward displacements, respectively.

Camber at midspan after strand release (Estimated at 7 days)

Theoretical camber of girder after strand release due to self-weight of girder and prestressing forces shall be determined at 7 days as:

$\,\Delta _{7}=\Delta _{IC}+\Delta _{CR\ at\ 7\ days}$

Where:

$\,\Delta _{7}$	= Camber at 7 days after strand release with creep
$\,\Delta _{CR\ at\ 7\ days}$	= Time - dependent camber due to creep at 7 days

Note: Camber is calculated 7 days after strand release to allow sufficient time for inspection. See EPG 1029 Fabricating Prestressed Concrete Members for Bridges.

Camber at midspan after erection (Estimated at 90 days)

Theoretical camber of girder after erection due to self-weight of girder and prestressing forces shall be determined at 90 days as:

$\,\Delta _{90}=\Delta _{IC}+\Delta _{CR\ at\ 90\ days}$

Where:

$\,\Delta _{90}$	= Camber at 90 days after strand release with creep
$\,\Delta _{CR\ at\ 90\ days}$	= Time - dependent camber due to creep at 90 days

Final camber at midspan after slab is poured

Total deformation after slab is poured can be determined as the sum of theoretical camber of girder after erection (90 days) and deflections due to slab and concentrated loads (haunch, diaphragms, etc.) before composite action between slab and girder.

$\,\Delta _{FC}=\Delta _{90}+\Delta _{S}+\sum \Delta _{C}$

Where:

$\,\Delta _{FC}$	=	Final camber after slab is poured
$\,\Delta _{s}$	=	Deflection due to weight of slab
$\,\sum \Delta _{c}$	=	Deflection due to concentrated loads (haunch, diaphragms, etc.)

Final camber along span length

Deformations along the span length can be approximately determined as a product of final camber at midspan times correction factors.

$\,\Delta _{0.10}$	=	0.3140 $\,\Delta _{FC}$ at span fraction of 0.10
$\,\Delta _{0.20}$	=	0.5930 $\,\Delta _{FC}$ at span fraction of 0.20
$\,\Delta _{0.25}$	=	0.7125 $\,\Delta _{FC}$ at span fraction of 0.25
$\,\Delta _{0.30}$	=	0.8130 $\,\Delta _{FC}$ at span fraction of 0.30
$\,\Delta _{0.40}$	=	0.9520 $\,\Delta _{FC}$ at span fraction of 0.40
$\,\Delta _{0.50}$	=	1.0000 $\,\Delta _{FC}$ at span fraction of 0.50

Calculation of camber (upward)

Camber at midspan due to strand forces is determined as the following: For straight strands,

$\,\Delta _{SS}=\Delta _{ss-j}+\Delta _{ss-l}$

Where:   $\,\Delta _{ss-j}={\frac {F_{1-j}e_{1}L^{2}}{8E_{ci}I_{tri}}}$     $\,\Delta _{ss-l}={\frac {F_{1-l}e_{1}L^{2}}{8E_{c}I_{tr}}}$

Where:

$\,F_{1-j}$	= Total prestressing force of straight strands at transfer (including losses due to elastic shortening), (kips)
$\,F_{1-l}$	= Total prestressing force in straight strands due to time-dependent losses prior to slab placement (kips). Opposite in sign to $\,F_{1-j}$, typically.
$\,L$	= Distance between centerlines of bearing pads, (in.)
$\,E_{ci}$	= Initial concrete modulus of elasticity based on $\,f'_{ci}$, (ksi)
$\,E_{c}$	= Final concrete modulus of elasticity based on $\,f'_{c}$ (ksi)
$\,I_{tri}$	= Moment of inertia of transformed non-composite section computed based on $\,E_{ci}$, (in.4)
$\,I_{tr}$	= Moment of inertia of transformed non-composite section based on $\,E_{c}$, (in.4)
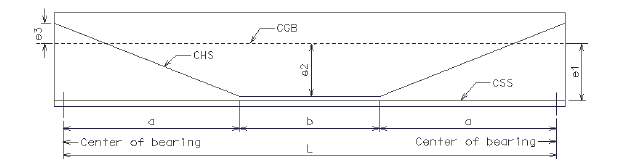
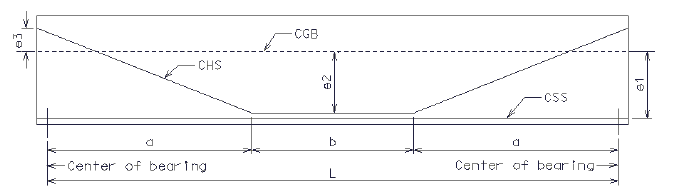
$\,e_{1}$	= Eccentricity between centroid of straight strands (CSS) and center of gravity of transformed non-composite section (CGB) as shown in Figure below, (in.)

For two-point harped strands,

$\,\Delta _{HS}=\Delta _{HS-j}+\Delta _{HS-l}$

Where:   $\,\Delta _{HS-j}={\frac {F_{2-j}e_{2}L^{2}}{8E_{ci}I_{tri}}}-{\frac {F_{2-j}(e_{2}+e_{3})a^{2}}{6E_{ci}I_{tri}}}$     $\,\Delta _{HS-l}={\frac {F_{2-l}e_{2}L^{2}}{8E_{c}I_{tr}}}-{\frac {F_{2-l}(e_{2}+e_{3})a^{2}}{6E_{c}I_{tr}}}$

$a=(L-b)/2$

Where:

$\,F_{2-j}$	= Total prestressing force of harped strands at transfer (including loss due to elastic shortening), (kips)
$\,F_{2-l}$	= Total prestressing force of harped strands due to time-dependent losses prior to slab placement (kips)
$\,b$	= Length between harped points, (in.)
$\,e_{2}$	= Eccentricity between centroid of harped strands (CHS) and center of gravity of transformed non-composite section (CGB) at midspan as shown in Figure below, (in.)
$\,e_{3}$	= Eccentricity between centroid of harped strands (CHS) and center of gravity of transformed non-composite section (CGB) at the end of girder as shown in Figure below, (in.)

Details of girder showing distances and eccentricities used in camber calculations

Calculations of deflections (downward)

Deflections at midspan due to dead loads are determined as the following: For self-weight of girder,

$\,\Delta _{g}={\frac {5W_{g}L^{4}}{384E_{ci}I_{tri}}}$

Where:

$W_{g}$

=

Uniform load due to self-weight of girder, (kip/in.)

For self-weight of slab,

$\,\Delta _{s}={\frac {5W_{s}L^{4}}{384E_{c}I'_{tr}}}$

Where:

$\,W_{s}$

=

Uniform load due to self-weight of slab, (kip/in.)

Weight of additional slab haunch may be treated as uniform or concentrated load as appropriate. Diaphragm weight should be treated as concentrated load.

For one concentrated load at midspan,

$\,\Delta _{c}={\frac {PL^{3}}{48E_{c}I'_{tr}}}$

For two equal concentrated loads,

$\,\Delta _{c}={\frac {Px}{24E_{c}I'_{tr}}}(3L^{2}-4x^{2})$

Where:

$\,P$	= Concentrated load due to diaphragm and/or additional slab haunch, (kips)
$\,x$	= Distance from the centerline of bearing pad to the applied load, P, (in.)

Creep coefficient

Research has indicated that high strength concrete (HSC) undergoes less ultimate creep and shrinkage than conventional concrete.

Creep is a time-dependent phenomenon in which deformation increases under a constant stress. Creep coefficient is a ratio of creep strain over elastic strain, and it can be estimated as follows:

$\,\Psi (t,t_{i})$	= $\,1.9k_{s}k_{hc}k_{f}k_{td}t_{i}^{-0.118}$
$\,k_{s}$	= $\,1.45-0.13(v/s)>=1.0$
$\,k_{hc}$	= $\,1.56-0.008H$
$\,k_{f}$	= $\,5/(1+f'_{ci})$
$\,k_{td}$	= $\,t/(61-4f'_{ci}+t)$

Where:

$\,\Psi$	= Creep coefficient.
$\,H$	= 70, Average annual ambient relative humidity
$\,t$	= Maturity of concrete, (days)     Use 7 days for camber design after strand release     Use 90 days for camber design after erection
$\,t_{i}$	= Age of concrete when a load is initially applied, (days)     Use 0.75 days for camber design.
$\,v/s$	= Volume-to-surface area ratio, (in.)
$\,f'_{ci}$	= Initial girder concrete compressive strength, (ksi)

$\,\Delta _{CR}={\frac {(M_{g}-F_{1-j}e_{1}-F_{2-j}e_{2})\Psi L^{2}}{8E_{ci}I_{tri}}}$

Where:

$\,M_{g}$

= Moment due to girder weight at mid span.

751.22.3 Details

751.22.3.1 Reinforcement Criteria

Minimum Concrete Cover

• 2.0" (Min.) to centerline of strands
• 1.0" for stirrups

Minimum Radius of Bar Bend in Stirrups

1. 3 through #5 bars = 4.0 x Bar Diameter.

Minimum Spacing of Reinforcement Bars For precast concrete, the clear distance between parallel bars in a layer shall not be lesser than:

• Nominal Diameter of bar
• 1.33 x Maximum Aggregate Size
• 1.0"

Minimum Spacing of Prestressing Strands Spacing between each pretressing strand shall not be less than the larger of:

• A clear distance of 1.33 x Maximum Aggregate Size
• Center-to-center spacing of 2" for 0.6" strand diameter
• Center-to-center spacing of 1.75" for 0.5" strand diameter

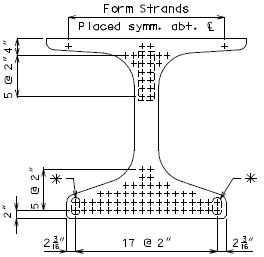
751.22.3.2 MoDOT Standard Girders

751.22.3.2.1 Beam Type 2 Dimensions/Strand Arrangements

GIRDERS 2A THRU 2C $A$ = 310.9 SQ. IN. $Y_{b}$ = 14.08 IN. $I$ = 33,974 IN.4

GIRDER 2A (11 STRANDS)

GIRDER 2B (12 STRANDS)

GIRDERS 2C (14 STRANDS)

NOTE: Investigate the possibility of using all straight strands when strength check of a hold-down device exceeds allowable. Strand arrangements shown for Girders 2A thru 2C have straight strands only. Strand arrangements other than those shown may be investigated by the designer.

$A$ = 310.9 SQ. IN. $Y_{b}$ = 14.08 IN. $I$ = 33,974 IN.4

GROUP I

GROUP II

Numbers shown on girders relate to strand locations.

ATTENTION: Location of harped strands shown in top flange are at end of girder and harped strands in bottom flange are at centerline.

If the web thickness is required to be increased, then the top and bottom flanges are to be increased by the same amount. (1" increments, 2" max.)

751.22.3.2.2 Beam Type 3 Dimensions/Strand Arrangements

GIRDERS 3A THRU 3B $A$ = 381.9 SQ. IN. $Y_{b}$ = 17.08 IN. $I$ = 61,841 IN.4

GIRDER 3A (11 STRANDS)

GIRDER 3B (12 STRANDS)

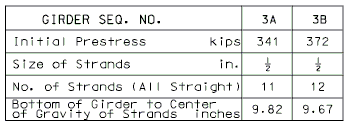
Note: Investigate the possibility of using all straight strands when strength check of a hold-down device exceeds allowable. Strand arrangements shown for Girders 3A thru 3B have straight strands only. Strand arrangements other than those shown may be investigated by the designer.

$A$ = 381.9 SQ. IN. $Y_{b}$ = 17.08 IN. $I$ = 61,841 IN.4

GROUP I

GROUP II

Numbers shown on girders relate to strand locations.

ATTENTION: Location of harped strands shown in top flange are at end of girder and harped strands in bottom flange are at centerline.

If the web thickness is required to be increased, then the top and bottom flanges are to be increased by the same amount. (1" increments, 2" max.)

751.22.3.2.3 Beam Type 4 Dimensions/Strand Arrangements

GIRDERS 4A THRU 4C $A$ = 428.9 SQ. IN. $Y_{b}$ = 19.54 IN. $I$ = 92,450 IN.4

GIRDER 4A (10 STRANDS)

GIRDER 4B (11 STRANDS)

GIRDERS 4C (13 STRANDS)

NOTE: Investigate the possibility of using all straight strands when strength check of a hold-down device exceeds allowable. Strand arrangements shown for Girders 4A thru 4C have straight strands only. Strand arrangements other than those shown may be investigated by the designer.

$A$ = 428.9 SQ. IN. $Y_{b}$ = 19.54 IN. $I$ = 92,450 IN.4

GROUP I

GROUP II

Numbers shown on girders relate to strand locations.

ATTENTION: Location of harped strands shown in top flange are at end of girder and harped strands in bottom flange are at centerline.

If the web thickness is required to be increased, then the top and bottom flanges are to be increased by the same amount. (1" increments, 2" max.)

751.22.3.2.4 Beam Type 6 Dimensions/Strand Arrangements

$\,A$ = 643.6 Sq. In. $\,Y_{b}$ = 25.92 In. $\,I$ = 235,735 In.4

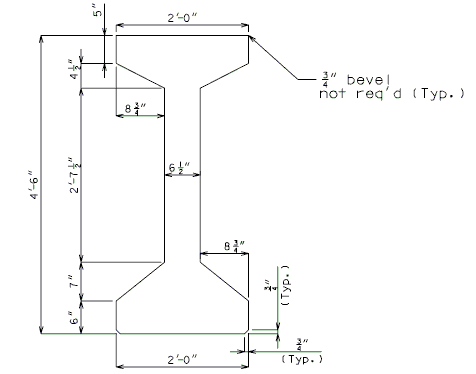
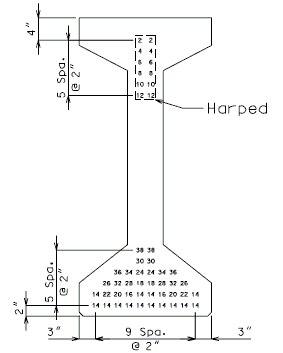GROUP I

Numbers shown on girders relate to strand locations.

ATTENTION: Location of harped strands shown in top flange are at end of girder and harped strands in bottom flange are at centerline.

If the web thickness is required to be increased, then the top and bottom flanges are to be increased by the same amount. (1" increments, 2" max.)

751.22.3.2.5 Beam Type 7 Dimensions/Strand Arrangements

$\,A$ = 787.4 Sq. In. $\,Y_{b}$ = 37.58 In. $\,I$ = 571,047 In.4

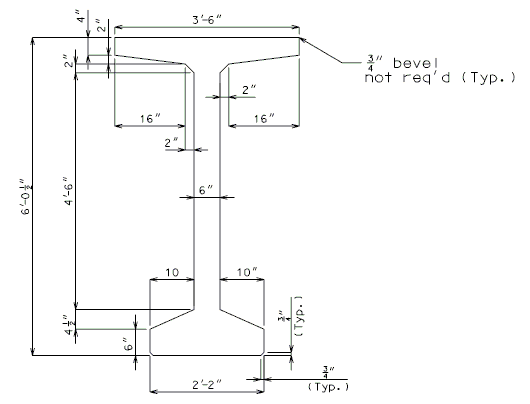
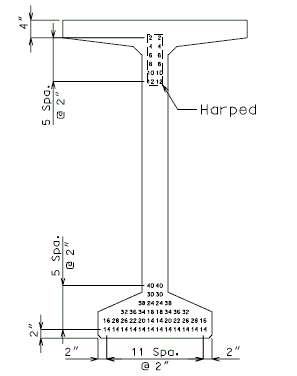GROUP I

Numbers shown on girders relate to strand locations.

ATTENTION: Location of harped strands shown in top flange are at end of girder and harped strands in bottom flange are at centerline.

751.22.3.3 NU Standard Girders

NU Girder Dimensions/Strand Arrangements

* Strands shall be placed on outer edge to help place confinement steel

Note: Strand arrangements shall start at the bottom row and then move up for the most efficient design.

751.22.3.4 Beam Section Properties Tables - Conventional Concrete Strength

The properties of prestressed I-girders in the following tables are valid for $\,f'_{ci}$ = 4.5 ksi and $\,f'_{c}$ = 6 ksi. The modular ratio , $\,n$, is 8 for the initial moment of inertia, $\,I_{initial}$, and 7 for the final moment of inertia, $\,I_{final}$.

Note: Moments of inertia, $\,I_{initial}$ and $\,I_{final}$ are computed based on transformed non-composite section and are used in camber calculations.

Definitions used in tables are:

Section Area	=	Gross area of girder, (in.2)
Section $\,Y_{b}$	=	Distance from bottom of girder to center of gravity of non-transformed non-composite section, (in.)
$\,I_{nontransformed}$	=	Moment of inertia of non-transformed non-composite section, (in.4)
Depth	=	Height of girder, (in.)
Strand size	=	Strand diameter, (in.)
e1*	=	Eccentricity between centroid of straight strands (CSS) and center of gravity of non-transformed non-composite section (CGB) as shown in figure below, (in.)
e2*	=	Eccentricity between centroid of harped strands (CHS) and center of gravity of non-transformed non-composite section (CGB) at midspan as shown in figure below, (in.)
e3*	=	Eccentricity between centroid of harped strands (CHS) and center of gravity of non-transformed non-composite section (CGB) at the end of girder as shown in figure below, (in.)

$\,*$ A more accurate value can be used based on transformed non-composite section. The final camber calculation will not be significantly different using values between transformed and non-transformed sections.

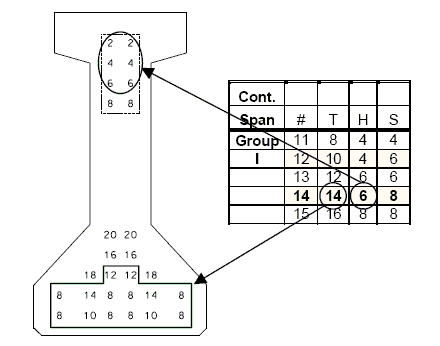
Steps for detailing strand patterns from Prestressed Beam Tables

1. For strand locations at mid-span of girder: Look up the "Total Number of Strands" value for the corresponding strand pattern number. The strands will then be located at that number and all numbers below that number. Ex. For 14 total strands, the strands will be placed at all locations numbered ≤14.
2. For harped strand locations at end of girder: Look up the "Number of Harped Strands" value for the corresponding strand pattern number. The strands will then be located at that number and all numbers below that number. Ex. For 6 harped strands, the strands will be placed at all locations numbered ≤6.

GROUP I

Section Properties
Beam Type 2 -- 6" Web

Section Area =	310.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	14.08	in
$\,I_{nontransformed}$ =	33,974	in4
Depth=	32	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

								Iinitial		Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars, 2-#6
Group	1	8	4	4	11.08	11.08	13.92	36,147	36,627	35,837	36,248
I	2	10	4	6	11.41	11.08	13.92	36,453	36,938	36,100	36,515
	3	12	6	6	11.41	10.08	12.92	36,587	37,075	36,215	36,632
	4	14	6	8	11.08	10.08	12.92	36,794	37,286	36,394	36,814
	5	16	8	8	11.08	9.08	11.92	36,866	37,360	36,456	36,878
	6	18	8	10	10.48	9.08	11.92	36,994	37,491	36,568	36,992
Group	7	8	2	6	11.41	10.08	14.92	36,147	36,627	35,837	36,248
II	8	10	2	8	11.58	10.08	14.92	36,453	36,938	36,100	36,515
	9	12	4	8	11.08	11.08	13.92	36,663	37,151	36,280	36,698
	10	14	4	10	11.28	9.08	13.92	36,794	37,286	36,394	36,814
	11	16	6	10	11.28	8.08	12.92	36,866	37,360	36,456	36,878
	12	18	6	12	10.75	8.08	12.92	36,994	37,491	36,568	36,992
	13	20	6	14	10.65	6.08	12.92	37,024	37,522	36,594	37,019

Section Properties
Beam Type 2 -- 7" Web

Section Area =	342.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	14.26	in
$\,I_{nontransformed}$ =	36,812	in4
Depth=	32	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

								Iinitial		Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	11.26	11.26	13.74	38,994	39,464	38,683	39,085
I	2	10	4	6	11.59	11.26	13.74	39,310	39,784	38,954	39,360
	3	12	6	6	11.59	10.26	12.74	39,450	39,927	39,075	39,482
	4	14	6	8	11.26	10.26	12.74	39,666	40,146	39,261	39,671
	5	16	8	8	11.26	9.26	11.74	39,742	40,225	39,327	39,739
	6	18	8	10	10.66	9.26	11.74	39,877	40,363	39,444	39,858
Group	7	8	2	6	11.59	10.26	14.74	38,994	39,464	38,683	39,085
II	8	10	2	8	11.76	10.26	14.74	39,310	39,784	38,954	39,360
	9	12	4	8	11.26	11.26	13.74	39,528	40,005	39,142	39,550
	10	14	4	10	11.46	9.26	13.74	39,666	40,146	39,261	39,671
	11	16	6	10	11.46	8.26	12.74	39,742	40,225	39,327	39,739
	12	18	6	12	10.93	8.26	12.74	39,877	40,363	39,444	39,858
	13	20	6	14	10.83	6.26	12.74	39,910	39,473	39,473	39,888

Section Properties
Beam Type 2 -- 8" Web

Section Area =	374.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	14.41	in
$\,I_{nontransformed}$ =	39,632	in4
Depth=	32	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

								Iinitial		Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	11.41	11.41	13.59	41,823	42,283	41,510	41,905
I	2	10	4	6	11.74	11.41	13.59	42,147	42,611	41,789	42,186
	3	12	6	6	11.74	10.41	12.59	42,292	42,760	41,914	42,313
	4	14	6	8	11.41	10.41	12.59	42,515	42,985	42,106	42,508
	5	16	8	8	11.41	9.41	11.59	42,596	43,068	42,176	42,579
	6	18	8	10	10.81	9.41	11.59	42,737	43,212	42,298	42,703
Group	7	8	2	6	11.74	10.41	14.59	41,823	42,283	41,510	41,905
II	8	10	2	8	11.91	10.41	14.59	42,147	42,611	41,789	42,186
	9	12	4	8	11.41	11.41	13.59	42,371	42,839	41,982	42,382
	10	14	4	10	11.61	9.41	13.59	42,515	42,985	42,106	42,508
	11	16	6	10	11.61	8.41	12.59	42,596	43,068	42,176	42,579
	12	18	6	12	11.08	8.41	12.59	42,737	43,212	42,298	42,703
	13	20	6	14	10.98	6.41	12.59	42,772	43,249	42,329	42,736

Section Properties
Beam Type 3 -- 6" Web

Section Area =	381.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	17.08	in
$\,I_{nontransformed}$ =	61,841	in4
Depth=	39	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	13.08	14.08	17.92	65,179	65,930	64,702	65,346
I	2	10	4	6	13.75	14.08	17.92	65,659	66,415	65,114	65,762
	3	12	4	8	13.58	14.08	17.92	66,014	66,776	65,421	66,072
	4	14	6	8	13.58	13.08	16.92	66,265	67,032	65,637	66,292
	5	16	6	10	13.48	13.08	16.92	66,614	67,386	65,938	66,597
	6	18	8	10	13.48	12.08	15.92	66,776	67,552	66,079	66,740
	7	20	8	12	13.08	12.08	15.92	67,020	67,799	66,290	66,954
	8	22	8	14	12.51	12.08	15.92	67,178	67,961	66,427	67,095
	9	24	10	14	12.51	11.08	14.92	67,270	68,056	66,508	67,177
Group	10	8	2	6	13.75	13.08	18.92	65,179	65,930	64,702	65,346
II	11	10	2	8	14.08	13.08	18.92	65,659	66,415	65,114	65,762
	12	12	2	10	13.88	13.08	18.92	66,014	66,776	65,421	66,072
	13	14	4	10	13.48	14.08	17.92	66,366	67,134	65,724	66,379
	14	16	4	12	13.75	12.08	17.92	66,614	67,386	65,938	66,597
	15	18	6	12	13.75	11.08	16.92	66,776	67,552	66,079	66,740
	16	20	6	14	13.37	11.08	16.92	67,020	67,799	66,290	66,954
	17	22	6	16	12.83	11.08	16.92	67,178	67,961	66,427	67,095
	18	24	8	16	12.83	10.08	15.92	67,270	68,056	66,508	67,177

Section Properties
Beam Type 3 -- 7" Web

Section Area =	420.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	17.31	in
$\,I_{nontransformed}$ =	66,991	in4
Depth=	39	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	13.31	14.31	17.69	70,343	71,077	69,865	70,493
I	2	10	4	6	13.98	14.31	17.69	70,838	71,577	70,289	70,922
	3	12	4	8	13.81	14.31	17.69	71,207	71,951	70,607	71,243
	4	14	6	8	13.81	13.31	16.69	71,469	72,218	70,833	71,473
	5	16	6	10	13.71	13.31	16.69	71,832	72,585	71,146	71,789
	6	18	8	10	13.71	12.31	15.69	72,004	72,760	71,295	71,940
	7	20	8	12	13.31	12.31	15.69	72,259	73,019	71,516	72,164
	8	22	8	14	12.74	12.31	15.69	72,427	73,190	71,662	72,312
	9	24	10	14	12.74	11.31	14.69	72,526	73,292	71,749	72,401
Group	10	8	2	6	13.98	13.31	18.69	70,343	71,077	69,865	70,493
II	11	10	2	8	14.31	13.31	18.69	70,838	71,577	70,289	70,922
	12	12	2	10	14.11	13.31	18.69	71,207	71,951	70,607	71,243
	13	14	4	10	13.71	14.31	17.69	71,572	72,322	70,922	71,562
	14	16	4	12	13.98	12.31	17.69	71,832	72,585	71,146	71,789
	15	18	6	12	13.98	11.31	16.69	72,004	72,760	71,295	71,940
	16	20	6	14	13.60	11.31	16.69	72,259	73,019	71,516	72,164
	17	22	6	16	13.06	11.31	16.69	72,427	73,190	71,662	72,312
	18	24	8	16	13.06	10.31	15.69	72,526	73,292	71,749	72,401

Section Properties
Beam Type 3 -- 8" Web

Section Area =	459.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	17.49	in
$\,I_{nontransformed}$ =	72,106	in4
Depth=	39	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	13.49	14.49	17.51	75,470	76,191	74,990	75,607
I	2	10	4	6	14.16	14.49	17.51	75,977	76,703	75,425	76,046
	3	12	4	8	13.99	14.49	17.51	76,357	77,087	75,752	76,376
	4	14	6	8	13.99	13.49	16.51	76,628	77,363	75,986	76,613
	5	16	6	10	13.89	13.49	16.51	77,002	77,740	76,308	76,939
	6	18	8	10	13.89	12.49	15.51	77,182	77,923	76,464	77,096
	7	20	8	12	13.49	12.49	15.51	77,446	78,191	76,692	77,328
	8	22	8	14	12.92	12.49	15.51	77,622	78,370	76,845	77,483
	9	24	10	14	12.92	11.49	14.51	77,728	78,479	76,938	77,577
Group	10	8	2	6	14.16	13.49	18.51	75,470	76,191	74,990	75,607
II	11	10	2	8	14.49	13.49	18.51	75,977	76,703	75,425	76,046
	12	12	2	10	14.29	13.49	18.51	76,357	77,087	75,752	76,376
	13	14	4	10	13.89	14.49	17.51	76,733	77,468	76,076	76,704
	14	16	4	12	14.16	12.49	17.51	77,002	77,740	76,308	76,939
	15	18	6	12	14.16	11.49	16.51	77,182	77,923	76,464	77,096
	16	20	6	14	13.78	11.49	16.51	77,446	78,191	76,692	77,328
	17	22	6	16	13.24	11.49	16.51	77,622	78,370	76,845	77,483
	18	24	8	16	13.24	10.49	15.51	77,728	78,479	76,938	77,577

Section Properties
Beam Type 4 -- 6" Web

Section Area =	428.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	19.54	in
$\,I_{nontransformed}$ =	92,450	in4
Depth=	45	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	15.54	16.54	21.46	97,077	98,118	96,416	97,308
I	2	10	4	6	16.21	16.54	21.46	97,727	98,775	96,974	97,872
	3	12	4	8	16.04	16.54	21.46	98,231	99,286	97,408	98,310
	4	14	6	8	16.04	15.54	20.46	98,608	99,669	97,733	98,640
	5	16	6	10	15.94	15.54	20.46	99,103	100,170	98,160	99,071
	6	18	8	10	15.94	14.54	19.46	99,368	100,441	98,390	99,305
	7	20	8	12	15.54	14.54	19.46	99,735	100,813	98,707	99,626
	8	22	8	14	14.97	14.54	19.46	99,995	101,078	98,933	99,856
	9	24	8	16	15.29	12.54	19.46	100,168	101,254	99,083	100,009
	10	26	10	16	15.29	11.54	18.46	100,271	101,360	99,174	100,102
	11	28	10	18	15.32	9.54	18.46	100,323	101,414	99,220	100,149
Group	12	8	2	6	16.21	15.54	22.46	97,077	98,118	96,416	97,308
II	13	10	2	8	16.54	15.54	22.46	97,727	98,775	96,974	97,872
	14	12	4	8	16.04	16.54	21.46	98,231	99,286	97,408	98,310
	15	14	4	10	15.94	16.54	21.46	98,730	99,792	97,838	98,745
	16	16	4	12	16.21	14.54	21.46	99,103	100,170	98,160	99,071
	17	16	6	10	15.94	15.54	20.46	99,103	100,170	98,160	99,071
	18	18	6	12	16.21	13.54	20.46	99,368	100,441	98,390	99,305
	19	20	6	14	15.83	13.54	20.46	99,735	100,813	98,707	99,626
	20	22	6	16	15.29	13.54	20.46	99,995	101,078	98,933	99,856
	21	24	6	18	15.32	11.54	20.46	100,168	101,254	99,083	100,009
	22	26	6	20	15.14	9.54	20.46	100,271	101,360	99,174	100,102
	23	26	8	18	15.32	10.54	19.46	100,271	101,360	99,174	100,102
	24	28	6	22	14.81	7.54	20.46	100,323	101,414	99,220	100,149
	25	28	8	20	15.14	8.54	19.46	100,323	101,414	99,220	100,149
	26	30	8	22	14.81	6.54	19.46	100,341	101,433	99,236	100,166
	27	32	8	24	14.37	4.54	19.46	100,342	101,435	99,238	100,168

Section Properties
Beam Type 4 -- 7" Web

Section Area =	473.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	19.82	in
$\,I_{nontransformed}$ =	100,400	in4
Depth=	45	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	15.82	16.82	21.18	105,048	106,065	104,384	105,256
I	2	10	4	6	16.49	16.82	21.18	105,719	106,743	104,960	105,837
	3	12	4	8	16.32	16.82	21.18	106,242	107,272	105,410	106,291
	4	14	6	8	16.32	15.82	20.18	106,636	107,671	105,750	106,635
	5	16	6	10	16.22	15.82	20.18	107,151	108,192	106,193	107,083
	6	18	8	10	16.22	14.82	19.18	107,431	108,476	106,436	107,328
	7	20	8	12	15.82	14.82	19.18	107,815	108,866	106,768	107,664
	8	22	8	14	15.25	14.82	19.18	108,090	109,145	107,007	107,906
	9	24	8	16	15.57	12.82	19.18	108,275	109,334	107,168	108,070
	10	26	10	16	15.57	11.82	18.18	108,388	109,449	107,266	108,171
	11	28	10	18	15.60	9.82	18.18	108,446	109,510	107,318	108,224
Group	12	8	2	6	16.49	15.82	22.18	105,048	106,065	104,384	105,256
II	13	10	2	8	16.82	15.82	22.18	105,719	106,743	104,960	105,837
	14	12	4	8	16.32	16.82	21.18	106,242	107,272	105,410	106,291
	15	14	4	10	16.22	16.82	21.18	106,760	107,796	105,857	106,742
	16	16	4	12	16.49	14.82	21.18	107,151	108,192	106,193	107,083
	17	16	6	10	16.22	15.82	20.18	107,151	108,192	106,193	107,083
	18	18	6	12	16.49	13.82	20.18	107,431	108,476	106,436	107,328
	19	20	6	14	16.11	13.82	20.18	107,815	108,866	106,768	107,664
	20	22	6	16	15.57	13.82	20.18	108,090	109,145	107,007	107,906
	21	24	6	18	15.60	11.82	20.18	108,275	109,334	107,168	108,070
	22	26	6	20	15.42	9.82	20.18	108,388	109,449	107,266	108,171
	23	26	8	18	15.60	10.82	19.18	108,388	109,449	107,266	108,171
	24	28	6	22	15.09	7.82	20.18	108,446	109,510	107,318	108,224
	25	28	8	20	15.42	8.82	19.18	108,446	109,510	107,318	108,224
	26	30	8	22	15.09	6.82	19.18	108,469	109,533	107,338	108,245
	27	32	8	24	14.65	4.82	19.18	108,472	109,537	107,341	108,248

Section Properties
Beam Type 4 -- 8" Web

Section Area =	518.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	20.06	in
$\,I_{nontransformed}$ =	108,288	in4
Depth=	45	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	16.06	17.06	20.94	112,955	113,952	112,289	113,143
I	2	10	4	6	16.73	17.06	20.94	113,645	114,648	112,881	113,739
	3	12	4	8	16.56	17.06	20.94	114,185	115,193	113,345	114,208
	4	14	6	8	16.56	16.06	19.94	114,594	115,607	113,698	114,563
	5	16	6	10	16.46	16.06	19.94	115,126	116,144	114,156	115,026
	6	18	8	10	16.46	15.06	18.94	115,419	116,442	114,409	115,282
	7	20	8	12	16.06	15.06	18.94	115,818	116,846	114,755	115,631
	8	22	8	14	15.49	15.06	18.94	116,107	117,138	115,004	115,884
	9	24	8	16	15.81	13.06	18.94	116,303	117,337	115,175	116,057
	10	26	10	16	15.81	12.06	17.94	116,424	117,461	115,281	116,165
	11	28	10	18	15.84	10.06	17.94	116,489	117,528	115,338	116,223
Group	12	8	2	6	16.73	16.06	21.94	112,955	113,952	112,289	113,143
II	13	10	2	8	17.06	16.06	21.94	113,645	114,648	112,881	113,739
	14	12	4	8	16.56	17.06	20.94	114,185	115,193	113,345	114,208
	15	14	4	10	16.46	17.06	20.94	114,720	115,734	113,806	114,673
	16	16	4	12	16.73	15.06	20.94	115,126	116,144	114,156	115,026
	17	16	6	10	16.46	16.06	19.94	115,126	116,144	114,156	115,026
	18	18	6	12	16.73	14.06	19.94	115,419	116,442	114,409	115,282
	19	20	6	14	16.35	14.06	19.94	115,818	116,846	114,755	115,631
	20	22	6	16	15.81	14.06	19.94	116,107	117,138	115,004	115,884
	21	24	6	18	15.84	12.06	19.94	116,303	117,337	115,175	116,057
	22	26	6	20	15.66	10.06	19.94	116,424	117,461	115,281	116,165
	23	26	8	18	15.84	11.06	18.94	116,424	117,461	115,281	116,165
	24	28	6	22	15.33	8.06	19.94	116,489	117,528	115,338	116,223
	25	28	8	20	15.66	9.06	18.94	116,489	117,528	115,338	116,223
	26	30	8	22	15.33	7.06	18.94	116,515	117,555	115,361	116,247
	27	32	8	24	14.89	5.06	18.94	116,520	117,560	115,366	116,252

Section Properties
Beam Type 6 -- 6.5" Web

Section Area =	643.6	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	25.92	in
$\,I_{nontransformed}$ =	235,735	in4
Depth=	54	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

								Iinitial	Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#6	A1 Bars 2-#6
Group	1	14	4	10	23.52	22.92	23.08	248,115	246,353
I	2	16	4	12	23.25	22.92	23.08	249,115	247,213
	3	18	6	12	23.25	21.92	22.08	249,933	247,918
	4	20	6	14	23.06	21.92	22.08	250,920	248,769
	5	22	6	16	22.92	21.92	22.08	251,901	249,616
	6	24	8	16	22.92	20.92	21.08	252,545	250,173
	7	26	8	18	22.59	20.92	21.08	253,342	250,862
	8	28	8	20	22.32	20.92	21.08	254,133	251,547
	9	30	10	20	22.32	19.92	20.08	254,626	251,975
	10	32	10	22	22.10	19.92	20.08	255,408	252,653
	11	34	10	24	21.75	19.92	20.08	256,032	253,195
	12	36	10	26	21.46	19.92	20.08	256,651	253,734
	13	38	12	26	21.46	18.92	19.08	257,011	254,048

Section Properties
Beam Type 6 -- 7.5" Web

Section Area =	697.6	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	26.00	in
$\,I_{nontransformed}$ =	248,915	in4
Depth=	54	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

								Iinitial	Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#6	A1 Bars 2-#6
Group	1	14	4	10	23.60	23.00	23.00	262,852	260,864
I	2	16	4	12	23.33	23.00	23.00	263,868	261,737
	3	18	6	12	23.33	22.00	22.00	264,701	262,454
	4	20	6	14	23.14	22.00	22.00	265,707	263,319
	5	22	6	16	23.00	22.00	22.00	266,706	264,180
	6	24	8	16	23.00	21.00	21.00	267,365	264,749
	7	26	8	18	22.67	21.00	21.00	268,178	265,452
	8	28	8	20	22.40	21.00	21.00	268,987	266,150
	9	30	10	20	22.40	20.00	20.00	269,493	266,589
	10	32	10	22	22.18	20.00	20.00	270,294	267,282
	11	34	10	24	21.83	20.00	20.00	270,933	267,836
	12	36	10	26	21.54	20.00	20.00	271,569	268,387
	13	38	12	26	21.54	19.00	19.00	271,941	268,712

Section Properties
Beam Type 6 -- 8.5" Web

Section Area =	751.6	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	26.07	in
$\,I_{nontransformed}$ =	262,087	in4
Depth=	54	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

								Iinitial	Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#6	A1 Bars 2-#6
Group	1	14	4	10	23.67	23.07	22.93	276,043	274,052
I	2	16	4	12	23.40	23.07	22.93	277,068	274,932
	3	18	6	12	23.40	22.07	21.93	277,908	275,656
	4	20	6	14	23.21	22.07	21.93	278,922	276,528
	5	22	6	16	23.07	22.07	21.93	279,930	277,396
	6	24	8	16	23.07	21.07	20.93	280,596	277,971
	7	26	8	18	22.74	21.07	20.93	281,418	278,680
	8	28	8	20	22.47	21.07	20.93	282,236	279,386
	9	30	10	20	22.47	20.07	19.93	282,750	279,831
	10	32	10	22	22.25	20.07	19.93	283,559	280,531
	11	34	10	24	21.90	20.07	19.93	284,207	281,093
	12	36	10	26	21.61	20.07	19.93	284,851	281,651
	13	38	12	26	21.61	19.07	18.93	285,230	281,981

Section Properties
Beam Type 7 -- 6" Web
Bulb-Tee Girder

Section Area =	787.4	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	37.58	in
$\,I_{nontransformed}$ =	571,047	in4
Depth=	72.5	in
Strand Size=	½	in
$\,f'_{ci}$ =	4.5	ksi
$\,f'_{c}$ =	6	ksi

Cont.								Iinitial	Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 4-#6	A1 Bars 4-#6
Group	1	14	4	10	35.58	34.58	29.92	603,636	598,983
I	2	16	4	12	35.25	34.58	29.92	606,025	601,033
	3	18	6	12	35.25	33.58	28.92	608,125	602,838
	4	20	6	14	35.01	33.58	28.92	610,490	604,871
	5	22	6	16	34.83	33.58	28.92	612,843	606,895
	6	24	8	16	34.83	32.58	27.92	614,652	608,453
	7	26	8	18	34.69	32.58	27.92	616,981	610,459
	8	28	8	20	34.58	32.58	27.92	619,299	612,457
	9	30	10	20	34.58	31.58	26.92	620,839	613,788
	10	32	10	22	34.31	31.58	26.92	622,864	615,536
	11	34	10	24	34.08	31.58	26.92	624,878	617,276
	12	36	10	26	33.89	31.58	26.92	626,881	619,009
	13	38	10	28	33.58	31.58	26.92	628,622	620,517
	14	40	12	28	33.58	30.58	25.92	629,902	621,627

751.22.3.5 Beam Section Properties Tables - Higher Concrete Strength

The properties of prestressed I-girders in the following tables are valid for $\,f'_{ci}$ = 5 ksi and $\,f'_{c}$ = 7 ksi. The modular ratio , $\,n$, is 7 for the initial moment of inertia, $\,I_{initial}$, and 6 for the final moment of inertia, $\,I_{final}$.

Note: Moments of inertia, $\,I_{initial}$ and $\,I_{final}$ are computed based on transformed non-composite section and are used in camber calculations. A1 Bar locations are assumed at 3" from the top of girder.

Definitions used in tables are:

Section Area	=	Gross area of girder, (in.2)
Section $\,Y_{b}$	=	Distance from bottom of girder to center of gravity of non-transformed non-composite section, (in.)
$\,I_{nontransformed}$	=	Moment of inertia of non-transformed non-composite section, (in.4)
Depth	=	Height of girder, (in.)
Strand size	=	Strand diameter, (in.)
e1*	=	Eccentricity between centroid of straight strands (CSS) and center of gravity of non-transformed non-composite section (CGB) as shown in figure below, (in.)
e2*	=	Eccentricity between centroid of harped strands (CHS) and center of gravity of non-transformed non-composite section (CGB) at midspan as shown in figure below, (in.)
e3*	=	Eccentricity between centroid of harped strands (CHS) and center of gravity of non-transformed non-composite section (CGB) at the end of girder as shown in figure below, (in.)

$\,*$ A more accurate value can be used based on transformed non-composite section. The final camber calculation will not be significantly different using values between transformed and non-transformed sections.

Steps for detailing strand patterns from Prestressed Beam Tables

1. For strand locations at mid-span of girder: Look up the "Total Number of Strands" value for the corresponding strand pattern number. The strands will then be located at that number and all numbers below that number. Ex. For 14 total strands, the strands will be placed at all locations numbered ≤14.
2. For harped strand locations at end of girder: Look up the "Number of Harped Strands" value for the corresponding strand pattern number. The strands will then be located at that number and all numbers below that number. Ex. For 6 harped strands, the strands will be placed at all locations numbered ≤6.

GROUP I

Section Properties
Beam Type 2 -- 6" Web

Section Area =	310.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	14.08	in
$\,I_{nontransformed}$ =	33,974	in4
Depth=	32	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

								Iinitial		Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars, 2-#6
Group	1	8	4	4	11.08	11.08	13.92	36,407	36,838	36,062	36,429
I	2	10	4	6	11.41	11.08	13.92	36,828	37,265	36,424	36,797
	3	12	6	6	11.41	10.08	12.92	36,983	37,424	36,559	36,935
	4	14	6	8	11.08	10.08	12.92	37,265	37,711	36,804	37,183
	5	16	8	8	11.08	9.08	11.92	37,304	37,753	36,839	37,221
	6	18	8	10	10.48	9.08	11.92	37,465	37,917	36,980	37,364
Group	7	8	2	6	11.41	10.08	14.92	36,407	36,837	36,061	36,429
II	8	10	2	8	11.58	10.08	14.92	36,829	37,265	36,425	36,797
	9	12	4	8	11.08	11.08	13.92	37,112	37,553	36,670	37,046
	10	14	4	10	11.28	9.08	13.92	37,265	37,711	36,804	37,183
	11	16	6	10	11.28	8.08	12.92	37,304	37,753	36,839	37,221
	12	18	6	12	10.75	8.08	12.92	37,466	37,918	36,981	37,365
	13	20	6	14	10.65	6.08	12.92	37,409	37,864	36,934	37,320

Section Properties
Beam Type 2 -- 7" Web

Section Area =	342.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	14.26	in
$\,I_{nontransformed}$ =	36,812	in4
Depth=	32	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

								Iinitial		Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	11.26	11.26	13.74	39,272	39,691	38,922	39,281
I	2	10	4	6	11.59	11.26	13.74	39,706	40,132	39,297	39,660
	3	12	6	6	11.59	10.26	12.74	39,871	40,300	39,440	39,806
	4	14	6	8	11.26	10.26	12.74	40,165	40,599	39,695	40,064
	5	16	8	8	11.26	9.26	11.74	40,211	40,648	39,736	40,108
	6	18	8	10	10.66	9.26	11.74	40,382	40,822	39,885	40,259
Group	7	8	2	6	11.59	10.26	14.74	39,271	39,691	38,921	39,280
II	8	10	2	8	11.76	10.26	14.74	39,707	40,133	39,297	39,660
	9	12	4	8	11.26	11.26	13.74	40,002	40,432	39,553	39,919
	10	14	4	10	11.46	9.26	13.74	40,165	40,599	39,695	40,064
	11	16	6	10	11.46	8.26	12.74	40,211	40,648	39,736	40,108
	12	18	6	12	10.93	8.26	12.74	40,383	40,823	39,886	40,260
	13	20	6	14	10.83	6.26	12.74	40,331	40,773	39,843	40,219

Section Properties
Beam Type 2 -- 8" Web

Section Area =	374.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	14.41	in
$\,I_{nontransformed}$ =	39,632	in4
Depth=	32	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

								Iinitial		Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	11.41	11.41	13.59	42,114	42,525	41,761	42,113
I	2	10	4	6	11.74	11.41	13.59	42,561	42,977	42,146	42,502
	3	12	6	6	11.74	10.41	12.59	42,734	43,154	42,296	42,654
	4	14	6	8	11.41	10.41	12.59	43,039	43,463	42,560	42,921
	5	16	8	8	11.41	9.41	11.59	43,091	43,518	42,607	42,970
	6	18	8	10	10.81	9.41	11.59	43,270	43,700	42,764	43,129
Group	7	8	2	6	11.74	10.41	14.59	42,114	42,525	41,761	42,112
II	8	10	2	8	11.91	10.41	14.59	42,562	42,978	42,147	42,502
	9	12	4	8	11.41	11.41	13.59	42,867	43,288	42,411	42,769
	10	14	4	10	11.61	9.41	13.59	43,039	43,463	42,560	42,921
	11	16	6	10	11.61	8.41	12.59	43,091	43,518	42,607	42,970
	12	18	6	12	11.08	8.41	12.59	43,271	43,701	42,765	43,130
	13	20	6	14	10.98	6.41	12.59	43,224	43,655	42,725	43,092

Section Properties
Beam Type 3 -- 6" Web

Section Area =	381.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	17.08	in
$\,I_{nontransformed}$ =	61,841	in4
Depth=	39	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	13.08	14.08	17.92	65,603	66,291	65,068	65,656
I	2	10	4	6	13.75	14.08	17.92	66,265	66,962	65,638	66,233
	3	12	4	8	13.58	14.08	17.92	66,753	67,457	66,060	66,660
	4	14	6	8	13.58	13.08	16.92	67,077	67,787	66,341	66,945
	5	16	6	10	13.48	13.08	16.92	67,555	68,271	66,755	67,364
	6	18	8	10	13.48	12.08	15.92	67,723	68,444	66,903	67,516
	7	20	8	12	13.08	12.08	15.92	68,042	68,769	67,182	67,799
	8	22	8	14	12.51	12.08	15.92	68,218	68,949	67,336	67,957
	9	24	10	14	12.51	11.08	14.92	68,260	68,994	67,376	67,998
Group	10	8	2	6	13.75	13.08	18.92	65,604	66,292	65,068	65,657
II	11	10	2	8	14.08	13.08	18.92	66,264	66,961	65,637	66,232
	12	12	2	10	13.88	13.08	18.92	66,753	67,457	66,060	66,660
	13	14	4	10	13.48	14.08	17.92	67,236	67,946	66,477	67,082
	14	16	4	12	13.75	12.08	17.92	67,556	68,272	66,756	67,366
	15	18	6	12	13.75	11.08	16.92	67,725	68,445	66,904	67,517
	16	20	6	14	13.37	11.08	16.92	68,045	68,771	67,184	67,800
	17	22	6	16	12.83	11.08	16.92	68,217	68,948	67,336	67,956
	18	24	8	16	12.83	10.08	15.92	68,259	68,993	67,375	67,998

Section Properties
Beam Type 3 -- 7" Web

Section Area =	420.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	17.31	in
$\,I_{nontransformed}$ =	66,991	in4
Depth=	39	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	13.31	14.31	17.69	70,792	71,464	70,251	70,826
I	2	10	4	6	13.98	14.31	17.69	71,477	72,156	70,841	71,420
	3	12	4	8	13.81	14.31	17.69	71,985	72,670	71,279	71,864
	4	14	6	8	13.81	13.31	16.69	72,326	73,016	71,575	72,163
	5	16	6	10	13.71	13.31	16.69	72,823	73,520	72,006	72,599
	6	18	8	10	13.71	12.31	15.69	73,006	73,707	72,166	72,762
	7	20	8	12	13.31	12.31	15.69	73,342	74,049	72,459	73,060
	8	22	8	14	12.74	12.31	15.69	73,532	74,242	72,626	73,229
	9	24	10	14	12.74	11.31	14.69	73,584	74,298	72,675	73,280
Group	10	8	2	6	13.98	13.31	18.69	70,793	71,465	70,252	70,826
II	11	10	2	8	14.31	13.31	18.69	71,476	72,155	70,840	71,420
	12	12	2	10	14.11	13.31	18.69	71,985	72,670	71,279	71,864
	13	14	4	10	13.71	14.31	17.69	72,487	73,178	71,714	72,303
	14	16	4	12	13.98	12.31	17.69	72,825	73,522	72,007	72,600
	15	18	6	12	13.98	11.31	16.69	73,007	73,708	72,167	72,764
	16	20	6	14	13.60	11.31	16.69	73,344	74,051	72,461	73,061
	17	22	6	16	13.06	11.31	16.69	73,531	74,242	72,625	73,229
	18	24	8	16	13.06	10.31	15.69	73,584	74,298	72,674	73,280

Section Properties
Beam Type 3 -- 8" Web

Section Area =	459.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	17.49	in
$\,I_{nontransformed}$ =	72,106	in4
Depth=	39	in
Strand Size=	0.60	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	13.49	14.49	17.51	75,940	76,598	75,394	75,957
I	2	10	4	6	14.16	14.49	17.51	76,642	77,307	75,999	76,567
	3	12	4	8	13.99	14.49	17.51	77,166	77,837	76,451	77,023
	4	14	6	8	13.99	13.49	16.51	77,520	78,196	76,758	77,334
	5	16	6	10	13.89	13.49	16.51	78,034	78,716	77,203	77,783
	6	18	8	10	13.89	12.49	15.51	78,229	78,914	77,373	77,956
	7	20	8	12	13.49	12.49	15.51	78,580	79,270	77,678	78,265
	8	22	8	14	12.92	12.49	15.51	78,781	79,475	77,855	78,445
	9	24	10	14	12.92	11.49	14.51	78,843	79,540	77,911	78,504
Group	10	8	2	6	14.16	13.49	18.51	75,941	76,599	75,395	75,958
II	11	10	2	8	14.49	13.49	18.51	76,641	77,306	75,998	76,566
	12	12	2	10	14.29	13.49	18.51	77,166	77,837	76,451	77,023
	13	14	4	10	13.89	14.49	17.51	77,684	78,361	76,899	77,476
	14	16	4	12	14.16	12.49	17.51	78,036	78,718	77,204	77,785
	15	18	6	12	14.16	11.49	16.51	78,230	78,916	77,374	77,957
	16	20	6	14	13.78	11.49	16.51	78,582	79,273	77,680	78,267
	17	22	6	16	13.24	11.49	16.51	78,780	79,475	77,854	78,444
	18	24	8	16	13.24	10.49	15.51	78,842	79,540	77,911	78,503

Section Properties
Beam Type 4 -- 6" Web

Section Area =	428.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	19.54	in
$\,I_{nontransformed}$ =	92,450	in4
Depth=	45	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	15.54	16.54	21.46	97,723	98,691	96,972	97,800
I	2	10	4	6	16.21	16.54	21.46	98,623	99,602	97,748	98,583
	3	12	4	8	16.04	16.54	21.46	99,318	100,305	98,347	99,189
	4	14	6	8	16.04	15.54	20.46	99,818	100,812	98,780	99,627
	5	16	6	10	15.94	15.54	20.46	100,497	101,501	99,369	100,223
	6	18	8	10	15.94	14.54	19.46	100,808	101,818	99,640	100,499
	7	20	8	12	15.54	14.54	19.46	101,297	102,314	100,066	100,930
	8	22	8	14	14.97	14.54	19.46	101,611	102,634	100,341	101,210
	9	24	8	16	15.29	12.54	19.46	101,761	102,789	100,475	101,347
	10	26	10	16	15.29	11.54	18.46	101,762	102,794	100,480	101,356
	11	28	10	18	15.32	9.54	18.46	101,633	102,667	100,372	101,250
Group	12	8	2	6	16.21	15.54	22.46	97,724	98,692	96,973	97,801
II	13	10	2	8	16.54	15.54	22.46	98,622	99,601	97,747	98,582
	14	12	4	8	16.04	16.54	21.46	99,318	100,305	98,347	99,189
	15	14	4	10	15.94	16.54	21.46	100,005	101,001	98,941	99,790
	16	16	4	12	16.21	14.54	21.46	100,499	101,503	99,370	100,224
	17	16	6	10	15.94	15.54	20.46	100,497	101,501	99,369	100,223
	18	18	6	12	16.21	13.54	20.46	100,810	101,819	99,641	100,500
	19	20	6	14	15.83	13.54	20.46	101,300	102,317	100,068	100,932
	20	22	6	16	15.29	13.54	20.46	101,610	102,633	100,340	101,209
	21	24	6	18	15.32	11.54	20.46	101,762	102,790	100,476	101,349
	22	26	6	20	15.14	9.54	20.46	101,762	102,794	100,480	101,356
	23	26	8	18	15.32	10.54	19.46	101,764	102,796	100,482	101,357
	24	28	6	22	14.81	7.54	20.46	101,629	102,663	100,369	101,246
	25	28	8	20	15.14	8.54	19.46	101,631	102,666	100,371	101,248
	26	30	8	22	14.81	6.54	19.46	101,381	102,417	100,158	101,037
	27	32	8	24	14.37	4.54	19.46	101,033	102,069	99,860	100,739

Section Properties
Beam Type 4 -- 7" Web

Section Area =	473.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	19.82	in
$\,I_{nontransformed}$ =	100,400	in4
Depth=	45	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	15.82	16.82	21.18	105,729	106,673	104,971	105,778
I	2	10	4	6	16.49	16.82	21.18	106,661	107,614	105,773	106,587
	3	12	4	8	16.32	16.82	21.18	107,384	108,345	106,396	107,216
	4	14	6	8	16.32	15.82	20.18	107,908	108,876	106,850	107,675
	5	16	6	10	16.22	15.82	20.18	108,617	109,593	107,464	108,295
	6	18	8	10	16.22	14.82	19.18	108,950	109,931	107,753	108,589
	7	20	8	12	15.82	14.82	19.18	109,464	110,453	108,201	109,041
	8	22	8	14	15.25	14.82	19.18	109,801	110,795	108,495	109,340
	9	24	8	16	15.57	12.82	19.18	109,968	110,967	108,644	109,492
	10	26	10	16	15.57	11.82	18.18	109,984	110,986	108,661	109,512
	11	28	10	18	15.60	9.82	18.18	109,864	110,869	108,562	109,415
Group	12	8	2	6	16.49	15.82	22.18	105,730	106,674	104,971	105,779
II	13	10	2	8	16.82	15.82	22.18	106,660	107,613	105,772	106,586
	14	12	4	8	16.32	16.82	21.18	107,384	108,345	106,396	107,216
	15	14	4	10	16.22	16.82	21.18	108,100	109,069	107,015	107,841
	16	16	4	12	16.49	14.82	21.18	108,619	109,595	107,465	108,296
	17	16	6	10	16.22	15.82	20.18	108,617	109,593	107,464	108,295
	18	18	6	12	16.49	13.82	20.18	108,951	109,933	107,755	108,590
	19	20	6	14	16.11	13.82	20.18	109,467	110,456	108,203	109,044
	20	22	6	16	15.57	13.82	20.18	109,800	110,794	108,484	109,339
	21	24	6	18	15.60	11.82	20.18	109,970	110,969	108,645	109,494
	22	26	6	20	15.42	9.82	20.18	109,984	110,986	108,661	109,512
	23	26	8	18	15.60	10.82	19.18	109,985	110,988	108,663	109,514
	24	28	6	22	15.09	7.82	20.18	109,860	110,865	108,559	109,411
	25	28	8	20	15.42	8.82	19.18	109,862	110,867	108,561	109,413
	26	30	8	22	15.09	6.82	19.18	109,618	110,624	108,353	109,207
	27	32	8	24	14.65	4.82	19.18	109,271	110,278	108,056	108,910

Section Properties
Beam Type 4 -- 8" Web

Section Area =	518.9	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	20.06	in
$\,I_{nontransformed}$ =	108,288	in4
Depth=	45	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

Cont.								Iinitial		Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 2-#5	A1 Bars 2-#6	A1 Bars 2-#5	A1 Bars 2-#6
Group	1	8	4	4	16.06	17.06	20.94	113,668	114,591	112,902	113,692
I	2	10	4	6	16.73	17.06	20.94	114,627	115,559	113,727	114,523
	3	12	4	8	16.56	17.06	20.94	115,375	116,314	114,372	115,174
	4	14	6	8	16.56	16.06	19.94	115,921	116,866	114,844	115,651
	5	16	6	10	16.46	16.06	19.94	116,655	117,608	115,480	116,291
	6	18	8	10	16.46	15.06	18.94	117,007	117,965	115,786	116,601
	7	20	8	12	16.06	15.06	18.94	117,544	118,509	116,252	117,073
	8	22	8	14	15.49	15.06	18.94	117,900	118,870	116,563	117,387
	9	24	8	16	15.81	13.06	18.94	118,084	119,058	119,725	117,553
	10	26	10	16	15.81	12.06	17.94	118,112	119,089	116,754	117,584
	11	28	10	18	15.84	10.06	17.94	118,001	118,981	116,662	117,494
Group	12	8	2	6	16.73	16.06	21.94	113,669	114,592	112,903	113,693
II	13	10	2	8	17.06	16.06	21.94	114,626	115,558	113,726	114,522
	14	12	4	8	16.56	17.06	20.94	115,375	116,314	114,372	115,174
	15	14	4	10	16.46	17.06	20.94	116,116	117,062	115,012	115,819
	16	16	4	12	16.73	15.06	20.94	116,657	117,610	115,481	116,293
	17	16	6	10	16.46	16.06	19.94	116,655	117,608	115,480	116,291
	18	18	6	12	16.73	14.06	19.94	117,009	117,967	115,787	116,603
	19	20	6	14	16.35	14.06	19.94	117,547	118,512	116,255	117,075
	20	22	6	16	15.81	14.06	19.94	117,899	118,869	116,562	117,387
	21	24	6	18	15.84	12.06	19.94	118,085	119,059	116,727	117,554
	22	26	6	20	15.66	10.06	19.94	118,112	119,089	116,754	117,584
	23	26	8	18	15.84	11.06	18.94	118,113	119,091	116,755	117,585
	24	28	6	22	15.33	8.06	19.94	117,997	118,977	116,659	117,490
	25	28	8	20	15.66	9.06	18.94	117,999	118,979	116,661	117,493
	26	30	8	22	15.33	7.06	18.94	117,760	118,742	116,458	117,291
	27	32	8	24	14.89	5.06	18.94	117,415	118,397	116,163	116,996

Section Properties
Beam Type 6 -- 6.5" Web

Section Area =	643.6	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	25.92	in
$\,I_{nontransformed}$ =	235,735	in4
Depth=	54	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

								Iinitial	Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#6	A1 Bars 2-#6
Group	1	14	4	10	23.52	22.92	23.08	251,047	248,880
I	2	16	4	12	23.25	22.92	23.08	252,425	250,070
	3	18	6	12	23.25	21.92	22.08	253,525	251,022
	4	20	6	14	23.06	21.92	22.08	254,886	252,199
	5	22	6	16	22.92	21.92	22.08	256,238	253,370
	6	24	8	16	22.92	20.92	21.08	257,053	254,081
	7	26	8	18	22.59	20.92	21.08	258,130	255,017
	8	28	8	20	22.32	20.92	21.08	259,191	255,940
	9	30	10	20	22.32	19.92	20.08	259,751	256,433
	10	32	10	22	22.10	19.92	20.08	260,803	257,350
	11	34	10	24	21.75	19.92	20.08	261,604	258,052
	12	36	10	26	21.46	19.92	20.08	262,412	258,760
	13	38	12	26	21.46	18.92	19.08	262,739	259,053

Section Properties
Beam Type 6 -- 7.5" Web

Section Area =	697.6	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	26.00	in
$\,I_{nontransformed}$ =	248,915	in4
Depth=	54	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

								Iinitial	Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#6	A1 Bars 2-#6
Group	1	14	4	10	23.60	23.00	23.00	264,293	262,115
I	2	16	4	12	23.33	23.00	23.00	265,686	263,318
	3	18	6	12	23.33	22.00	22.00	266,801	264,281
	4	20	6	14	23.14	22.00	22.00	268,178	265,473
	5	22	6	16	23.00	22.00	22.00	269,548	266,658
	6	24	8	16	23.00	21.00	21.00	270,378	267,381
	7	26	8	18	22.67	21.00	21.00	271,472	268,330
	8	28	8	20	22.40	21.00	21.00	272,551	269,269
	9	30	10	20	22.40	20.00	20.00	273,125	269,772
	10	32	10	22	22.18	20.00	20.00	274,195	270,705
	11	34	10	24	21.83	20.00	20.00	275,014	271,420
	12	36	10	26	21.54	20.00	20.00	275,839	272,143
	13	38	12	26	21.54	19.00	19.00	276,180	272,447

Section Properties
Beam Type 6 -- 8.5" Web

Section Area =	751.6	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	26.07	in
$\,I_{nontransformed}$ =	262,087	in4
Depth=	54	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

								Iinitial	Ifinal
	#	T	H	S	e1	e2	e3	A1 Bars 2-#6	A1 Bars 2-#6
Group	1	14	4	10	23.67	23.07	22.93	277,522	275,336
I	2	16	4	12	23.40	23.07	22.93	278,930	276,549
	3	18	6	12	23.40	22.07	21.93	280,057	277,523
	4	20	6	14	23.21	22.07	21.93	281,449	278,727
	5	22	6	16	23.07	22.07	21.93	282,834	279,925
	6	24	8	16	23.07	21.07	20.93	283,678	280,658
	7	26	8	18	22.74	21.07	20.93	284,786	281,620
	8	28	8	20	22.47	21.07	20.93	285,881	282,571
	9	30	10	20	22.47	20.07	19.93	286,468	283,085
	10	32	10	22	22.25	20.07	19.93	287,554	284,030
	11	34	10	24	21.90	20.07	19.93	288,388	284,758
	12	36	10	26	21.61	20.07	19.93	289,228	285,493
	13	38	12	26	21.61	19.07	18.93	289,581	285,807

Section Properties
Beam Type 7 -- 6" Web
Bulb-Tee Girder

Section Area =	787.4	in2	NOTE: # = strand pattern number   T = total number of strands   H = number of harped strands   S = number of straight strands
Section $\,Y_{b}$ =	37.58	in
$\,I_{nontransformed}$ =	571,047	in4
Depth=	72.5	in
Strand Size=	0.6	in
$\,f'_{ci}$ =	5	ksi
$\,f'_{c}$ =	7	ksi

Cont.								Iinitial	Ifinal
Span	#	T	H	S	e1	e2	e3	A1 Bars 4-#6	A1 Bars 4-#6
Group	1	14	4	10	35.58	34.58	29.92	609,994	604,448
I	2	16	4	12	35.25	34.58	29.92	613,316	607,307
	3	18	6	12	35.25	33.58	28.92	616,196	609,790
	4	20	6	14	35.01	33.58	28.92	619,469	612,612
	5	22	6	16	34.83	33.58	28.92	622,719	615,417
	6	24	8	16	34.83	32.58	27.92	625,140	617,512
	7	26	8	18	34.69	32.58	27.92	628,347	620,286
	8	28	8	20	34.58	32.58	27.92	631,536	623,046
	9	30	10	20	34.58	31.58	26.92	633,518	624,769
	10	32	10	22	34.31	31.58	26.92	636,280	627,166
	11	34	10	24	34.08	31.58	26.92	639,012	629,539
	12	36	10	26	33.89	31.58	26.92	641,737	631,909
	13	38	10	28	33.58	31.58	26.92	644,052	633,926
	14	40	12	28	33.58	30.58	25.92	645,607	635,289

751.22.3.6 Girder Reinforcement

Bar Reinforcing Steel Details for MoDOT Standard Girders

TABLE OF DIMENSIONS BY GIRDER TYPE

TYPE 2

TYPE 3

TYPE 4

TYPE 6

TYPE 7

WEB

6"

7"

8"

6"

7"

8"

6"

7"

8"

6½"

7½"

8½"

6"

"A"

5½"

5½"

5½"

5½"

5½"

5½"

5½"

5½"

5½"

8¾"

8¾"

8¾"

10"

"B"

4"

4"

4"

4"

4"

4"

4"

4"

4"

4"

4"

4"

4"

"C"

6"

6"

6"

6"

6"

6"

6"

6"

6"

7"

7"

7"

4½"

"D"

3¼"

3¼"

3¼"

5⅛"

5⅛"

5⅛"

6¼"

6¼"

6¼"

4⅛"

4⅛"

4⅛"

4⅛"

"E"

13"

14"

15"

13"

14"

15"

13"

14"

15"

18"

19"

20"

20"

"F"

2"

2"

2"

2"

2"

2"

2"

2"

2"

3"

3"

3"

7¾"

"G"

11"

12"

13"

11"

12"

13"

11"

12"

13"

22"

23"

24"

2'-10"

"H"

2'-6"

2'-6"

2'-6"

3'-1"

3'-1"

3'-1"

3'-7"

3'-7"

3'-7"

4'-4"

4'-4"

4'-4"

5'-10½"

"I"

3'-0½"

3'-0½"

3'-0½"

3'-7½"

3'-7½"

3'-7½"

4'-1½"

4'-1½"

4'-1½"

4'-10½"

4'-10½"

4'-10½"

6'-5"

Note: Dimensions shown above are out to out.

TOTAL BAR LENGTH BY GIRDER TYPE

TYPE 2

TYPE 3

TYPE 4

TYPE 6

TYPE 7

WEB

6"

7"

8"

6"

7"

8"

6"

7"

8"

6½"

7½"

8½"

6"

#4-B1

4'-1"

4'-1"

4'-1"

4'-8"

4'-8"

4'-8"

5'-2"

5'-2"

5'-2"

5'-11"

5'-11"

5'-11"

7'-8"

#5-B1

4'-1"

4'-1"

4'-1"

4'-8"

4'-8"

4'-8"

5'-2"

5'-2"

5'-2"

5'-11"

5'-11"

5'-11"

7'-7"

#6-B1

3'-11"

3'-11"

3'-11"

4'-6"

4'-6"

4'-6"

5'-0"

5'-0"

5'-0"

5'-9"

5'-9"

5'-9"

7'-6"

#6-B2

3'-5"

3'-5"

3'-5"

4'-0"

4'-0"

4'-0"

4'-6"

4'-6"

4'-6"

5'-3"

5'-3"

5'-3"

6'-11"

#4-C1

13"

14"

15"

13"

14"

15"

13"

14"

15"

2'-2"

2'-3"

2'-4"

3'-5"

#4-D1

2'-3"

2'-4"

2'-5"

2'-5"

2'-6"

2'-7"

2'-6"

2'-7"

2'-8"

3'-0"

3'-1"

3'-2"

3'-1"

Note: For girders that have stepped flanges, create new B1 and C1 bars and adjust bar lengths for step heights.

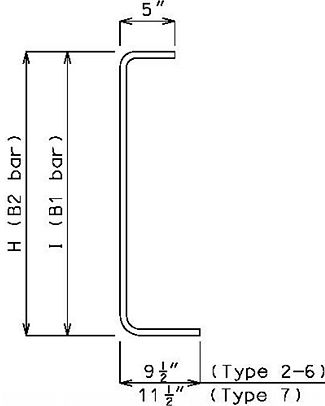
C1 BAR (Girders Type 2-6)		C1 BAR (Girder Type 7)
	B1 and B2 Bar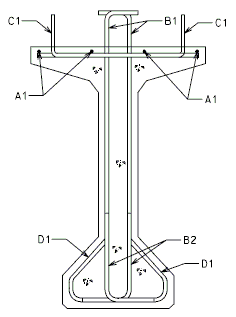
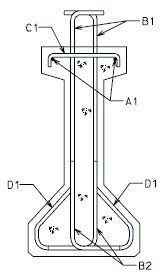
	D1 BAR
SECTION THRU GIRDER (Typical for MoDOT standard girder Type 2-6)		SECTION THRU GIRDER (MoDOT standard girder Type 7)

Welded Wire Reinforcing Steel Details for NU Standard Girders

(See Bridge Standard Drawings for details.)

Alternate Bar Reinforcing Steel Details for NU Standard Girders

Alternate bar reinforcing steel details shall be provided for all NU girders for all spans.

Details shall be similar to details provided for MoDOT Standard Girders:

End section reinforcement shall be designed using #6 B2 Bars @ 3 ½” cts.

C1 bars shall be straight bars without legs.

All B1 bars shall be epoxy coated.

B1 bars shall be #4 or #5 bar maximum. (#4 bars are preferred to allow permissible alternate bar shape.)

Section Reinforcement Details

(Typ. for MoDOT Standard Girder Types 2 thru 6 and NU Standard Girders.)

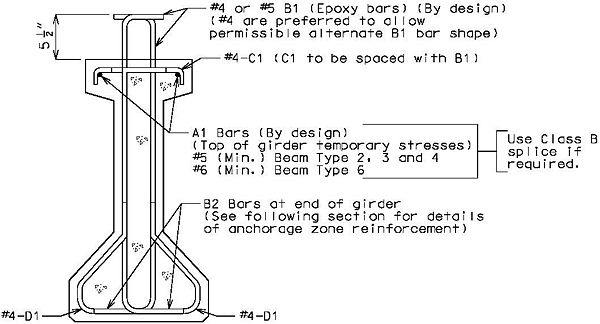MILD STEEL REINFORCEMENT	Guidance: For MoDOT Standard Girder Types 7 or 8, using #6 B1 bars does not provide 1” clearance when center strands are spaced 1” off centerline of girder between hold down devices because of bend radius of #6 bar. Use of #5 bar is preferred. For NU Standard Girders and alternate bar reinforcing steel details, use of #5 bar is preferred similarly.
	Note: B1, B2 and D1 bars shall be placed in pairs. A note on the girder sheet gives the fabricator the option of furnishing the 2-D1 bars as one bar.

B1 BAR PERMISSIBLE ALTERNATE SHAPE

Note: Place the above detail on Prestress I-Girder sheets only where #4-B1 reinforcing bars are used.

Anchorage Zone Reinforcement

The following details meet the criteria for anchorage zone reinforcement for pretensioned girders in EPG 751.22.2.4 for all MoDOT and NU standard girder shapes.

MoDOT Standard Girder End Section Reinforcement

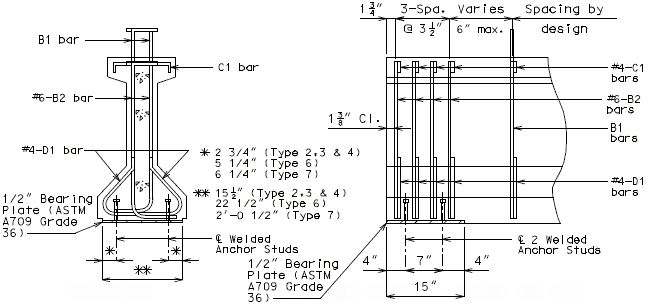
NU Standard Girder End Section Reinforcement

Typical end section reinforcement shall be all welded wire reinforcement (WWR) or all deformed bars. If additional reinforcement is required with WWR, the following options shall be considered.

Option 1 (Preferred)		Option 2
		(Use for heavier reinforcement)

Minimum spacing of reinforcing bars shall be in accordance with LRFD 5.10.3.1.2.

Consideration shall be given to spacing reinforcing bars 1” clear from welded studs on bearing plates (not shown).

Bearing Plate Anchor Studs

The standard ½" bearing plate will be anchored with four ½" x 4" studs for MoDOT shapes and eight ½” x 5” studs for NU shapes.

If required, increase the number of ½" studs and space between open B2 bars.

The minimum ¼" fillet weld between the ½" bearing plate and 1½" sole plate is adequate for all cases.

LFD Seismic Design

Studs shall be designed to meet the criteria of 2002 AASHTO 17^th Edition Division I-A in Seismic Performance Category C or D.

Stud capacity is determined as follows for:

Stud Cap. = (n)(A_s)(0.4F_y)(1.5)

Where:

N = number of studs

A_s = area of stud

F_y = yield strength of stud (50 ksi)

0.4F_y = Allowable Shear in Pins AASHTO Table 10.32.1A

1.5 = seismic overload factor

If required, increase the number of 1/2” studs to six and space between open B2 bars or WWF. If this is still not adequate, 5/8” studs may be used. The following table may be used as a guide for upper limits of dead load reactions:

No. of Studs	Stud Dia.	Max Allowable D.L Reaction (kips)
		A = 0.30	A = 0.36
4	1/2”	78	65
6	1/2”	117	98
4	5/8”	122	102
6	5/8”	184	153
8	1/2”	156	130
10	1/2”	195	163
8	5/8”	244	204
10	5/8”	306	255

751.22.3.7 Bent-up Strands

Bent-up strands for positive moment connection

Tables below show the number of bent-up strands for closed and open diaphragms (with a continuous superstructure), respectively. Provide a minimum number of bent-up strands as shown in tables at the bottom of girder ends. These bent-up strands shall be adequate to resist a positive moment over the bents.

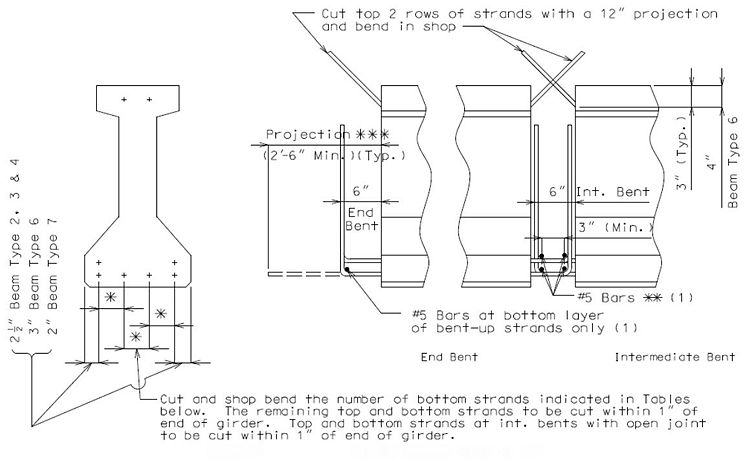
$*$     Varies
$**$  #5 bars typical at each layer of bent-up strands.
$***$  Use 3’-0” projection for NU Girders.
(1)   #5-strand tie bars normal to girder.

WEB THICKNESS (INCHES)	NUMBER OF BOTTOM STRANDS FOR POSITIVE MOMENT CONNECTION (C)
	BEAM TYPE 2	BEAM TYPE 3	BEAM TYPE 4	BEAM TYPE 6	BEAM TYPE 7 (BULB-TEE)
6	6	6	8	---	12
6-1/2	---	---	---	10	---
7(A)	6	8	8	---	---
7-1/2(B)	---	---	---	12	---
8(A)	6	8	10	---	---
8-1/2(B)	---	---	---	12	---

(A) Modified Beam Type 2, 3 or 4.

(B) Modified Beam Type 6.

(C) If available. Otherwise, bend all bottom strands.

NUMBER OF BOTTOM STRANDS FOR POSITIVE MOMENT CONNECTION (C)
NU 35	10
NU 43	10
NU 53	10
NU 63	12
NU 70	12

751.22.3.8 Camber, Haunching and Girder Steps

Camber

Compute theoretical camber of girder at 90 days and show on the plan as a “Theoretical camber of girder after erection (Estimated at 90 days)". Compute theoretical camber of girder at 7 days and show on the plan as a “Theoretical camber of girder after strand release (Estimated at 7 days)". Camber shall be reported to the nearest 1/8 inch.

Sample detail:

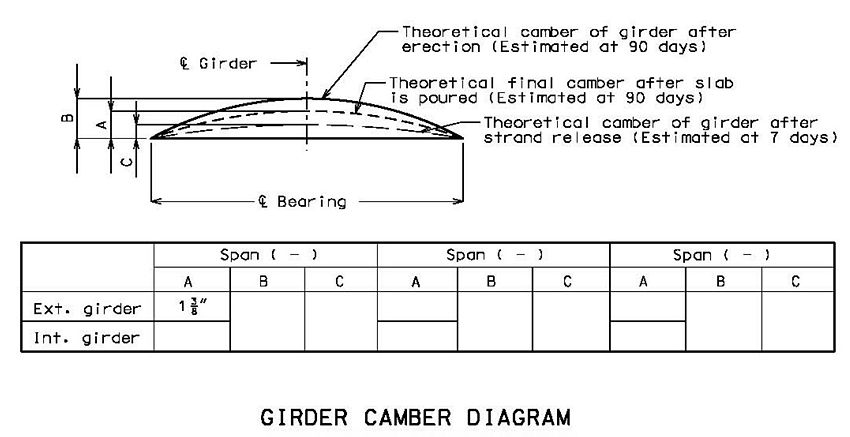
Show conversion factors for girder camber with camber diagram as per EPG 751.50 H2c6.1.

Note: The example shows Dimension A as greater than Dimension C. When Dimension A is less than Dimension C, modify detail to show this correctly keeping definitions of Dimensions A and C the same. MS Cells are given for each case.

Haunching

Haunching for a P/S I-girder bridge is the distance between the top of the girder and the bottom of the slab.

Haunching shall be computed at quarter (1/4) points for bridges with spans less than 75 feet, and at tenth (1/10) points for span 75 feet and longer. Haunching shall be reported to the nearest 1/8 inch. A typical theoretical slab haunching diagram as shown below shall be provided on all P/S I-girder bridges.

For full depth cast-in-place decks, a minimum haunch of 1 in. at the centerline of girder and 1/2 in. at the edge of the girder flange shall be provided to allow for construction tolerances and normal concrete variations. For NU and MoDOT Bulb-Tee standard girders, the minimum haunch may need to be increased. See the Structural Project Manager or Structural Liaison Engineer for full depth cast-in-place decks.

For the same reasons the following minimum haunch shall be provided for precast prestressed panel deck slabs:

1 1/8” for MoDOT standard girders Type 2, 3 and 4

1 1/4” for MoDOT standard girder Type 6

1 1/2” for MoDOT standard girders Type 7 and 8 (bulb-tee) and NU standard girders

A minimum of 1 in. shall be made available below the precast prestressed panels to allow for adequate flow of concrete below the panel. This is accomplished by specifying the placement of 1 in. minimum joint filler thickness under all panels.

The following maximum haunch at the centerline of the girder is allowed when prestressed panels are used:

2 1/2" for MoDOT standard girders Type 2, 3 and 4

4 1/2” for MoDOT standard girders Type 6, 7 and 8 and NU standard girders

A maximum haunch of 3 1/2 in. is allowed for all girders when only the cast-in-place option is used.

The maximum joint filler thickness to be used for supporting panels shall be 2 inches for MoDOT standard girders Type 2, 3 and 4 or 4 inches for MoDOT standard girders Type 6, 7 and 8 and NU standard girders; the remaining haunch thickness will be addressed by varying the slab thickness.

Sample detail:

MoDOT Standard Girders Type 6, 7 and 8 and NU Standard Girders 1) #4 Hairpin bars at 12 in. centers are required where haunch exceeds 4 inches.

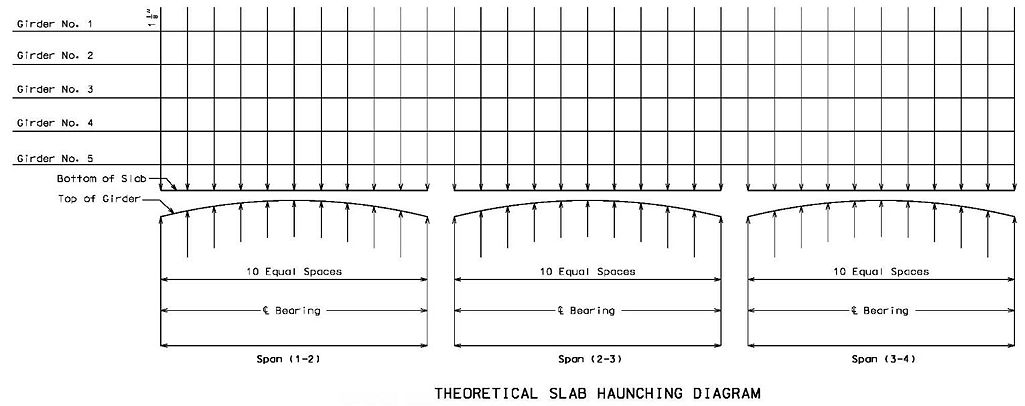
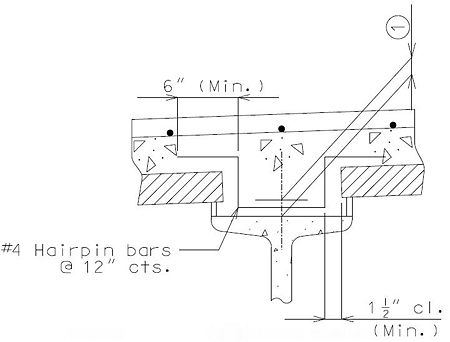
Girder Steps

Steps shall be provided on prestressed girders with precast prestressed panels as shown below to keep the haunch from exceeding 2 in. for MoDOT standard girders Type 2, 3 and 4 or exceeding 4 in. for MoDOT standard girders Type 6, 7 and 8 and NU standard girders. The minimum step height shall be 1/2 in. with 1/2 in. increments with no limit of the number of steps. If the steps accumulate to 1 in. or more, adjust the height of the B1 bars or WWR in 1 in. increments. Length of WWR sections for NU girders should be based on shear and confinement requirements before adjusting height to avoid multiple short length sections.

PART ELEVATION OF GIRDER

SECTION A-A

Girder Top Flange Step Example

Top of Girder

Tops of girders, for bridges with a superelevation of more than 2 percent, shall be sloped across the top flange to match the superelevation as shown below. The minimum thickness of the top flange shall be the standard flange thickness and the overall height at the minimum point shall be the standard girder height.

NU and MoDOT Bulb-Tee standard girders with top flanges exceeding a 4 percent cross-slope may experience sweep after form removal because of the unsymmetrical section and a resulting imbalanced prestressed load. It is recommended that the flange thickness be increased to only half of that required (but less than or equal to 4 percent cross-slope) and the height difference mitigated using thicker joint filler on the high side. If thicker joint filler cannot be fully used to compensate for the height difference, the extra load of a thicker slab must be accounted for in the design of the girders.

Top Flange Slope with Superelevation

751.22.3.9 Open Intermediate Bent Diaphragms

(Expansion Intermediate Bent with Continuous Slab)

Dimensions:

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION

PART PLAN

PART SECTION A-A

* A sloped diaphragm allows clearance for the future placement of jacks needed to replace bearings. ** For Bulb-Tee Girder, spacings less than 8'-8" dimensions A, B & C may have to be modified. *** Make sure the diaphragm is wide enough to provide enough cover for the Coil Tie Rods. **** Not given on plans.

GIRDER TYPE DIMENSIONS A B C TYPE 2 2'-8" 12" 15" 13" TYPE 3 3'-3" 17" 15" 19" TYPE 4 3'-9" 19" 18" 21" TYPE 6 4'-6" 2'-3" 21" 2'-1" BULB-TEE 6'-0½ * 3'-0" 2'-6½" 2'-9" NU 35 **** 18” 14” NU 43 **** 18” 19” NU 53 **** 20” 22” NU 63 **** 2’-0” 2’-0” NU 70 **** 2’-4” 2’-7”

(Expansion Intermediate Bent with Continuous Slab)

Coil Tie Rod:

PART ELEVATION

* 6" (Min.) shall be used for all I-Girders including Bulb-Tee and NU Girders.

PART SECTION A-A

DETAILS OF COIL TIE RODS IN BULB-TEE GIRDERS

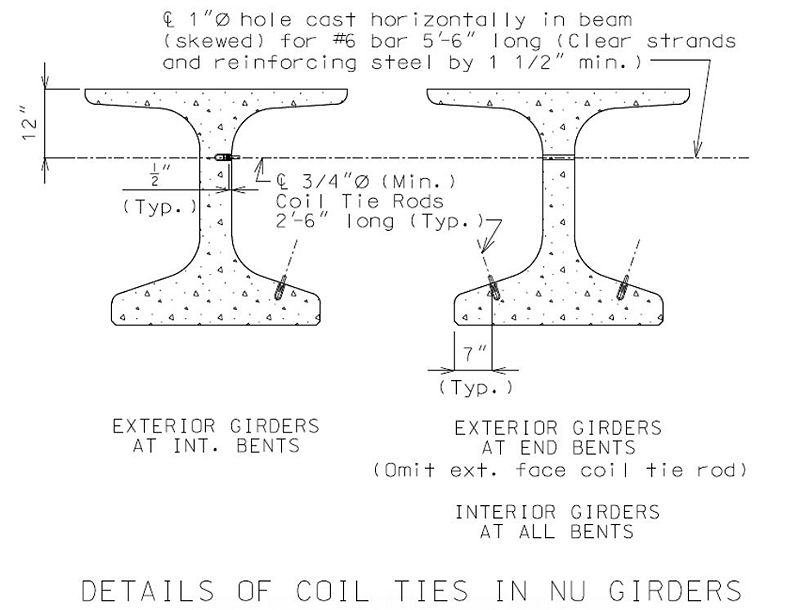
(Expansion Intermediate Bent with Continuous Slab)

Reinforcement:

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION

PART PLAN

(1) For Bulb-Tee Girders, the first #6 Bar shall be placed 10" from the centerline of Web (Top Flange will prevent some Bars from extending into the Slab).
(*) See Section "A" for the placement of reinforcement. (**) Use the same clearance as longitudinal slab steel. (***) #5 Bars for each layer of bent up strands.	PART SECTION A-A

751.22.3.10 Closed Intermediate Bent Diaphragms

(Fixed Intermediate Bents with Continuous Slab)
Dimensions:

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION

PART PLAN

PART PLAN
(Showing Joint Filler)

(*) Make flush with Bent Caps less than 3'-0" wide. For Bent Caps 3'-0" and over, make Diaphragms 2'-6" wide unless skew requires wider Diaphragm to accommodate Coil Ties.   (**) For tapered bearings or for bearings with different thickness use the following note: "Fill area under girders with Joint Filler."

(Expansion Intermediate Bents with Continuous Slab)
Dimensions:

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION

PART ELEVATION FOR NU GIRDERS

PART PLAN

(*) Make flush with Bent Caps less than 3'-0" wide. For Bent Caps 3'-0" and over, make Diaphragm 2'-6" wide unless skew requires wider Diaphragm to accommodate Coil Ties. (**) Use Shear Blocks when Bent Cap steps down in one direction or when there are less than two steps in each direction with maximum step height less than 1 1/2" each. Shear Blocks shall be detailed parallel to the centerline of roadway and shall be designed to resist 0.20 times the tributary weight where tributary weight is defined as the total bent dead load reaction. See this section for shear block design method.

PART LONGITUDINAL ELEVATION

(Fixed and Expansion Intermediate Bents with Continuous Slab)
Reinforcement (Square Structure):

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION

PART ELEVATION FOR NU GIRDERS

(1) For Bulb-Tee Girders, the #6 Bar shall be a min. of 15" from centerline of Girder and will not extend past the bottom of the top flange. (2)For Bulb-Tee Girders, use 3-#4 Bars in each Diaphragm face. (*) #5 Bars for each layer of bent up strands. (**) Use the same clearance as the longitudinal slab steel. (***) By design, Min. #6 Dowel bars @ 12" cts. (Typ.) (Fixed bent only).

PART ELEVATION A-A	SECTION THRU DIAPHRAGM

(Fixed and Expansion Intermediate Bents with Continuous Slab)
Reinforcement (Skewed Structure):

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION

(1) For Bulb-Tee Girders, the #6 Bar shall be a min. of 15" from centerline of Girder and will not extend past the bottom of the top flange. (2)For Bulb-Tee Girders, use 3-#4 Bars in each Diaphragm face. (*) #5 Bars for each layer of bent up strands. (**) Omit leg on outside of exterior girder. (***) By design, Min. #6 Dowel bars @ 12" cts. (Typ.) (Fixed bent only).

SKEWS THRU 25 DEG.	SKEWS OVER 25 DEG.

(Change in Girder Height at Fixed Bents)
General:

Change girder heights within a continuous girder series only when specified on Design Layout or by Structural Project Manager.

Girder heights can only be changed at fixed bents for continuous series.

See EPG 751.11.3.6 Girder/Beam Chairs for additional girder chair details.

(Change in Girder Height at Fixed Bents) Reinforcement:

PART ELEVATION

PART PLAN

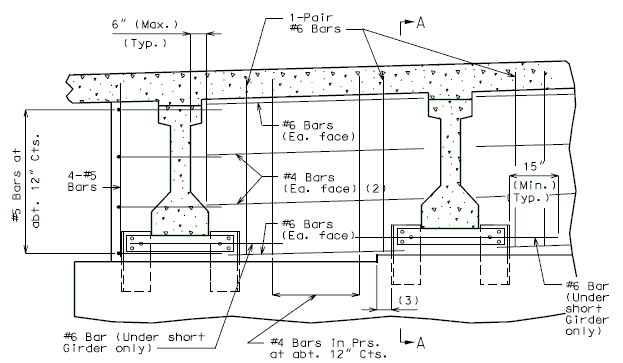
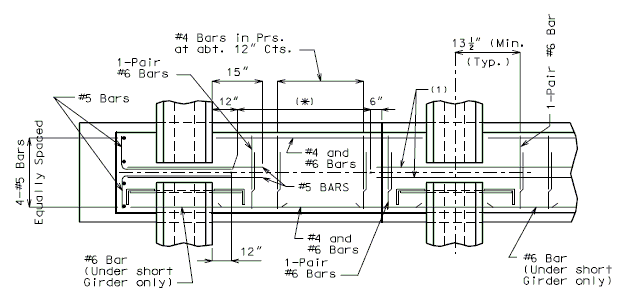
(*) By design, min. #6 dowel bars @ 12" cts. (Typ.) (1) At each layer of bent strands. (2) For bulb-tee girders, use 3-#4 bars in each diaphragm face. (3) 3" min. when using beam step. (4) By design, min. #6 @ 12" cts. dowel bars (Typ.)

PART SECTION A-A THRU DIAPHRAGM

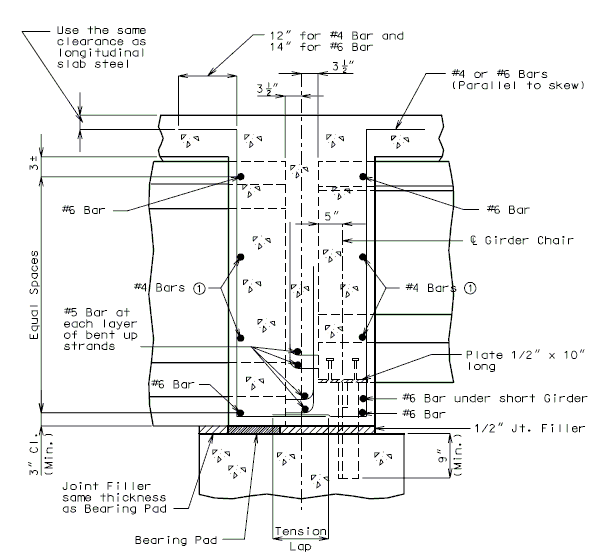
Note: Girder heights can change a maximum of one girder type. (1) For bulb-tee girders, use 3-#4 bars in each diaphragm face.

(Change in Girder Height at Fixed Bents)
Edge Distance Details:

PART PLAN SKEWED STRUCTURES

NOTE:

Field bending may be required for #4 and #6 H Bars in skewed structures near short exterior girder.

PART PLAN SQUARED STRUCTURES

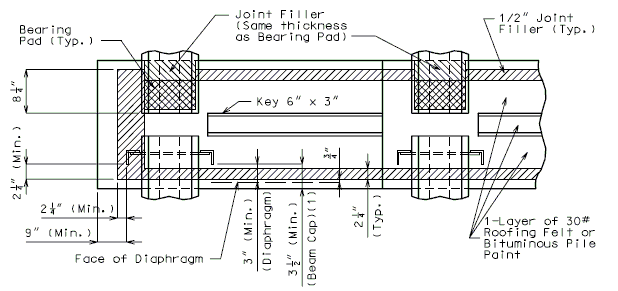
(1) When beam width is controlled by girder chair clearance, make diaphragm flush with beam cap.

3/4" Chamfer and 1/2" Joint Filler

SECTION THRU
INTERMEDIATE DIAPHRAGMS

DETAIL "A"

751.22.3.11 Non-integral End Bent Diaphragms

(End Diaphragm with no Expansion Devices)
Dimensions:

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION NEAR END BENT

PART PLAN NEAR END BENT

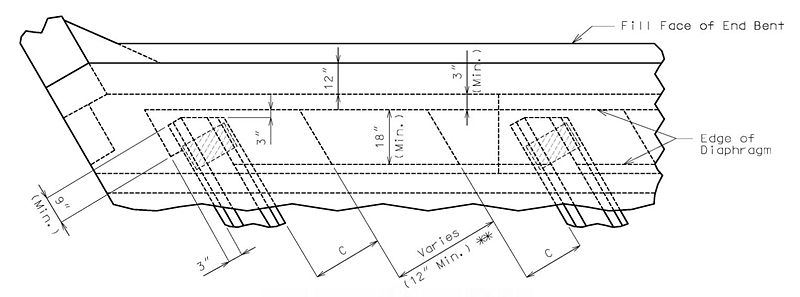
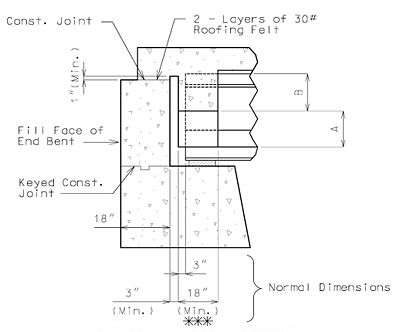PART SECTION A-A

* A sloped diaphragm allows clearance for the future placement of jacks needed to replace bearings.   ** For Bulb-Tee Girder, spacings less than 8'-8" dimensions A, B & C may have to be modified.   *** Make sure the diaphragm is wide enough to provide cover for the coil tie rods.   **** Not given on plans.

GIRDER TYPE DIMENSIONS A B C TYPE 2 2'-8" 12" 15" 13" TYPE 3 3'-3" 17" 15" 19" TYPE 4 3'-9" 19" 18" 21" TYPE 6 4'-6" 2'-3" 21" 2'-1" BULB-TEE 6'-0½ * 3'-0" 2'-6½" 2'-9" NU 35 **** 18” 14” NU 43 **** 18” 19” NU 53 **** 20” 22” NU 63 **** 2’-0” 2’-0” NU 70 **** 2’-4” 2’-7”

(End Diaphragm with no Expansion Devices)
Coil Tie Rods:

PART ELEVATION NEAR END BENT

NOTE:	For location of the Coil Tie Rods in a plan view, see Coil Ties.
	* 6" (Min.) shall be used for all I-Girders including Bulb-Tee and NU Girders.

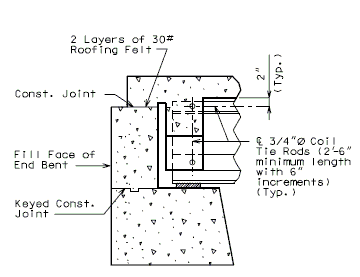
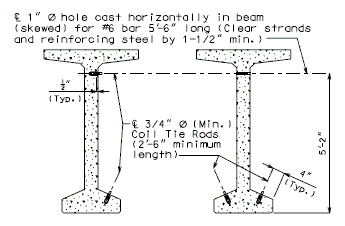
	EXTERIOR GIRDERS	INTERIOR GIRDERS
PART SECTION A-A	DETAILS OF COIL TIE RODS IN BULB-TEE GIRDERS

(End Diaphragm with no Expansion Devices)
Reinforcement:

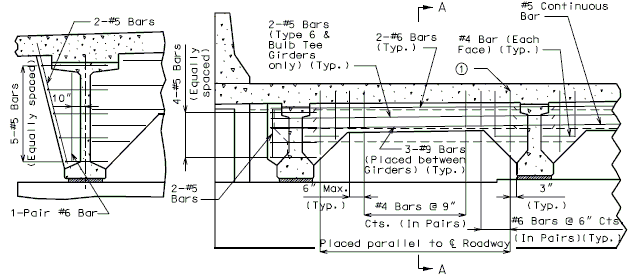
PART ELEVATION NEAR END BENT FOR BULB-TEE GIRDERS

PART ELEVATION NEAR END BENT

PART PLAN NEAR END BENT

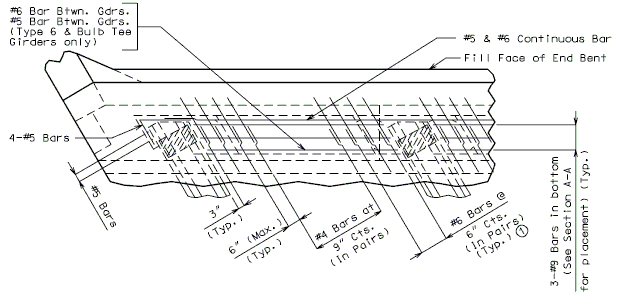
	(1) For Bulb-Tee Girders, the first #6 Bar shall be placed 10" from the centerline of Web (Top Flange will prevent some Bars from extending into the Slab).
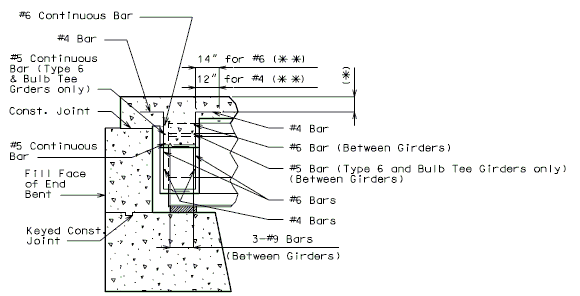
	NOTE: Bars across end of girders to be continuous.
	(*) Use the same clearance as longitudinal slab steel. (**) Show this dimension Bridge Plan Sheets.

PART SECTION A-A

(End Diaphragm with Expansion Devices)
Dimensions:

PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION NEAR END BENT

PART PLAN NEAR END BENT

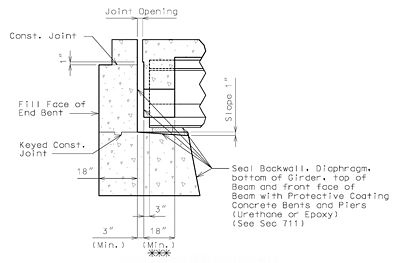PART SECTION A-A	* For Bulb-Tee Girder, spacings less than 8'-8" dimensions A, B & C may have to be modified.	GIRDER TYPE DIMENSIONS A B C TYPE 2 2'-8" 12" 15" 13" TYPE 3 3'-3" 17" 15" 19" TYPE 4 3'-9" 19" 18" 21" TYPE 6 4'-6" 2'-3" 21" 2'-1" BULB-TEE 6'-0½ * 3'-0" 2'-6½" 2'-9" NU 35 **** 18” 14” NU 43 **** 18” 19” NU 53 **** 20” 22” NU 63 **** 2’-0” 2’-0” NU 70 **** 2’-4” 2’-7”
	** A sloped diaphragm allows clearance for the future placement of jacks needed to replace bearings.
	*** Make sure the diaphragm is wide enough to provide cover for the coil tie rods.
*** Not given on plans.

(End Diaphragm with Expansion Devices)
Coil Tie Rods:

PART ELEVATION NEAR END BENT

NOTE:	For location of the Coil Tie Rods in a plan view, see Coil Ties.
	* 6" (Min.) shall be used for all I-Girders including Bulb-Tee and NU Girders.

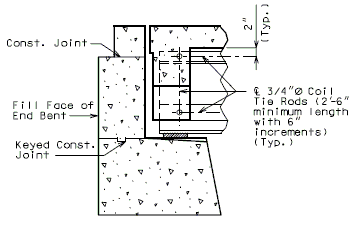
	EXTERIOR GIRDERS	INTERIOR GIRDERS
PART SECTION A-A	DETAILS OF COIL TIE RODS IN BULB-TEE GIRDERS

(End Diaphragm with Expansion Devices)
Reinforcement:

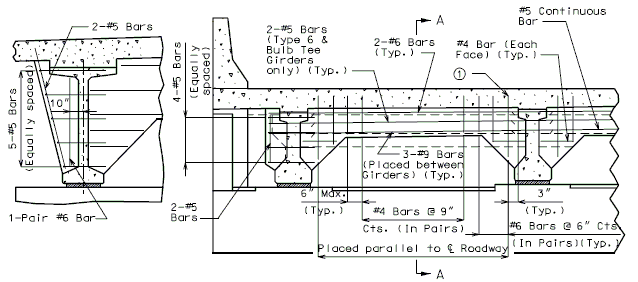
PART ELEVATION NEAR END BENT FOR BULB-TEE GIRDERS

PART ELEVATION NEAR END BENT

PART PLAN NEAR END BENT

	(1) For Bulb-Tee Girders, the first #6 Bar shall be placed 10" from the centerline of Web (Top Flange will prevent some Bars from extending into the Slab).
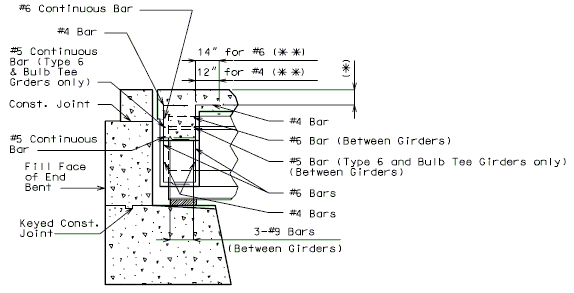
	NOTE: Epoxy Coat all Reinforcing Steel in the End of Diaphragms. NOTE: Bars across end of girders to be continuous.
	(*) Use the same clearance as longitudinal slab steel. (**) Show this dimension Bridge Plan Sheets.

PART SECTION A-A

751.22.3.12 Non-integral Intermediate Bent Diaphragms

(End Diaphragms with Expansion Device)
Dimensions:

NOTE: Slope at top of Beam Cap and Protective
Coating to be used on Structures with Expansion
Devices.

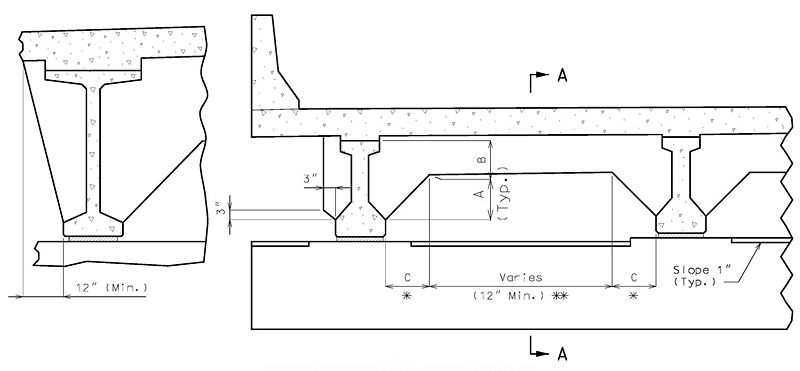
PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION NEAR INT. BENT

PART PLAN NEAR INT. BENT

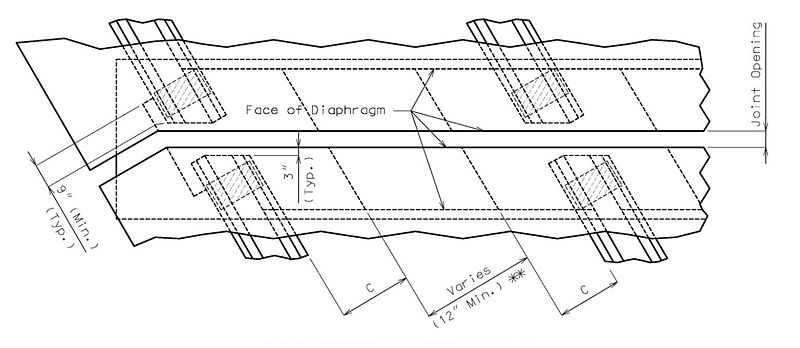
PART SECTION A-A	* A sloped diaphragm allows clearance for the future placement of jacks needed to replace bearings.	GIRDER TYPE DIMENSIONS A B C TYPE 2 2'-8" 12" 15" 13" TYPE 3 3'-3" 17" 15" 19" TYPE 4 3'-9" 19" 18" 21" TYPE 6 4'-6" 2'-3" 21" 2'-1" BULB-TEE 6'-0½ * 3'-0" 2'-6½" 2'-9" NU 35 **** 18” 14” NU 43 **** 18” 19” NU 53 **** 20” 22” NU 63 **** 2’-0” 2’-0” NU 70 **** 2’-4” 2’-7”
	** For Bulb-Tee Girder, spacings less than 8'-8" dimensions A, B & C may have to be modified.
	*** Make sure the diaphragm is wide enough to provide enough cover for the Coil Tie Rods.
**** Not given on plans.

(End Diaphragms with Expansion Device)
Coil Tie Rods:

PART ELEVATION NEAR INT. BENT

NOTE:	For location of the Coil Tie Rods in a plan view, see Coil Ties.
	* 6" (Min.) shall be used for all I-Girders including Bulb-Tee and NU Girders.

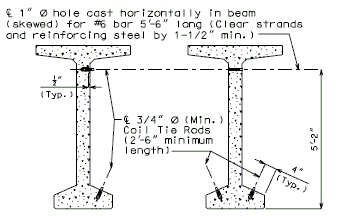
	EXTERIOR GIRDERS	INTERIOR GIRDERS
PART SECTION A-A	DETAILS OF COIL TIE RODS IN BULB-TEE GIRDERS

(End Diaphragms with Expansion Device)
Reinforcement:

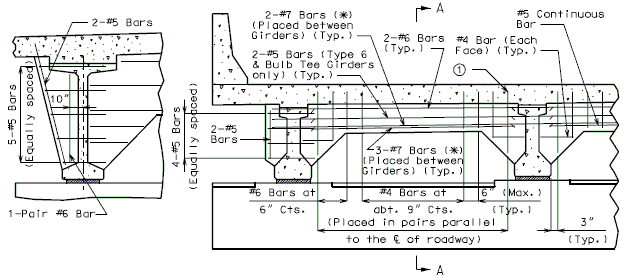
PART ELEVATION NEAR INT. BENT FOR BULB-TEE GIRDERS

PART ELEVATION NEAR INT. BENT

Note: Slope at top of beam cap and protective coating to be used on structures with expansion devices.

(1) For Bulb-Tee Girders, the first #6 Bar shall be placed 10" from the centerline of Web (Top Flange will
prevent some Bars from extending into the Slab).

PART PLAN NEAR INT. BENT

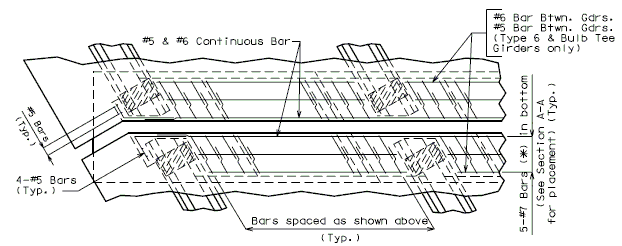
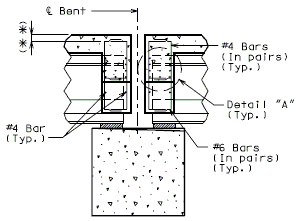
PART SECTION A-A	DETAIL "A"

(*) See Detail "A" for the placement of reinforcement. (**) Use the same clearance as longitudinal slab steel. NOTE: Epoxy coat all reinforcing steel in the end diaphragms.

(End Diaphragm with Finger Plate Expansion Device)
Diaphragm Reinforcements:

CLOSED DIAPHRAGM:

(NOTE: Use only when expansion device connects prestress girder series and steel girder series.)

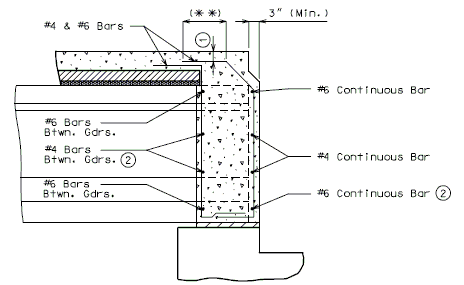
NOTE: See preceding sheets for bar spacing and detail not shown. A protective coating shall be applied to concrete surface exposed to drainage from roadway. Indicate surface to be coated on plans. Epoxy coat all reinforcing steel in the end diaphragms.

(2) For Bulb-Tee Girders use 3-#4 Bars in each face.

OPEN DIAPHRAGM

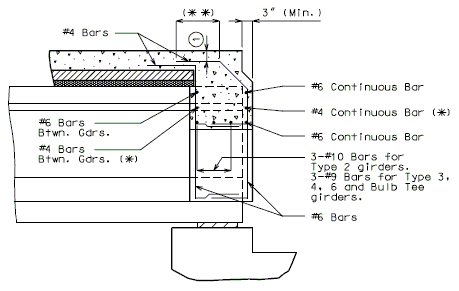
(*)	Use only on Type 6 Girder
(**)	12" for #4 Bars 14" for #6 Bars (Shown on Plans)

(1) Use the same clearance as longitudinal slab steel.

751.22.3.13 Intermediate Diaphragms

Use steel intermediate diaphragm for prestressed spans over 50 feet except for NU 35 and NU 43 girders.

Bridge Standard Drawings

Steel Intermediate Diaphragms

All Girders

Use straight diaphragm normal to girders for skews thru 20°.

Use stepped diaphragm for skews over 20°.

Bulb-Tee Girders

Bulb-Tee spans of 90 ft. or less require one intermediate diaphragm per span.

Bulb-Tee spans of over 90 ft. require two intermediate diaphragms per span (spaced equally as allowed by clearance to harped strands). Maximum spacing is 50 ft.

NU 35 and NU 43 Girders

Permanent intermediate diaphragms are not required for NU 35 and NU 43 standard girders. Temporary intermediate diaphragms/bracing are required for construction of the bridge deck. See EPG 751.50 Note H2c2.2.

751.22.3.14 Coil Ties

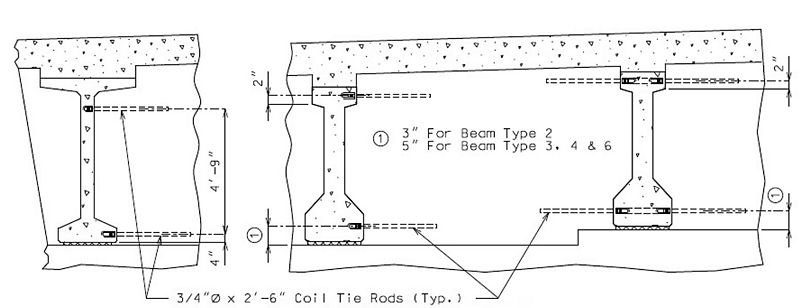
PART ELEVATION FOR BULB-TEE GIRDERS

PART ELEVATION

PART PLAN
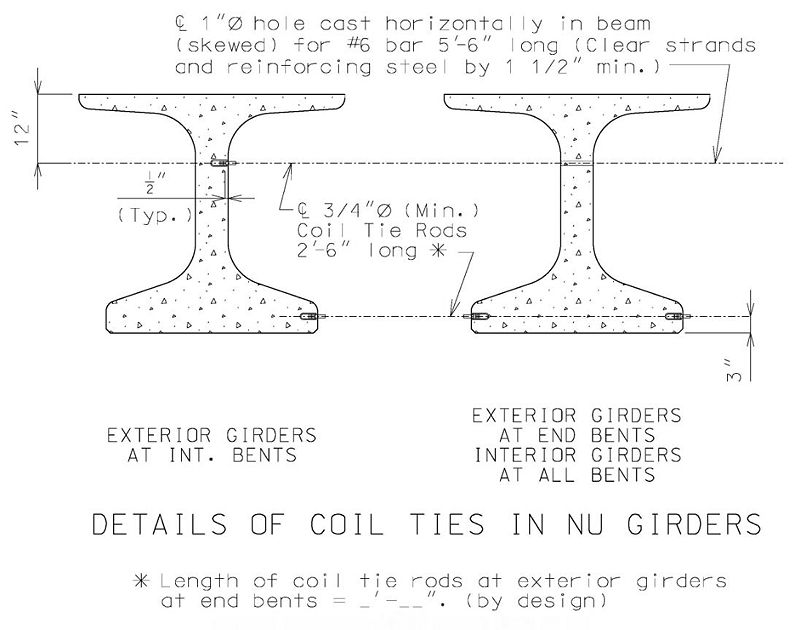
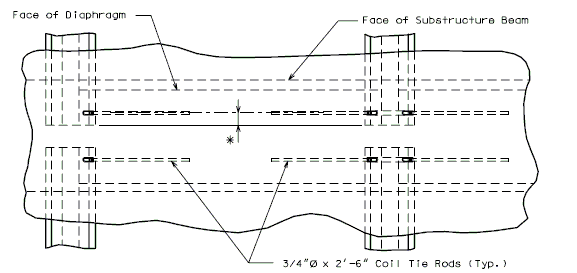
(SQUARE)
* 4" Min. (Typ.) (Do not show Dim. on Plans)

PART PLAN
(SKEWED TO 20 DEG.)

PART PLAN
(SKEWED OVER 20 DEG.)

EXTERIOR GIRDER AT END BENT

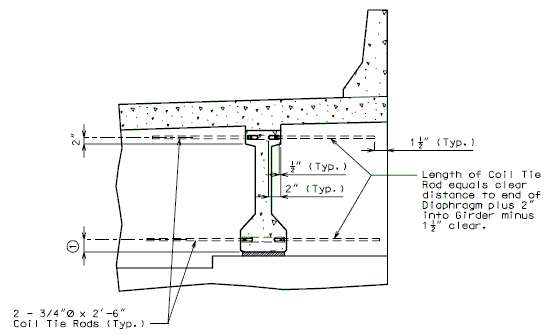
(1)	3" For Beam Type 2 5" For Beam Type 3, 4 & 6
NOTE:	See previous page for location of Coil Tie Rods on Bulb-Tee girders.

751.22.3.15 Dowel Bars (Rods)

PART ELEVATION (FIXED BENT)

SECTION A-A

Dowel bars shall be used for all fixed intermediate bents under prestressed superstructures. Generally, shear resistance from shear key is not considered for typical bridges in seismic performance Category A.

Dowel bars shall be determined by design. (Minimum #6 Bars @ 12" Cts.) For shear stress, f_v, computation, see EPG 751.9.3.1.2 Dowel Rods.

f_v ≤ $\,\phi$_v ● Fv_n

Where,

$\,\phi$_v = Resistance factor

f_v = Shear stress (ksi)

Fv_n = Nominal shear resistance of dowel bar (ksi)

751.22.3.16 Vent Holes

Note: Use vent holes on all stream crossing structures.

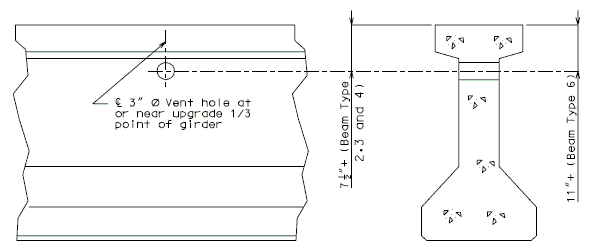
PART ELEVATION OF GIRDER

PART SECTION NEAR VENT HOLE

Note: Place vent holes at or near upgrade of 1/3 point of girders and clear
reinforcing steel or strands by 1-1/2" minimum and steel intermediate
diaphragms bolt connection by 6" minimum.

751.22.3.17 Shear Blocks

A minimum of two Shear Blocks 12" wide x (1) high by width of diaphragm, will be detailed at effective locations on open diaphragm bent caps when adequate structural restraint cannot be provided for with anchor bolts.

ELEVATION VIEW

(1) Height of shear block shall extend a minimum of 1" above the top pf the sole plate.

ELEVATION VIEW

PLAN VIEW

Note: Shear blocks shall be used at bents with open diaphragms when anchor bolts can not be designed to resist earthquake loading.

PLAN VIEW OF BEAM CAP EXPANSION BENTS WITH OPEN DIAPHRAGMS

Note: For Expansion Bents with open diaphragms, the steps or Shear Block (if applicable) should be normal to the length of cap.

PLAN VIEW OF BEAM CAP EXPANSION BENTS WITH CLOSED DIAPHRAGMS

Note: For Closed Diaphragm Expansion Bents, the steps or haunches shall be detailed parallel to the centerline of roadway. For Integral End Bents the steps may be skewed due to stirrups being placed parallel to centerline of roadway. Shear Blocks for Expansion Bents with Closed Diaphragms shall be detailed parallel to the centerline of roadway. Shear Blocks used in conjunction with sole plates and anchor bolts shall be detailed parallel to the edge of sole plate.

751.22.3.18 Miscellaneous

Dimensional Tolerances

I-Girders, Solid Slab Beams, Voided Slab Beams, Box Beams, Double-Tee Girders, Deck Panels and Miscellaneous Prestress Units, see Sec 1029

Expansion Device Support Slots

Used with preformed compression joint seal, flat plate, strip seal or finger plate expansion devices.

PART PLAN OF P/S CONC. I-GIRDER @ EXP. DEVICE END

PART ELEVATION OF P/S CONC. I-GIRDER @ EXP. DEVICE END

(*) Show these dimensions on the P/S concrete girder sheet.

Anchor Bolts
Simple Spans

PART ELEVATION

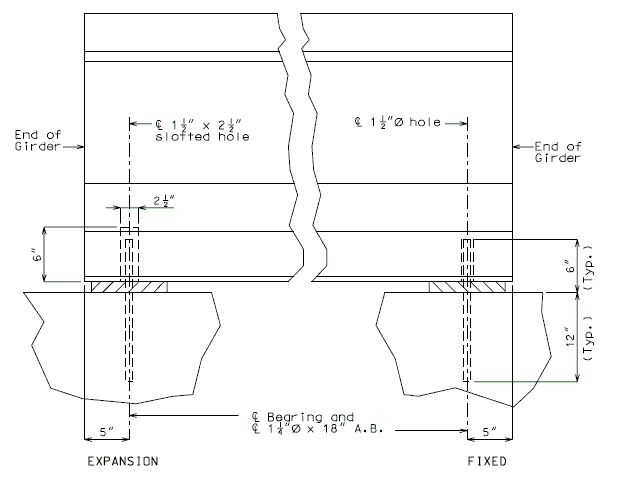
Note: It is permissible for the reinforcing bars and or the strands to come in contact with the materials used in forming A.B. holes. If A.B. holes are formed with galvanized sheet metal, the forms may be left in place. Hole (1-1/2"ø) to be grouted with expansive type mortar meeting the requirements of Sec 1066.